Potential Energy Formula, Definition and Solved Problems

What is Potential Energy and its Formula

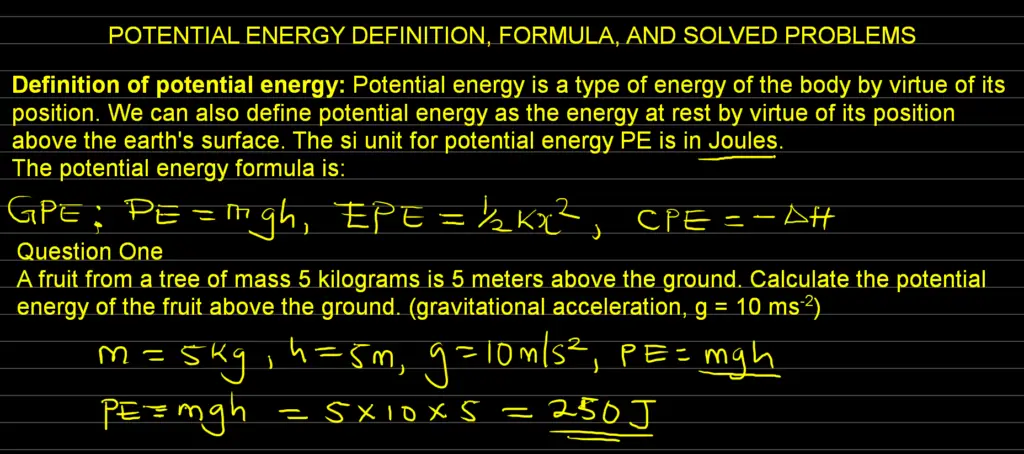
Definition of potential energy: Potential energy is a type of energy of the body by virtue of its position. We can also define potential energy as the energy at rest by virtue of its position above the earth’s surface. The si unit for potential energy PE is in Joules.

The potential energy formula is:

Potential Energy (PE) = mass (m) × gravitational acceleration (g) × height (h)

PE = mgh

Where:

PE is the potential energy in joules (J)

m is the mass of the object in kilograms (kg)

g is the gravitational acceleration (approximately 9.81 m/s² on Earth)

h is the height above a reference point in meters (m)

This formula quantifies the energy an object possesses due to its position relative to the Earth’s surface. It’s fundamental in various physics applications, including understanding work, kinetic energy, and conservation of energy.

Potential energy is a fundamental concept in the world of physics and engineering. Understanding potential energy and its various forms is crucial for solving problems in these fields. In this comprehensive guide, we will explore potential energy in-depth, discussing its types, calculations, real-world applications, and safety considerations. By the end of this article, you will have a solid grasp of potential energy and be equipped to tackle complex problems with confidence.

Video Explanation: Potential Energy Formula and Solved Problems

Here is a video that explains the concept of potential energy and its calculations:

Potential Energy Formula and Solved Problems With Solutions

We can use the following potential energy formula to solve a problem depending on the type of energy we are dealing with:

Gravitational potential energy: PE = mgh

Elastic Potential Energy: PE = [1/2]Kx²

We will now apply the formula to solve a few problems:

Problem 1

A fruit from a tree of mass 5 kilograms is 5 meters above the ground. Calculate the potential energy of the fruit above the ground. (gravitational acceleration, g = 10 ms^-2)

Data

Mass of the fruit, m = 5 kg

Distance above the ground = height, h = 5 m

Gravitational acceleration, g = 10 ms^-2

Unknown value to find

Potential energy, P.E =?

Formula

Potential energy, P.E = mgh

Solution

P.E = mgh = 5 x 5 x 10 = 250 J

Therefore, the potential energy is 250 Joules.

Problem 2

Calculate the potential energy of the Kainji dam if the height is 200 meters and the mass and the mass of the water is 5 kilograms. Take gravitational acceleration as 10 meters per second square.

Data

Height of the dam, h = 200 m

Mass of the water, m = 5 kg

Gravitational acceleration, g = 10 ms^-2

Unknown value to find

Potential energy, P.E =?

The Formula to apply

Potential energy, P.E = mgh

Solution

We can now insert our data into the formula to solve the problem

Potential energy, P.E = mgh = 200 x 5 x 10 = 10,000 J = 10kJ

Therefore, the potential energy of the Kainji dam is 10 kilojoules.

Problem 3

A stone of mass 750 grams is thrown vertically upward with a velocity of 10 meters per second. Find the potential energy at the greatest height if its height above the ground is 5 meters

Data

Mass of the stone, m = 750 g = 0.75 kg

The velocity of the stone, v = 10 m/s

Height above the ground, h = 5 meters

Gravitational acceleration, g = 10 ms^-2

The formula to solve the problem is

Potential energy, P.E = mgh

Solution

P.E = mgh = 0.75 x 10 x 5 = 37.5 J

Therefore, the potential energy P.E is 37.5 Joules

Problem 4

A bag of mass 20 kilograms is lifted to a height of 3 meters. Find the potential energy of the bag.

Data

Height of the bag, h = 3 m

Mass of the bag, m = 20 kg

Gravitational acceleration, g = 10 ms^-2

Unknown value to find

Potential energy, P.E =?

The Formula to apply

Potential energy, P.E = mgh

Solution

We can now insert our data into the formula to solve the problem

Potential energy, P.E = mgh = 3 x 20 x 10 = = 600 J

Therefore, the potential energy of the bag is 600 Joules.

Problem 5

A ball with a mass of 0.5 kilograms is thrown vertically up a clip with a height of 50 meters. Determine the potential energy of the ball at the top of its trajectory.

Data

Height of the bag, h = 50 m

Mass of the bag, m = 0.5 kg

Gravitational acceleration, g = 10 ms^-2

Unknown value to find

Potential energy, P.E =?

The Formula to apply

Potential energy, P.E = mgh

Solution

We can now insert our data into the formula to solve the problem

Potential energy, P.E = mgh = 50 x 0.5 x 10 = = 250 J

Therefore, the potential energy of the bag is 250 Joules.

Problem 6

Though chemical potential energy calculations are more complex, let’s explore a simplified example:

Problem: In a chemical reaction, 1000 Joules of energy is released. Calculate the change in chemical potential energy (ΔH) for the reaction.

Solution:

ChemicalPE=−ΔH, and ΔH=−1000Joules

ChemicalPE = − 1000 Jolues

In this case, the chemical reaction resulted in a release of 1000 Joules of energy.

Types of Potential Energy

1. Gravitational Potential Energy

Gravitational potential energy (GPE) is the energy an object possesses due to its position relative to the Earth’s surface. The formula for calculating GPE is straightforward:

GPE = mgh

Where:

GPE represents gravitational potential energy in joules.
m is the mass of the object in kilograms.
g is the acceleration due to gravity (approximately 9.81 m/s² on Earth).

h is the height or vertical distance in meters.

Practically, GPE can be observed when lifting heavy objects or in the motion of a pendulum. For example, lifting a book onto a shelf increases its GPE, which will be converted into kinetic energy when the book falls.

2. Elastic Potential Energy

Elastic potential energy is stored in objects that can be deformed, such as springs or rubber bands. When these objects are stretched or compressed, they store potential energy. The formula for calculating elastic potential energy is:

PE = [1/2]Kx²

Where:

PE is the elastic potential energy in joules.

k is the spring constant, a measure of stiffness.
x is the displacement from the equilibrium position in meters.

Elastic potential energy is at play in various everyday items, from trampolines to shock absorbers in cars.

3. Chemical Potential Energy

Chemical potential energy is associated with the energy stored in the bonds between atoms and molecules. It plays a significant role in chemistry and is vital for understanding chemical reactions. When chemical bonds are broken or formed, the energy changes are described by chemical potential energy. This form of potential energy is essential in balancing chemical equations and predicting the outcomes of reactions.

Calculating Potential Energy

Gravitational Potential Energy Calculation

Calculating gravitational potential energy is relatively straightforward. Let’s walk through a step-by-step guide:

First Step: Determine the mass of the object in kilograms (m).

Second Step: Measure the height or vertical distance from a reference point to the object’s position (h) in meters. Third Step: Use the formula GPE=mgh to find the gravitational potential energy in joules.

For example, if you have a 5 kg object placed 10 meters above the ground, its GPE would be:

GPE=(5kg)(9.81m/s²)(10m) = 490.5Joules

Elastic Potential Energy Calculation

Elastic potential energy calculations are crucial in understanding the behaviour of springs and similar systems. To calculate elastic potential energy:

First Step: Determine the spring constant (k) in N/m, which characterizes the spring’s stiffness.

Second Step: Measure the displacement (x) in meters from the spring’s equilibrium position.

Third Step: Use the formula EPE=[1/2]kx² to calculate the elastic potential energy in joules.

For instance, if you have a spring with a spring constant of 200 N/m and you stretch it by 0.2 meters, its elastic potential energy would be:

EPE=[1/2](200N/m)(0.2m)²=4Joules

Chemical Potential Energy Calculation

Chemical potential energy calculations are a bit more complex and involve the enthalpy change (ΔH) of a chemical reaction. The relationship is described by the equation:

Chemical PE = −ΔH

Where:

Chemical PE is the chemical potential energy change in joules.
ΔH is the enthalpy change of the reaction in joules.

This change in chemical potential energy is typically determined experimentally and is fundamental in understanding the energy changes in chemical reactions. Balancing chemical equations involves considering these energy changes.

Interactions between Kinetic and Potential Energy

The Relationship between KE and PE Kinetic energy (KE) and potential energy (PE) are closely related. The principle of conservation of mechanical energy states that the sum of an object’s kinetic and potential energies remains constant in the absence of external forces like friction or air resistance. Mathematically, this can be expressed as:

KE+PE=Constant

This concept is particularly important in understanding the motion of objects in various scenarios.

Real-World Examples

The interplay between kinetic and potential energy can be observed in numerous real-world situations:

Roller Coaster Physics: When you’re on a roller coaster, your potential energy increases as you climb the first hill. As you descend, potential energy is converted into kinetic energy, providing the thrilling speed and twists of the ride.

Swinging Pendulum Analogy: A swinging pendulum is another classic example. At the highest point of its swing, it has maximum potential energy and minimal kinetic energy. Conversely, at the lowest point, potential energy is minimal, and kinetic energy is at its peak.

Potential Energy in Different Fields

Physics

In physics, potential energy plays a crucial role in understanding motion, especially in the context of Newton’s Laws of Motion. Potential energy concepts are also fundamental in the Work-Energy Theorem, which relates the work done on an object to its change in kinetic energy.

Engineering

Engineers use the principles of potential energy in various applications. In structural design, potential energy considerations help ensure the stability of buildings and bridges. Additionally, potential energy is a key factor in designing energy storage systems, such as batteries and capacitors.

Environmental Science

In the realm of environmental science, potential energy is closely linked to renewable energy sources. Understanding how potential energy can be harnessed from sources like wind, water, and solar power is essential for creating sustainable energy solutions and combating climate change.

Safety Considerations

Potential Energy Hazards

Understanding potential energy is crucial for safety in various fields. For example, in construction, potential energy can become a hazard when heavy objects are lifted or moved at heights. In chemistry labs, chemical potential energy changes can lead to explosions or chemical spills if not handled properly.

Safety Measures in Relevant Fields

Safety measures are implemented to mitigate potential energy-related risks:

Construction:

Engineers design structures with safety margins to handle potential energy changes.
Workers use safety harnesses and follow strict protocols when working at heights.

Chemistry Labs:

Chemists wear appropriate protective gear.
Labs are equipped with safety devices to contain potential energy releases in case of accidents.

Importance of Awareness Being aware of potential energy and its implications is essential for preventing accidents and ensuring the safety of individuals working in environments where potential energy is a factor.

Reads

You may also like to read:

How to Calculate Kinetic Energy

How to Calculate Work Done in Physics

References

Khan Academy – Gravitational Potential Energy
HyperPhysics – Elastic Potential Energy
Chemical Education Digital Library – Chemical Potential Energy

University of Maryland – Work and Energy

Wikipedia