Newton's Laws of Motion

Newton’s Three Laws of Motion

Over the last 300 years, Newton’s laws of motion became one of the most famous laws of physics. The laws were stated by a famous English physicist and mathematician, and his name is Sir Isaac Newton. Below is a video explaining of the three laws of motion.

Newton’s three laws of motion are as follows:

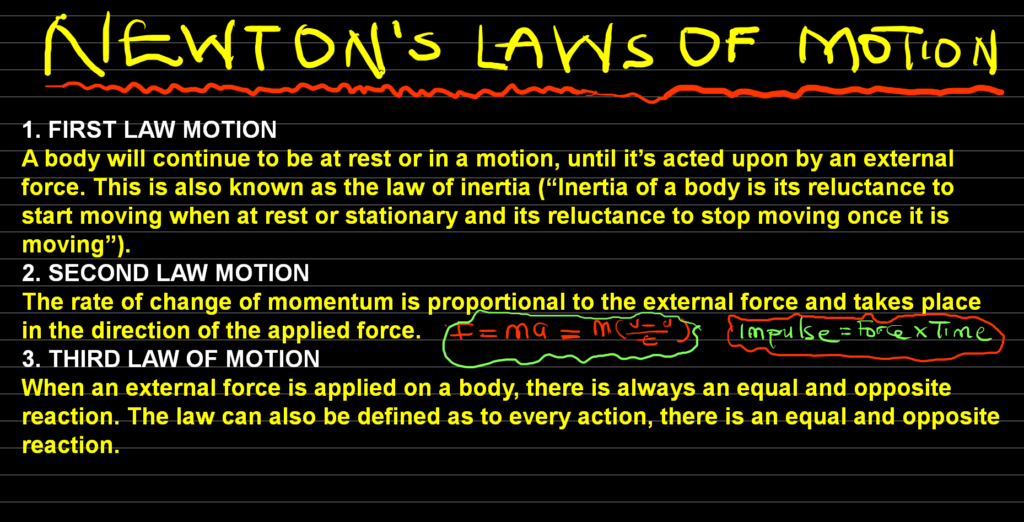
A body will continue to be at rest or in a motion until it’s acted upon by an external force. This is also known as the law of inertia (“Inertia of a body is its reluctance to start moving when at rest or stationary and its reluctance to stop moving once it is moving”).
The rate of change of momentum is proportional to the external force and takes place in the direction of the applied force.
When an external force is applied to a body, there is always an equal and opposite reaction. The law can also be defined as to every action, there is an equal and opposite reaction.

You may also like to read:

Newton’s Laws of Motion Project Ideas

Brief History of the Laws of Motion

Newton was born in England on 4th of January, 1643 in Woolsthorpe Manor House. During his time, children were trained to rear sheeps to earn a living. However, Newton was uninterested in making a living as a farmer. Instead, he was more attracted to books.

At young age, he found love in the books that were arranged in the shelves of his parent’s library. The famous scientist dedicated his time to observing the possible occurrences that happen between sunrise to sunset, spring to summer, autumn to winter. Newton tried to understand what triggers these changes, and it led him to understanding the gravity.

He observed the effect of sun, wind and water on materials like water clocks, windmills, and kites. Isaac finally realizes that the earth and the sky work on the same principle which is contrary to Aristotle’s understanding.

The influential English scientist devoted himself to reading the works of ancient Greeks, Islamic and medieval views on gravity. Newton asked himself as to why an apple fruit falls on the ground instead of going up to the sky. He provided mathematical evidence to Galilei Galileo and Johannes Kepler’s theory of gravity.

Isaac Newton started his journey of research at the age of 23. He came up with a book on classical mechanics, and the name of the book is “Principia Mathematica Philosophiae Naturalis.”. The meaning of the book in English is “Mathematical principles of natural philosophy”.

This book perfectly explained the 3 laws of motion. The three (3) laws of motion shaped our understanding of gravity and we call them Newton’s laws of motion.

Newton’s Laws of Motion in our Everyday Life

We apply Newton’s laws of motion in our everyday life. For example, while playing soccer, we must apply force for the ball to move. Once we apply force on the ball, it will head towards our target due to momentum. The wide range of applications of Newton’s laws is as follows:

Basketball
Rocket science
Javelin throw
Table tennis
Squash sport
Riding Bicycle
Driving a Bus on the road
Eating with a Spoon
An airplane taking off
A bag resting on a desk, and many more.

You may also like to read:

The Newton’s Second Law Practice Problems

Second Law of Motion Examples

Newton’s First (1st) Law of Motion: Law of Inertia

The first law of motion states that A body will continue to be at rest or in motion, until it’s acted upon by an external force. According to this law, when you hide an object in your room. The object will continue to remain in that place for millions of years, until someone or something applies a force to move the object from its original hiding position.

Therefore, when you keep something in a safe place. We can easily say that the object will remain in that place until you decide to change its position or someone moves it from its original position.

Additionally, the same law state that when you set your car in motion. The car will continue to move in a straight line and will never stop. It will wait for you to apply brakes before it can stop. Additionally, the car may also stop if the engine is bad or the fuel is empty. Hence, there must be an application of external force for the car to stop.

Also, if you don’t control the steering, it will continue to move in a straight line until you decide to go left or right. This scenario also explains Newton’s first law of motion or law of inertia.

Understanding Inertia

Newton’s first law of motion is also known as the law of inertia. As defined in his book, the law of inertia is the power by which an object, if it’s at rest, stays at rest, or if in motion, will travel in a straight line.

To understand this law, try rolling a marble on smooth tiles, the marble will continue to move until an object blocks its movement. Alternatively, the friction of the tiles will make the speed of the marble keep decreasing until it comes to rest.

This scenario of a rolling marble against the tiles perfectly describes Newton’s first law of motion. This law explains why your coffee remains peacefully in its cup until you decide to stir it or why your car continues down the highway unless you hit the brakes.

Everyday Examples

Consider a few everyday examples:

A hockey puck sliding on an ice rink with no friction would continue forever if not for external forces like air resistance or the rink’s boards.
In the vacuum of space, where there is no air resistance, celestial bodies such as planets and moons continue in their orbits with the same speed and direction due to their inertia.

You may also like to read:

Second Law Practice Problems

Laws of Motion Project Ideas

Newton’s Second (2nd) Law of Motion

The second law of motion states that when you apply an external force on an object the rate of change of momentum is proportional to the applied force and it takes place in the direction of the force.

Here is the best way to explain this law, when you push a shopping cart in the supermarket. The shopping cart will move because of the external force you apply on it, and the mass of the cart.

Therefore, the mass of the , mass of the body and the external force acting upon it plays a crucial role in setting a body into motion.

Here is another example, before buying anything, push the cart for a while. You will notice that it moves faster. Now, add some of the items you bought from the store into the cart. What did you notice? It’s heavier than it was, right? You may need to add extra force to push it this time around.

Ask yourself again, what brought this sudden change? Here is the answer to your question: “The more mass you add to the cart, the heavier it becomes, and the more force you will need to apply to move the cart”.

Hence, there exists a relationship between the mass of the body and the applied external force.

The mathematical expression (formula) for newton’s second law of motion is

Force (F) = Mass (m) x Acceleration (a)

Which can be written as

F = ma

The S.I unit of force is called Newton (Honors Isaac Newton) or kilogram meter per second square. The symbol of Newton is N, while the symbol for kilogram meters per second square is kgm/s²

The unit of mass is in kilograms (kg)

The unit of acceleration is in meter per second square (m/s²)

Practical Examples

In simpler terms, the more force you apply to an object, the faster it will accelerate, while a heavier object requires more force to achieve the same acceleration.

When you push a shopping cart, the harder you push, the faster it accelerates.
In sports, athletes understand this law intuitively. A soccer player applies more force to the ball to make it accelerate quickly.

How to Derive Newton’s Second Law

According to Newton’s second law, the rate of change of momentum is proportional to the applied force. Therefore, this law can be translated into

Force (F) = change in momentum / time (t)

Change in momentum is the product of mass and velocity.

Thus,

Change in momentum = mass x velocity

Which can be further broken down into

Change in momentum = mv – mu [where v = final velocity and u = initial velocity]

Therefore, we can insert (Change in momentum = mv – mu) into [Force = change in momentum / time] to obtain

F = (mv – mu) / t

Hence,

F = m (v – u) / t

And acceleration is the rate of change of displacement

Acceleration, a = (v – u) / t

And we have F = ma which is Newton’s second law of motion.

Momentum

This is the product mass and velocity of an object. The S.I unit of momentum is kilogram meter per second (kgm/s). The formula for calculating momentum is

Momentum (M) = mass (m) x velocity (v)

The rate of change of momentum = (change in momentum) / time

Impulse of a Force

The impulse of a force is the instantaneous response of an object to the force that acts on it. We can also define impulse (I) as the product of the force and the time it takes momentum to change. The S.I unit of impulse is Newton-second (Ns).

Impulse (I) = Force (F) x Time (t)

Calculations Involving Second Law of Motion

Here are some questions to help you understand the applications of Newton’s second law of motion to solve a problem.

Problem 1

An object of mass 7.5 kilograms has a force applied to it that pulls eastward with a magnitude of 50 Newtons and another applied to it that pulls westward with a magnitude of 150 Newtons. What is the westward acceleration of the object? Give your answer to one decimal place.

Data

Mass of the object is m = 7.5 N

Initial Force, F1 = 50 Newtons

Final force, F2 = 150 Newtons

Unknown

Acceleration of the object, a = ?

Formula

The formula we need to apply to solve this problem is

F_Net = ma

But F_Net = F2 – F1

Therefore, w can further break it down into

F₂ – F₁ = ma

Solution

We can now insert our data into F₂ – F₁ = ma

150 – 50 = 7.5 x a

Make a subject of the formula

a = (150 – 50) / 7.5

Hence,

a = 100/7.5 = 13 m/s²

Problem 2

An object of mass 3 kilograms has a force of 9 Newtons applied to it. At what rate does the force accelerate the object?

Data

Mass of the object, m = 3 kg

Applied force, F = 9 N

Unknown

Acceleration, a = ?

Formula

The formula that can help us to solve this problem is

a = F/m

Solution

We can substitute our formula with our data

Acceleration, a = 9/3 = 3 m/s2

Therefore, the acceleration (a) is 3 meters per second square.

Problem 3

A boy pushes his toy car with a cat in inside. The mass of the toy car and the cat put together is 70 kilograms. The toy car accelerates at 0.9 meters per second square. Find the force that the boy applies to pull the toy car and the cat together.

Data

Mass of the toy car with a cat is m = 70 kg

Acceleration of the toy car, a = 0.9 m/s2

Unknown

Force, F = ?

Formula

We will use the formula F = ma to solve the problem

Solution

Insert the data into the above formula

F = 70 x 0.9 = 63 N

Therefore, the applied force is 63 Newtons

Third Newton’s Law of Motion

The third Newton’s law of motion states that action and reaction are equal and opposite. This law explains that when a force is applied to a body, there is a chain of equal and opposite reactions that will follow.

For example, when you blow a balloon, and later release the air entrance. The balloon will run away from you.

What if we decide to insert the balloon inside a toy canoe? We will blow the balloon and position its air entrance to face the opposite direction of the canoe’s motion. After releasing the air entrance of the balloon, it will set the toy canoe in motion on water.

Therefore, we can see that when an object X applies a force on another object Y. Object Y will equally apply the same type of force on X. Both applied forces are equal in magnitude but opposite in direction.

We can demonstrate this mathematically as

Force X = – Force Y [ Force X is the action force, and Force Y is the reaction Force]

Another example of Newton’s third law of motion is how the rocket operates. When a rocket is launched into space, it will accelerate away from the earth surface. This is thanks to the burning fuel of the rocket that kept igniting fire at the back of the rocket to push it in the upward direction.

We have many examples to demonstrate the effect of this law in our everyday life. Among these examples are:

The lift of an airplane
Student’s school bag on the desk
A hammer hitting a nail
A bird flying
A boy pushing against the wall
Applying brakes on your car
A duck swimming in the water

Everyday Examples

In other words, when one object exerts a force on another object, the second object exerts an equal and opposite force on the first.

Walking: When you walk, your foot pushes backward on the ground, and the ground pushes your foot forward, propelling you forward.
Rocket Propulsion: Rockets work by expelling high-speed exhaust gases backward. The force of the exhaust gas going backward propels the rocket forward.

Principle of Conservation of Momentum

We can state the principle of conservation of momentum in three ways:

The principle states that when two bodies that are traveling in a straight line collide, the total force before collision is equal to the total force after collision provided no external force acts on the body.
In any system of colliding bodies, the total momentum is always conserved provided that there is no net external force acting on the system.
The total momentum of an isolated or closed system of colliding bodies remains constant.

Therefore, the mathematical definition of this principle is

m₁v₁ = m₂v₂ [Where m₁v₁ is the initial momentum, and m₂v₂ is the final momentum

Now, if we have two balls M and N exerting equal forces on each other. We can say that

F_M = – F_N

And since F = ma

Then, it’s easy to say that

m_Ma_M = – m_Na_N

Additionally, a = (v – u) / t

Which implies

m_M [ (v_m – u_m) / t ] = – m_N [ (v_N – u_N) / t ]

And we will end up with

m_Mv_m – m_Mu_m = – ( m_Nv_N – m_Nu_N)

The above equation will give us

m_Mu_m + m_Nu_N = m_Mv_m + m_Nu_N

And the above expression shows that momentum before collision is equal to the momentum after collision.

Applications of Newton’s Third Law to Solve a Problem

Here are a few problems to help you understand how to solve problems that are related to newtons third law of motion, momentum, and impulse.

Problem 1

A body (P) has mass of 5 kilograms moving with a velocity of 30 m/s collides with another body (Q), moving in opposite direction with a velocity of 20 m/s. If both bodies now move in the direction of P at a velocity of 10 m/s. Calculate the mass of Q.

Newton’s Laws of Motion

Solution

To solve this problem, we need to remember that

m₁v₁ = m₂v₂

Which implies that

(m₁ + m₂)v = m₁u₁ – m₂u₂

and m₁u₁ – m₂u₂ = Before collision

(m₁ + m₂)v = After collision

Therefore, since m₁ = 5kg, u₁ = 30 m/s, m₂ = ? , u₂ = 20 m/s, v = 10 m/s

We can now insert the above information into (m₁ + m₂)v = m₁u₁ – m₂u₂ to obtain

(5 + m₂) x 10 = (5 x 30) – (m₂ x 20)

We will now have

50 + 10m₂ = 150 – 20m₂

We can now collect like terms to get

150 – 50 = 20m₂ + 10m₂

And it will become

100 = 30m₂

By making m₂ subject of the formula, we will have

m₂ = 100 / 30 = 3 kg

Therefore, the mass of Q is 3 kilograms.

Problem 2

A bullet of mass 0.05 kg is fired horizontally into a 10 kg block which is fired free to move. If both bullet block move with velocity 0.5 m/s after the impact, find the velocity with which the bullet hit the body.

Data

Mass of the bullet = 0.05 kg, mass of the block = 10 kg, and the mass of both bullet and the block = 10 + 0.05 = 10.05

Initial mass of the bullet = u

The momentum before collision = Momentum after collision

0.05 x u = 10.05 x 0.5

Hence,

u = 5.025 / 0.05 = 100.5 = 101 m/s

Problem 3

A rifle of mass 15 kilograms fires a bullet of mass 60 grams with a velocity of 200 m/s. Calculate the recoil velocity of the rifle.

Solution

m₁ = 15 kg, and m₂ = 60 g = (60/1000) kg = 0.06 kg,

v₁ = ? and v₂ = 200 m/s

We need to remember that Momentum before impact = Momentum after impact

Momentum before impact = 0

And the Momentum after impact = m₁ v₁ + m₂ v₂

Hence,

m₁ v₁ + m₂ v₂ = 0

By inserting our data, we will have

15 x v₁ + 0.06 x 200 = 0

Which will become

15 v₁ + 12 = 0

After making v₁ subject of the formula

v₁ = – (12/8) = – 0.8 m/s

Therefore, the recoil velocity is 0.8 meters per second

Isaac Newton Defined the Following Quantities in His Book

Matter: Anything that takes up space

Mass: The measure of a quantity or amount of matter

Momentum: The quantity of motion, which is the product of velocity (speed) and mass

Inertia: The power by which an object, if it is at rest, stays at rest, or if in motion, will travel in a straight line

Force: An action applied upon a body

Centripetal Force: An attraction toward the center of something (as in gravity)

We apply Newton’s laws of motion in our everyday activities.

Importance and Applications

Now that we’ve explored the laws themselves, let’s consider their practical applications in our everyday lives and various fields of science and technology.

Transportation and Engineering

Newton’s Laws are fundamental in the design of vehicles and structures. They influence:

Automobiles: Every time you hit the gas pedal or apply the brakes, you’re experiencing Newton’s Laws in action. The force you apply determines the acceleration or deceleration of your vehicle.
Aviation: In aviation, understanding the interplay of forces is essential for safe and efficient flight. Pilots must balance thrust, lift, drag, and gravity to keep an aircraft in equilibrium.
Maritime Travel: Ships, too, rely on Newton’s Laws. When a ship’s engine propels it forward, the reaction is a force that moves the ship in the opposite direction.

Sports and Athletics

Newton’s Laws govern the dynamics of sports and athletics:

Baseball: When a pitcher throws a fastball, the force applied to the ball determines its speed and trajectory. Hitters use this knowledge to time their swings.
Swimming: Swimmers maximize their efficiency by minimizing water resistance and using the principles of buoyancy and propulsion, all in line with Newton’s Laws.
Billiards: In billiards, players use the principles of angles and collisions to control the path of the balls.

Space Exploration and Astronomy

Newton’s Laws are integral to space exploration. They underpin:

Spacecraft: Rockets and spacecraft rely on these laws to propel them through the vacuum of space. Rocket engines function by expelling mass at high velocities, creating a reaction that propels the spacecraft in the opposite direction. Without Newton’s Third Law, space exploration as we know it would be impossible.

Historical Significance

The importance of Newton’s Laws is not limited to their practical applications. They also hold historical significance as they mark a turning point in the world of science.

A Scientific Revolution

Newton’s Laws were part of a broader scientific revolution that also included the works of Galileo, Copernicus, and Kepler. They offered a unified and mathematically rigorous framework for understanding motion and paved the way for classical physics.

Revolutionizing Astronomy

In addition to their impact on physics, Newton’s Laws played a pivotal role in the field of astronomy. His law of universal gravitation, which describes how all objects with mass are attracted to each other, led to the understanding of planetary motion and orbits. It was Newton’s mathematical genius that allowed scientists to predict the movement of celestial bodies with unparalleled accuracy.

Educational Impact

Newton’s Laws play a crucial role in science education and the development of critical thinking skills.

Importance of Teaching

These laws are fundamental to STEM (Science, Technology, Engineering, and Mathematics) education. They introduce students to the principles of physics and help them develop critical thinking skills. Educators emphasize the importance of teaching Newton’s Laws as a gateway to understanding the scientific method.

Resources for Learning

Numerous educational resources and tools are available to facilitate the learning of Newton’s Laws. These include interactive simulations, video lessons, and hands-on experiments that make physics accessible and engaging for students of all ages.

Newton’s Laws and the Bigger Picture

Newton’s Laws are not isolated principles; they are interconnected with other scientific concepts.

Gravity and Universal Gravitation

Newton’s Laws and the law of universal gravitation are closely related. Gravity, the force of attraction between masses, adheres to the principles set forth in Newton’s Laws. The orbits of celestial bodies, planetary motion, and the behavior of objects in free fall are all governed by a combination of these laws.

Laws of Thermodynamics

While the laws of thermodynamics primarily describe the flow of heat and energy, they are not separate from Newton’s Laws. For example, the behavior of gas particles in a container can be explained using the laws of motion as molecules collide and exert forces on one another.

Quantum Mechanics

Even in the realm of quantum mechanics, which explores the behavior of particles on the smallest scales, the foundations laid by Newton’s Laws still apply. While quantum mechanics introduces new principles, the motion of particles and the forces acting upon them remain consistent with Newton’s insights.

Quotes and Insights from Experts

To highlight the enduring importance of Newton’s Laws, let’s turn to the insights of renowned physicists and scientists.

Albert Einstein

Albert Einstein, the legendary physicist, once remarked, “You have this elementary particle, the electron, and it is attracted by positive charge, and repelled by negative charge. Newton would have despised that theory.” Einstein’s comment underscores the profound impact of Newton’s work and the subsequent developments in physics.

Richard Feynman

Richard Feynman, another Nobel laureate in physics, praised Newton’s contributions by stating, “In his day, the great truths which now seem so obvious were unknown… He took the known facts and extended our ideas to a remarkable degree in a precise quantitative way.” Feynman recognized the precision and depth of Newton’s work, which laid the groundwork for future scientific discoveries.

Interactive Demonstrations and Simulations

To truly grasp the significance of Newton’s Laws, there’s no substitute for hands-on experience. Interactive simulations and demonstrations can bring these laws to life, enabling learners to experiment and observe the principles of motion.

Hands-On Learning

We recommend exploring online platforms, such as PhET Interactive Simulations, where you can interact with virtual experiments that demonstrate the concepts of force, motion, and equilibrium. These tools offer an intuitive and engaging way to gain a deeper understanding of Newton’s Laws.

Conclusion

In conclusion, the importance of Newton’s Laws of Motion cannot be overstated. These fundamental principles, introduced by the genius of Sir Isaac Newton, continue to shape our understanding of the physical world. They govern everything from the way we travel to the devices we use, from the structures we build to the scientific inquiries we pursue.

The enduring significance of Newton’s Laws is a testament to their timeless relevance in science, technology, and education. As we journey further into the future, we carry with us the profound insights provided by these laws, reminding us of the boundless possibilities that lie ahead.

Call to Action

We encourage you to share this article with others who may be interested in the significance of Newton’s Laws. Additionally, if you have any questions or would like to share your insights on this topic, please leave a comment below. For those who wish to dive deeper into physics and scientific exploration, we recommend exploring the extensive resources available at academic institutions, physics organizations, and reputable educational websites.

References and External Links

We hope you’ve enjoyed this comprehensive exploration of the importance of Newton’s Laws of Motion. Your journey into the world of physics has only just begun, and we invite you to continue your quest for knowledge and understanding.

Sources