What is Speed?
Definition of Speed: Speed is the rate of change of distance with time. Speed is different from velocity because it’s not in a specified direction. In this article, you will learn how to solve speed, velocity, and acceleration problems.
Additionally, you need to know that speed is a scalar quantity and we can write its symbol as S. The formula for calculating the speed of an object is:
Speed, S = Distance (d) / Time (t)
Thus, s = d/t
Note: In most cases, we also use S as a symbol for distance.
The S.I unit for speed is meter per second (m/s) or ms-1
Non-Uniform or Average Speed: This is a non-steady distance covered by an object at a particular period of time. We can also define non-uniform speed as the type of distance that an object covered at an equal interval of time.
The formula for calculating non-uniform speed is
Average speed = Total distance covered by the object / Total time taken
Actual speed: This is also known as the instantaneous speed of an object which is the distance covered by an object over a short interval of time.
You may also like to read:
How to Find Displacement in Physics
also How to Find a Position in Physics
and How to Calculate Bearing in Physics
What is Velocity?
Definition of Velocity: Velocity is the rate of displacement with time. Velocity is the speed of an object in a specified direction. The unit of velocity is the same as that of speed which is meter per second (ms-1). We use V as a symbol for velocity.
Note: We often use U to indicate initial speed, and V to indicate final speed.
The formula for calculating Velocity (V) = displacement (S) / time (t)
The difference between velocity and speed is the presence of displacement and distance respectively. Because displacement is a measure of separation in a specified direction, while distance is not in a specified direction. Velocity is a vector quantity.
Uniform Velocity
Definition of uniform velocity: The rate of change of displacement is constant no matter how small the time interval may be. Also, uniform velocity is the distance covered by an object in a specified direction in an equal time interval.
The formula for uniform velocity = Total displacement / Total time taken
What is Acceleration?
Definition of Acceleration: Acceleration is the rate of change of velocity with time. Acceleration is measured in meters per second square (ms-2). The symbol for acceleration is a. Acceleration is also a vector quantity.
The formula for acceleration, a = change in velocity (v)/time taken (t)
Thus, a = v/t
We can also write acceleration as
a = change in velocity/time = ΔV/Δt = (v – u)/t
[where v = final velocity, u = initial velocity, and t = time taken]
Uniform Acceleration
In the case of uniform acceleration, the rate of change of velocity with time is constant.
Average velocity of the object = (Initial velocity + final velocity)/2
or
Average velocity = (v + u)/2
What is Retardation?
Retardation is the decreasing rate of change in velocity moved, covered, or traveled by an object.
The formula for calculating retardation is
Retardation = Change in a decrease in velocity/time taken
Equations of Motion
You can also apply the following equations of motion to calculate the speed, velocity, and acceleration of the body:
- v = u + at
- s = [ (v + u)/2 ]t
- v2 = u2 + 2as
- s = ut + (1/2)at2
Where v = final velocity, u = initial velocity, t = time, a = acceleration, and s = distance
You may also like to read:
How to Calculate Maximum height
How to Calculate Dimension in Physics
Solved Problems of Speed, Velocity, and Acceleration
Here are solved problems to help you understand how to calculate speed, velocity, and acceleration:
Problem 1
A train moves at a speed of 54km/h for a one-quarter minute. Find the distance travelled by train.
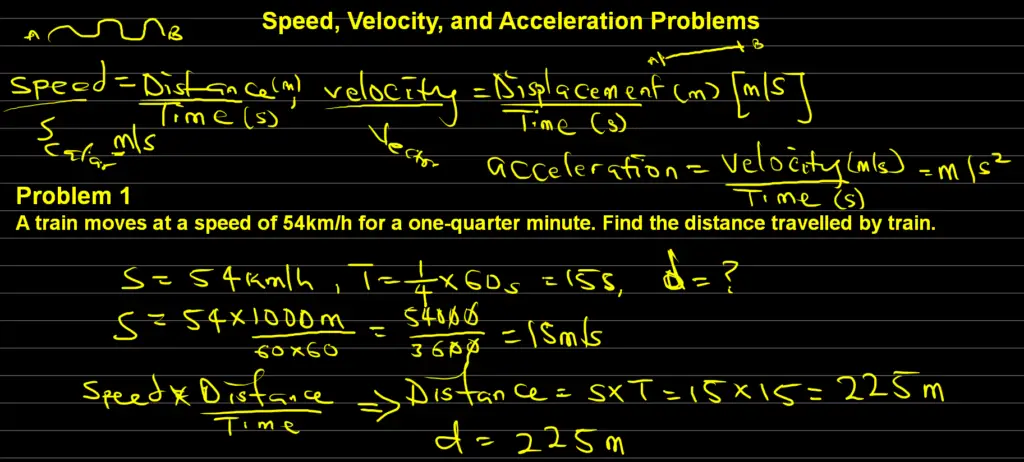
Solution
Data
From the question above
Speed = 54 km/h = (54 x 1000)/(60 x 60) = 54,000/3,600 = 15 m/s
Time = one quarter minute = 1/4 minute = (1/4) x 60 = 15 seconds
Since we have
speed = distance/time
After cross-multiplication, we will now have
Distance = speed x time
We can now insert our data into the above expression
Distance = 15 m/s x 15 s = 225 m
Therefore, the distance travelled by train is 225 meters.
Problem 2
A car travelled a distance of 5km in 50 seconds. Find the speed in meters per second.

Solution
Data:
Distance = 5km = 5 x 1000m = 5,000m
Time = 50 seconds
speed = ?
and the formula for speed
speed = distance/time = 5000/50 = 100m/s
Problem 3
A motorcycle starting from rest moves with a uniform acceleration until it attains a speed of 108 kilometres per hour after 15 seconds. Find its acceleration.
Solution
Data:
From the question above
Initial velocity, u = 0 (because the motorcycle starts from rest)
Final velocity, v = speed = 108 km/h = (108 x 1000m) / (60 x 60s) = 108,000/3,600 = 30m/s
Time taken, t = 15 seconds
Therefore, we can now apply the formula that says
Acceleration = change in velocity/ time = (v-u)/t = (30-0)/15 =30/15 = 2ms-2
Therefore, the acceleration of the motorcycle is 2ms-2
Problem 4
A bus covers 50 kilometres in 1 hour. What is it is the average speed?
Solution
Data
Total distance covered = 50 km = 50 x 1000m = 50,000m
Time taken = 1 hour = 1 x 60 x 60s =3,600s
Average speed = Total distance covered by the object / Total time taken
Therefore, we can now calculate the average speed of the bus by substituting our data into the above formula
Hence,
Average speed = 50,000/3,600 = 13.9 m/s
Therefore, the average speed of the bus is 13.9m/s or approximately 14 meters per second.
Problem 5
A car travels 80 km in 1 hour and then another 20 km in the next hour. Find the average velocity of the car.

Solution
Data:
Initial displacement of the car = 80km
Final displacement of the car = 20km
The total displacement of the car = initial displacement of the car + final displacement of the car
Therefore,
The total displacement of the car = 80km + 20km = 100km
Also,
The time for 80km is 1hr
And the time for 20km is 1hr
Total time taken = The time for 80km (1hr) + The time for 20km (1hr)
Hence
Total time taken = 1hr + 1hr = 2hrs
Now, to calculate the average velocity of the car, we apply the formula that says
Average velocity = total displacement/total time taken = 100km/2hrs = 50km/h
We can further convert the above answer into meters per second
Average velocity = 50km/h = (50 x 1000m)/(1 x 60 x 60s) = 50,000/3,600 = 13.9m/s or 14ms-1
Therefore, the average velocity of the car is 14ms-1
You may also like to read:
How to Conduct Physics Practical
and How to Calculate Velocity Ratio of an Inclined Plane
Problem 6
A body falls from the top of a tower 100 meters high and hits the ground in 5 seconds. Find its acceleration.
Solution
Data
Displacement = 100m
Time = 5 seconds
and we can apply the formula for acceleration that says
acceleration, a = Displacement/time2 = 100/52 = 100/25 = 4ms-2
Therefore the acceleration due to the gravity of the body is 4ms-2
Note: The acceleration due to the gravity of a body on the surface of the earth is constant (9.8ms-2), even though there may be a slight difference due to the mass and altitude of the body.
Problem 7
An object is thrown vertically upward at an initial velocity of 10ms-1 and reaches a maximum height of 50 meters. Find its initial upward acceleration.
Solution
Data
Initial velocity, u = 10ms-1
Final velocity, v = 0
maximum height = displacement = 50m
Initial upward acceleration, a =?
When we apply the formula that says a = (v2 – u2)/2d we will have
a = (0 – 102)/(2 x 50) = -100/100 = -1 ms-2
Hence, since our acceleration is negative, we can now say that we are dealing with retardation or deceleration.
Therefore, the retardation is -1ms-2
Note: Retardation is the negative of acceleration, thus it is written in negative form.
Problem 8
A car is traveling at a velocity of 8ms-1 and experiences an acceleration of 5ms-2. How far does it travel in 4 seconds?
Solution
Data:
Initial velocity, u = 8ms-1
acceleration, a = 5ms-2
Distance, s =?
time, t = 4s
We can apply the formula that says
s = ut + (1/2)at2
Thus
s = 8 x 4 + (1/2) x 5 x 42
s = 32 + (1/2) x 80 = 32 + 40 = 72m
Therefore, the distance covered by the car in 4s is 72 meters.
Problem 9
A body is traveling at a velocity of 10m/s and experiences a deceleration of 5ms-2. How long does it take the body to come to a complete stop?
Solution
Data
Initial velocity, u = 10m/s
Final velocity, v = 0
acceleration , a = retardation = -5ms-2
time, t = ?
We already know that acceleration, a = change in velocity/time
This implies that
Time, t = change in velocity/acceleration
Thus
t = (v – u)/t = (0-10)/-5 = -10/-5 = 2s
Therefore, the time it takes the car to stop is 2 seconds.
Problem 10
A body is traveling at a velocity of 20 m/s and has a mass of 10 kg. How much force is required to change its velocity by 10 m/s in 5 seconds?
Solution
Data
Change in velocity, v =10 m/s
mass of the object, m = 10kg
time, t = 5s
We can apply newton’s second law of motion which says f = ma
and since a = change in velocity/time
we will have an acceleration equal to
a = 10/5 = 2ms-2
Therefore, to find the force, we can now say
f = ma = 10 x 2 = 20N
Therefore, the force that can help us to change the velocity by 10 m/s in 5 seconds is 20-Newton.
Drop a comment if there is anything you don’t understand about speed, velocity, and acceleration Problems.
You may also like to read:
How to Calculate Cost of Electricity Per kwh
also How to Calculate the Efficiency of a Transformer
and How to Calculate Escape Velocity of a Satellite
Check our Website:
Wokminer – Apply for jobs