What is Centripetal Force?
Definition of Centripetal force: Centripetal force is the force of an object around a circular path that keeps the object moving at a constant speed in a circular path. When a body is under the effect of centripetal force, the direction of the motion of the object will continue to change. The symbol for centripetal is FT. Additionally, the Si unit of centripetal force is in Newton. In this article, you will learn how to calculate the centripetal force of an object. To learn how to calculate centripetal force, we need to understand important concepts like centripetal force definition, centripetal force symbol, centripetal force unit, centripetal force formula, and then how to apply this formula to calculate centripetal force. Therefore, I tried my best to break the topic in a manner you will get all the information you need including a video tutorial below.
Explanation: Centripetal Force
Similarly, we can equally define centripetal force as the force that acts on an object moving in a circular path, directing it towards the centre of that path. It is responsible for preventing the object from moving in a straight line tangent to the circle. This inward force allows the object to continually change direction without leaving the circular path.
Centripetal force is a fundamental concept in physics that describes the force that acts on an object moving in a circular path to keep it in that path. This force is directed toward the center of the circle around which the object is moving. Centripetal force is not a distinct or separate force in itself; rather, it is the result of other forces, such as tension, gravity, or friction, that must act on the object to keep it in circular motion.
Role in Circular Motion
Imagine swinging a ball attached to a string around in a circle. The string pulls the ball toward the centre of the circle, creating a force that allows the ball to maintain its circular trajectory. This force is none other than centripetal force, an essential component of circular motion.
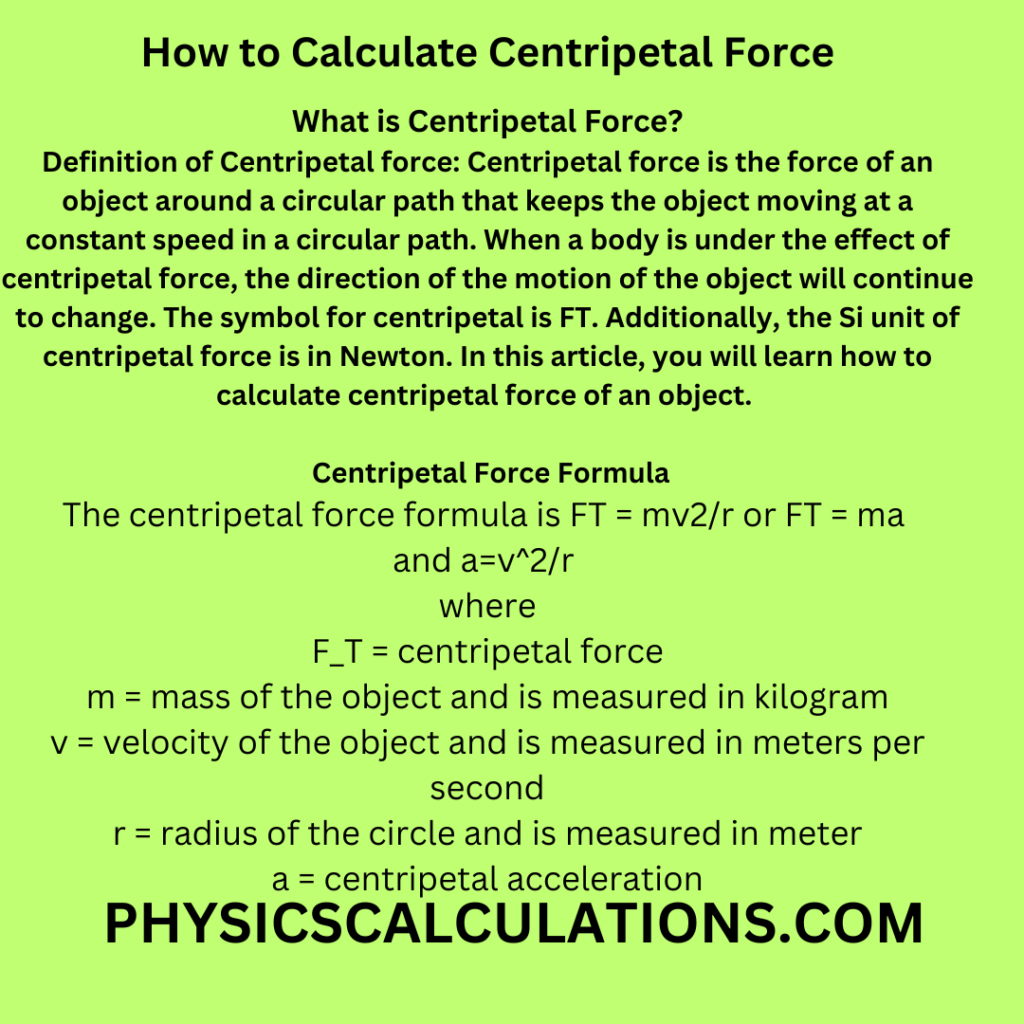
Centripetal Force Formula
The centripetal force formula is FT = mv2/r or FT = ma and a=v2/r
where
FT = centripetal force
m = mass of the object and is measured in kilogram
v = velocity of the object and is measured in meters per second
r = radius of the circle and is measured in meter
Derivation and Components
Centripetal acceleration can be derived from the equation for linear velocity (v) in a circular path: a = v2 / r, where r is the radius of the circle. This acceleration is always directed toward the centre of the circle, creating the need for the centripetal force.
Units of Measurement
The units of centripetal force depend on the units of mass (kg) and acceleration (m/s²). In the International System of Units (SI), the unit of force is the newton (N), which is equivalent to 1 kg m/s².
Centripetal force is perpendicular to the direction of the velocity of the object and it’s an inward force towards the center of the circle. This force keeps the body moving around the circle at a constant speed.
Examples of Centripetal Force
Examples of centripetal force are as follows:
- The way the moon moves around the earth
- How the earth moves around the sun
- The way an attached stone to a wheel keeps going around the wheel while in motion.
Solved Problems: How to Calculate Centripetal Force
Here are a few solved problems of how to calculate centripetal force:
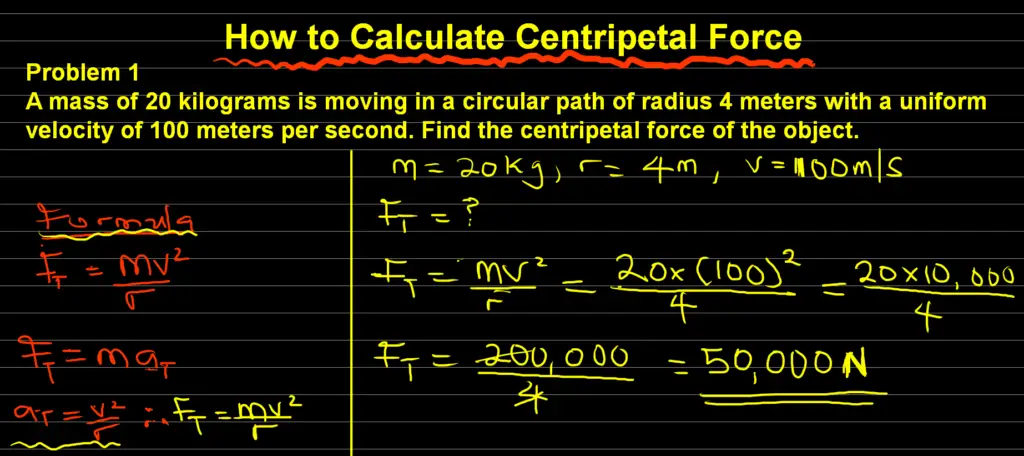
Problem 1
A mass of 20 kilograms is moving in a circular path of radius 4 meters with a uniform velocity of 100 meters per second. Find the centripetal force of the object.
Solution
Data:
mass, m = 20 kg
radius, r = 4 m
centripetal force, FT = ?
uniform velocity, v = 100 m/s
Centripetal force is calculated by using the formula that says
Force = mass x acceleration
and the formula for centripetal acceleration is a=v2/r
Which implies that a = 1002/4 = 2,500ms-2
Since FT = ma
we can now say that
FT = 20 x 2,500 = 50,000 N
Therefore, the centripetal force FT is 50,000 Newtons
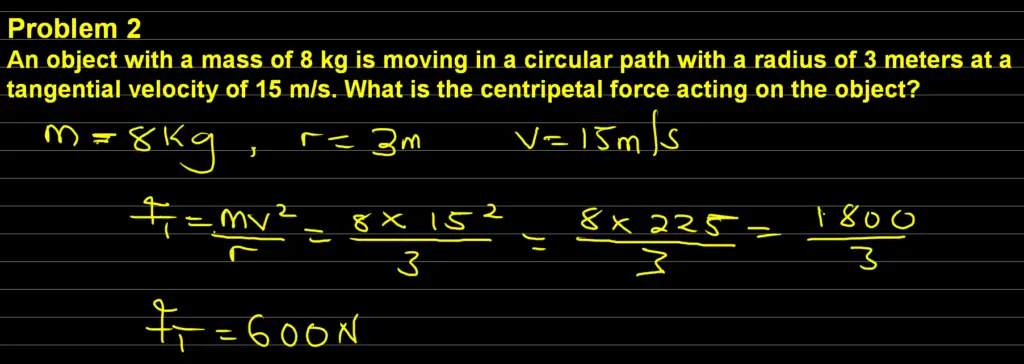
Problem 2
An object with a mass of 8 kg is moving in a circular path with a radius of 3 meters at a tangential velocity of 15 m/s. What is the centripetal force acting on the object?
Solution
Data:
Mass, m = 8 kg
Radius, r = 3 m,
Velocity, v = 15 m/s
Centripetal force, FT =?
and the formula for calculating centripetal force is FT = mv2/r
We can now substitute our data into the above formula to get
FT = (8 x 152)/3 = (8 x 225)/3 = 1,800/3 = 600 N
Therefore, the centripetal force is 600 Newtons.
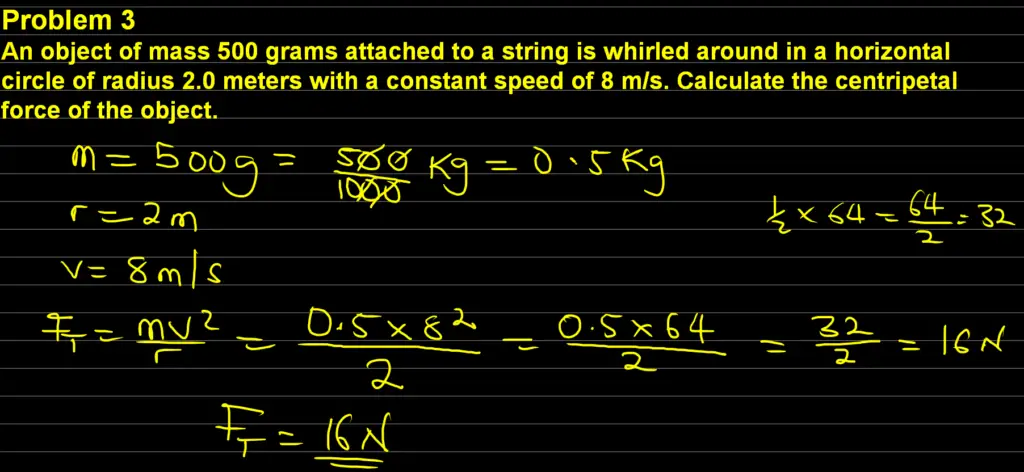
Problem 3
An object of mass 500 grams attached to a string is whirled around in a horizontal circle of radius 2.0 meters with a constant speed of 8 m/s. Calculate the centripetal force of the object.
Solution
Data:
Mass, m = 500 g = 500/1000 kg = 0.5 kg
radius, r = 2.0 m
Speed, v = 8 ms-1
FT =?
While FT = mv2/r
We can now plugin our data into the above formula
FT = (0.5 x 82)/2 = 32/2 = 16 N
Therefore, the centripetal force is 16 Newtons
Theoretical Underpinnings of Centripetal Force
Newton’s First Law and Inertia
We need to know that Newton’s laws of motion form the foundation of our understanding of the centripetal force. Newton’s first law states that an object at rest stays at rest, and an object in motion continues in motion with constant velocity unless acted upon by a net external force. In circular motion, this external force is the centripetal force, preventing the object from following its natural straight-line path.
Newton’s Second Law and Force
Newton’s second law relates force, mass, and acceleration. In the case of centripetal force, acceleration is directed toward the centre of the circle, and the force required to maintain this acceleration is the centripetal force.
Centripetal Force vs. Centrifugal Force
A common misconception is the idea of a “centrifugal force” pushing objects outward in circular motion. However, there’s no actual outward force—what we perceive as a centrifugal force is simply the result of inertia trying to keep objects moving in straight lines while they’re constrained to circular paths.
Real-World Examples
Planetary Motion
The dance of planets around the sun is a classic example of centripetal force in action. Gravity acts as the centripetal force, keeping planets in their elliptical orbits. Johannes Kepler’s laws of planetary motion provide mathematical descriptions of these orbits.
Artificial Satellites
Satellites orbiting the Earth, whether in geostationary or low Earth orbits, rely on centripetal force to maintain their trajectories. This force ensures that they don’t drift off into space or plummet back to Earth.
Carnival Rides and Amusement Parks
The exhilarating twists and turns of amusement park rides are orchestrated by centripetal force. Roller coasters, ferris wheels, and spinning rides all employ carefully calculated centripetal forces to provide thrilling and safe experiences for riders.
Car Racing and Banked Turns
In the world of car racing, the design of banked turns is a prime example of utilizing centripetal force. The banking angle and speed of the car work together to create a centripetal force that keeps the car on the track during turns.
String Instruments and Circular Motion
Musical instruments like violins and guitars produce sound through the vibrations of strings. These strings undergo circular motion when plucked or bowed, creating harmonic tones due to centripetal force.
Centripetal Force in Nature
Tornadoes and Hurricanes
Atmospheric phenomena like tornadoes and hurricanes exhibit circular motion driven by centripetal force. The Coriolis effect, caused by the Earth’s rotation, influences the direction of these storms.
Ocean Currents
Large oceanic gyres and currents are a result of centripetal forces driven by winds, temperature differences, and Earth’s rotation. These currents have far-reaching effects on climate and marine ecosystems.
Engineering and Technological Applications
Centrifuges in Laboratories
Centrifuges are used in laboratories to separate substances of different densities. The centripetal force generated causes denser particles to move outward while lighter substances stay closer to the center.
Designing Curved Roadways
Engineers design curved roadways with carefully calculated banking angles to ensure that vehicles can navigate turns safely and comfortably. Centripetal force plays a crucial role in preventing accidents.
Importance in Everyday Life
Centripetal force isn’t just a theoretical concept—it plays a vital role in numerous aspects of our daily lives. From the way cars navigate turns to the science behind amusement park rides, centripetal force is everywhere, shaping the way objects move in circular paths.
Centripetal Force vs. Gravity
Differentiating Between Forces
While gravity is the force of attraction between masses, centripetal force is the force that acts perpendicular to an object’s velocity, keeping it in a curved path.
Mutual Interaction in Celestial Bodies
Celestial bodies, such as planets and stars, interact through both gravitational and centripetal forces, shaping their orbits and motions within galaxies.
Misconceptions and Common Pitfalls
Misunderstanding Centrifugal Force
The notion of an outward-pushing centrifugal force is a misunderstanding. In reality, it’s inertia that makes objects “want” to move in straight lines.
Confusion with Tangential Velocity
Tangential velocity is the speed of an object along its circular path. It’s not the same as centripetal force, which is the inward force responsible for changing the direction of motion.
Key Points About Centripetal Force
- Direction: Centripetal force always acts inward and toward the center of the circular path. This inward force prevents the object from moving in a straight line and instead causes it to continuously change direction while maintaining its circular motion.
- Balanced Forces: In a uniform circular motion, there is a balance between centripetal force and the object’s inertia. This balance is necessary to keep the object on its circular path. If the centripetal force were to disappear, the object would move in a straight line due to its inherent tendency to do so (in accordance with Newton’s first law of motion).
- Types of Centripetal Forces: The specific force responsible for providing centripetal force depends on the situation. For example:
- Gravity can act as a centripetal force when an object orbits a planet or star.
- Tension in a string can provide a centripetal force to keep an object moving in a circle when swung around.
- Friction between a car’s tires and the road can provide the centripetal force needed to make a turn.
- In a centrifuge, the centripetal force is provided by mechanical means to separate substances based on their density.
You may also like to read:
How to Calculate the Cost of Electricity Per kWh
and How to Calculate Escape Velocity of a Satellite
Check our Websites:
Wokminer – Apply for jobs