What is Centripetal Acceleration?
Definition: Centripetal acceleration is the type of acceleration that is directed toward the centre of a circular path. It is si unit is in meters per second square (ms-2), and its symbol is a.
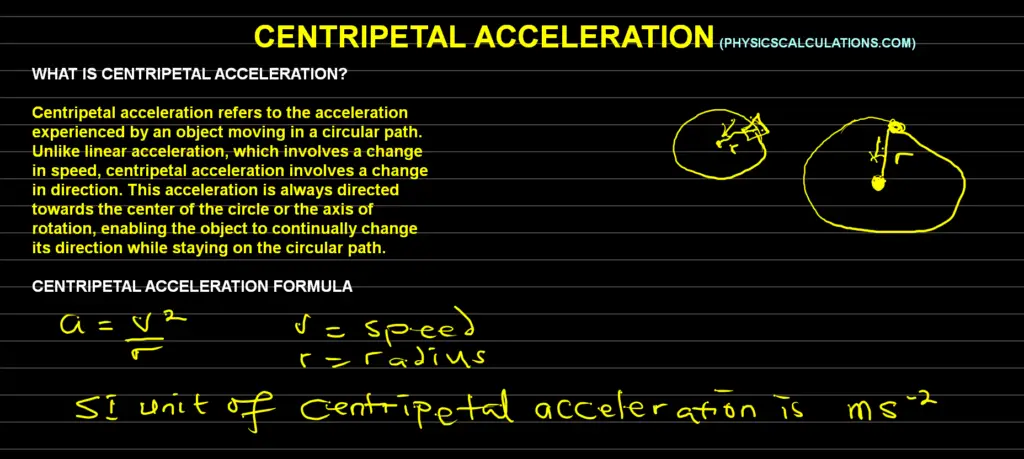
Additionally, we can define centripetal acceleration as the acceleration experienced by an object moving in a circular path. Unlike linear acceleration, which involves a change in speed, centripetal acceleration involves a change in direction. This acceleration is always directed towards the center of the circle or the axis of rotation, enabling the object to continually change its direction while staying on the circular path.
The centripetal acceleration formula is: a = v2/r or a = ω2r
[where a = centripetal acceleration, v = speed, ω = angular velocity, and r = radius of the circular path]
When you enter a merry-go-round, it will go around a circular path about an axis. its continuous movement would make you feel like you are being pushed outside. You are experiencing this outward push because the merry-go-round keeps changing direction in a circular path while your body wants to continue moving in a straight line.
Therefore, as the object keeps rotating, centripetal force provides sufficient force to help your body continue moving around the circular path. Additionally, centripetal acceleration is directed toward the centre of the circular path.
Many people kept asking a question as to whether centripetal acceleration is constant. The answer is Yes, and this is because the speed of the object is constant in magnitude but its direction is changing along a circular path. Thus, it is important to know that this type of acceleration is experienced by any object undergoing circular motion. It’s always directed toward the centre.
Centripetal Acceleration Derivation
The acceleration of an object moving in a circle:
- Is always directed toward the center of the circle.
- It has a constant magnitude
Therefore, we can derive the mathematical equation using the diagram below

The change in velocity Δv is directed toward the centre, and as such perpendicular, to v. Since the magnitude of the velocity at A and B are the same, despite their direction.
Therefore, the change in velocity Δv = vsinθ
As the angular change and the time to make the change are now smaller,
sinθ = θ
Therefore,
Δv = vθ
We need to also remember that acceleration is the change of velocity over time. Hence
a = Δv / t
Which will now become
a = vθ / t [Because Δv = vθ]
and the formula for angular velocity is ω = θ / t [θ =ωt]
a = vωt / t
Thus, we will now have
a = vω and ω = v/r which will give us a = (v x v) / r. Therefore, a = v2 / r
Additionally, since v = ωr
a = ωr x ω
Therefore, the centripetal acceleration formula for are:
a = ω2r or
a = v2 / r
How to Calculate Centripetal Acceleration
Here are a few solved problems:
Problem 1
A mass of 15 kilograms is moving in a circular path of radius 3 meters with a uniform speed of 30 meters per second. Find the centripetal acceleration of the object.

Solution
Data:
Mass, m = 15 kg,
Radius, r = 3 m, and
Uniform speed, v = 30 m/s
Centripetal accelaration, a =?
We can apply the formula that says a = v2/r
Therefore, we can now substitute our data into the above formula
a=v2/r = 302/3 = 900/3 = 300ms-2
Therefore, the centripetal acceleration of the body is 300 meters per second square (a=300ms-2).
Problem 2
A bus is travelling at a speed of 70 meters per second and is making a turn with a radius of 100 meters. What is the magnitude of the bus’ centripetal acceleration?
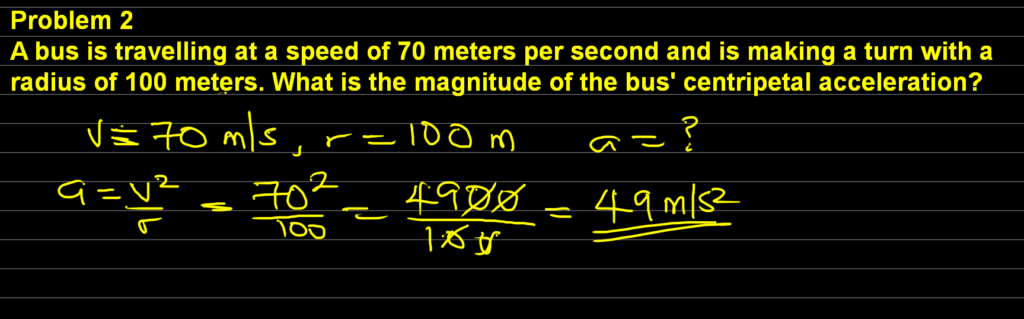
Solution
Data:
Speed, v = 70 m/s,
Radius, r = 100 m,
Centripetal acceleration, a =?
Since the formula is a=v2/r
We can now say that
a=702/100 = 4,900/100 = 49ms-2
Hence, the centripetal acceleration of the bus is 49 meters per second square (a=49ms-2)
Problem 3
An aeroplane is flying in a circular path at a speed of 400 meters per second and a radius of 1000 meters. Find the centripetal acceleration of the aeroplane.
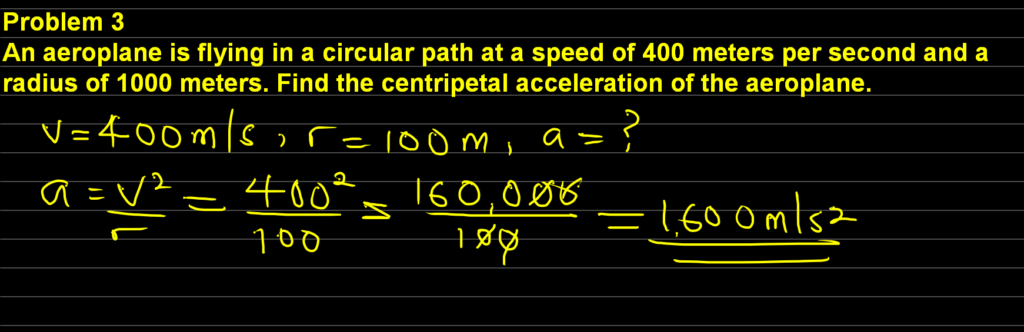
Solution
Data:
Speed, v = 400 m
Radius, r = 1000 m
Centripetal acceleration a=?
We can apply the formula a=v2/r to solve the problem
Hence, substitute our data into the above formula
a=v2/r = 4002/1000
Thus
a = 160,000/1000 = 160ms-2
Therefore, the centripetal acceleration of the aeroplane is 160 meters per second square (160ms-2)
Problem 4
A roller coaster makes a turn of 40 meters and a speed of 20 meters per second. Determine the centripetal acceleration of the roller coaster.
Solution
Data
The radius of the roller coaster, r = 40 m
Speed, v = 20 m/s
Centripetal acceleration, a =?
and the formula that will help us solve the problem is a=v2/r
After plugin our data into the above formula, we will get
a=v2/r = 202/60 = 400/40 = 10 ms-2
Therefore, the final answer is 10 meters per second square (10 ms-2).
Problem 5
A body moves along a circular path with a uniform angular speed of 0.7 rad/s and at a constant speed of 4.0 m/s. Calculate the acceleration of the body toward the centre of the circle.
Answer
Data:
Angular speed, ω = 0.7 rad/s
v = 4.0 m/s
since v = ωr
Therefore, radius r = v / ω = 4 / 0.7 = 5.7 m
Hence, the acceleration toward the centre is
a = v2 / r = 42 / 5.7 = 16 / 5.7 = 2.80 m/s2
Therefore, the acceleration toward the centre of the circle is 2.8 meters per second square (m/s2)
NOTE:
Centripetal force (f) is defined as the force that tends to keep an object of mass (m), around a circular path of radius (r). The formula for centripetal force is f = mv2/r.
Centrifugal force is the reaction force that tends to move a body away from the centre. In other words, it acts in the opposite direction to the centripetal force which can be written as (- mv2/r).
A centrifuge is a device used to separate particles in suspension from the liquid in which they are contained. It consists of two tubes that are whirled by electrical means in a circle at a uniform speed. When you use the centrifuge, particles suspended in the less dense liquid would be observed to collect at the bottom of the centrifuge tube. It will leave the clear liquid at the top. While those in a denser liquid will collect at the top leaving the clear liquid at the bottom.
Car Negotiating a Curve
Imagine you are driving a car around a curved road. How do you determine the centripetal acceleration required to keep the car on the curve without skidding?
To calculate a, you will need the car’s speed (v) and the radius (r) of the curve.
The formula is:
a = v2 / r
For instance, if you are driving at 60 mph (26.8 m/s) around a curve with a radius of 50 meters, a is
a = (26.8)2 / 50 ≈ 14.31 m/s2
Swing Ride at an Amusement Park
Consider a swing ride at an amusement park. Riders are rotating at a constant speed on swings that are 6 meters from the centre. How can you find the centripetal acceleration experienced by the riders?
Using the same formula, plug in the values:
a = v2 / r
If the swings rotate at 10 m/s, a will be
a = (10)2 / 6 ≈ 16.67 m/s2
This value indicates the acceleration towards the centre that prevents riders from flying off due to inertia.
Satellite in Orbit
Delving into more advanced scenarios, let’s discuss a satellite orbiting the Earth. The centripetal acceleration in this context is caused by the gravitational pull of the Earth and is given by:
a = G.M / r2
Where G is the gravitational constant, M is Earth’s mass, and r is the satellite’s distance from the Earth’s centre. Solving such problems requires an understanding of the gravitational forces at play.
Expert Tips for Problem Solving
- Draw Diagrams: Visual representations can clarify complex problems. Sketching the scenario aids in identifying key variables and relationships.
- Units Matter: Pay close attention to units when plugging values into formulas. Inconsistent units can lead to incorrect results.
- Practice Algebra: Many of it is problems involve algebraic manipulation. Brush up on algebraic techniques to simplify equations effectively.
- Newton’s Laws: Understand Newton’s laws of motion. These laws provide the foundation for solving various circular motion problems.
Applications of Centripetal Acceleration
Centripetal acceleration finds applications in various fields, shaping our understanding of the physical world:
- Automotive Safety: In the design of roads and curves, engineers use it for calculations to ensure vehicles can safely navigate turns without skidding.
- Amusement Parks: Roller coasters and other rides rely on centripetal acceleration to provide thrilling experiences while keeping passengers safe.
- Astronomy: Understanding this concept helps astronomers analyze the motion of planets, moons, and other celestial bodies within their orbits.
- Sports: Athletes in sports like gymnastics and figure skating leverage centripetal acceleration to execute impressive spins and routines.
- Physics Experiments: Centripetal acceleration plays a role in experiments involving rotating platforms or objects.
The Role of Centripetal Acceleration in Gravity
The concept of centripetal acceleration is closely linked to gravity, as it is the centripetal force that keeps planets and other objects in orbit around massive bodies like stars. This force counteracts the natural tendency of objects to move in a straight line and explains why celestial bodies maintain their elliptical paths over time.
FAQ’s
How is centripetal acceleration different from regular acceleration?
Centripetal acceleration involves a change in direction while moving in a circular path, whereas regular acceleration (linear acceleration) involves a change in speed in a straight line.
Can centripetal acceleration exist without centripetal force?
No, it is a result of the centripetal force. Without this force, an object would move tangentially to the circular path.
What is the relationship between centripetal acceleration and radius?
The relationship is inversely proportional. As the radius of the circular path decreases, a increases, given a constant velocity.
Are there any instances where centripetal acceleration is not applicable?
It is applicable to any object moving in a circular path, as long as there’s a force directed toward the centre of the circle.
How does centripetal acceleration relate to Newton’s laws of motion?
It is a consequence of Newton’s laws of motion. It’s a manifestation of the force required to keep an object in circular motion, as explained by the first law.
What happens if centripetal force is greater than centripetal acceleration?
Centripetal force is the cause, and centripetal acceleration is the effect. If the force is greater, the acceleration will also increase, potentially leading to a tighter circular path.
You may also like to read:
How to Calculate Centripetal Force
Reference