1. What is Viscosity?
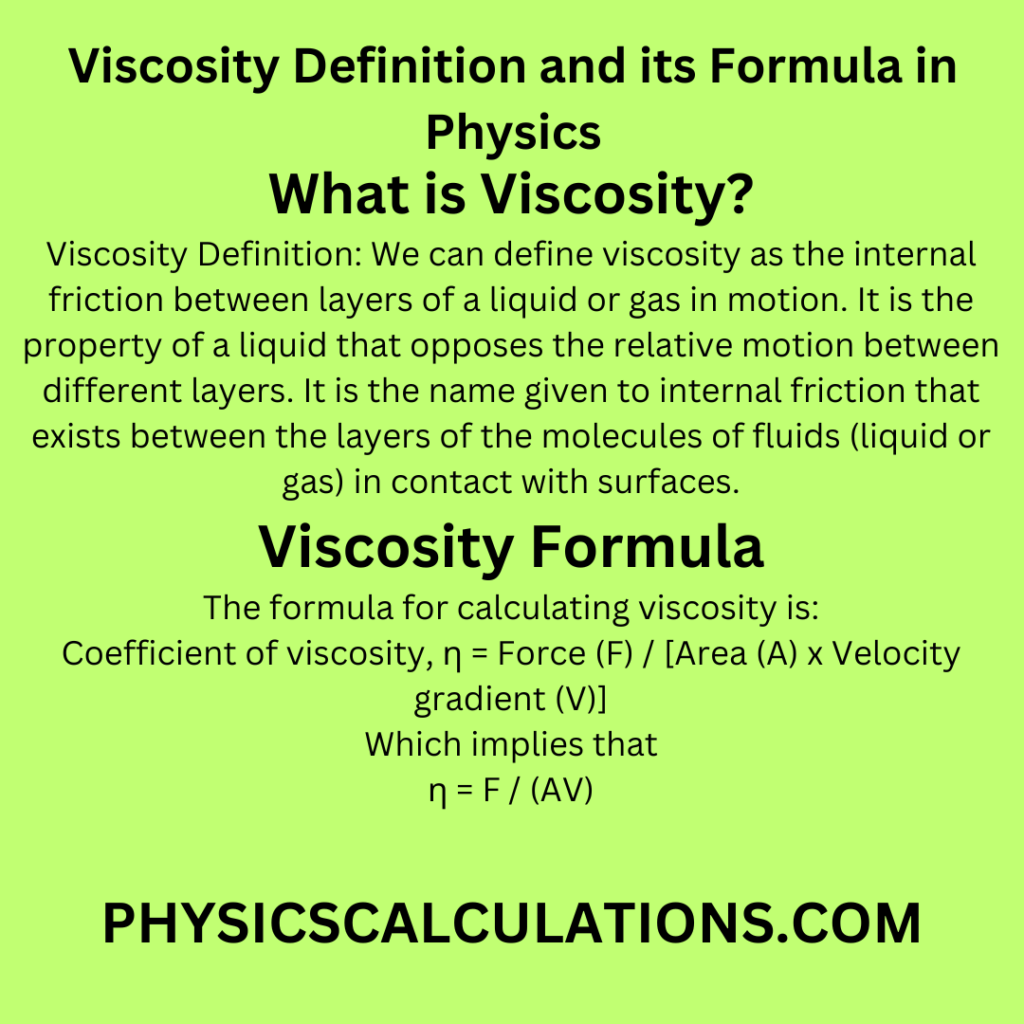
Viscosity Definition: We can define viscosity as the internal friction between layers of a liquid or gas in motion. It is the property of a liquid that opposes the relative motion between different layers. It is the name given to internal friction that exists between the layers of the molecules of fluids (liquid or gas) in contact with surfaces.
Additionally, viscosity, in fluid dynamics, is the measure of a fluid’s resistance to flow. This essential property helps us understand how different fluids behave and interact with their surroundings. When we observe fluids in motion, we are witnessing the effects of viscosity, which manifests as the resistance these fluids offer to any attempt to move them.
At its core, viscosity arises due to the relative motion between layers of a fluid. Imagine these layers as sheets of material sliding past one another when a fluid is in motion. The internal friction between these layers leads to the resistance we perceive as viscosity. In simpler terms, viscosity can be thought of as a measure of a fluid’s thickness or its ability to hinder the passage of objects through it.
2. Understanding Viscosity
For a proper explanation, let us pour water or kerosene into a bowl. Clean the bowl and pour honey into the same bowl. You will observe that it’s very easy to pour water into the bowl than to pour honey. The difference is because of the thickness of the honey which makes it very hard to move from one container to another.
When you drop a little stone into a container of water, it gets to the bottom of the container very easily and faster. Now, drop the same size of stone into a container of honey with the same volume of water. You will realize that the stone will get to the bottom of the container very slowly because of the thickness of the honey.

Therefore, the differences are due to the internal friction between the layers of the fluids. The honey is thicker and encourages more internal friction between its layers. We need to remember that friction is a force that resists motion. Hence, honey tends to resist the motion of objects between its layers due to its thickness. The thicker a liquid is, the higher its viscosity.
We consider liquids that pour slowly to be more viscous than those which pour faster. Hence very cold thick palm oil is more viscous than very cold water. Therefore, we can say that viscosity is friction in liquids.
3. Viscosity Definition: What is the SI Unit of Viscosity and its Formula
The formula for calculating viscosity is:
Coefficient of viscosity, η = Force (F) / [Area (A) x Velocity gradient (V)]
Which implies that
η = F / (AV)
When layers of water flow slowly and steadily through a pipe, it will be observed that each layer flows at different velocities. if:
- The velocities of adjacent layers A and B are V1 and V2 respectively
- The distance between them is h
- Therefore, the force exerted between the two layers will be directly proportional to the velocity gradient and the surface area A
F ∝ ( V1 – V2)A / h
Which will later become
F = η( V1 – V2)A / h
η = Constant of proportionality called the coefficient of viscosity
Another formula to apply is
Viscosity = shear stress / shear strain
We measure viscosity in Newton seconds per meter square (Ns/m2) or in Pascal seconds (Pas), and it’s a vector quantity. The derived standard international (SI) unit of dynamic viscosity is poiseiulle (PI).
4. Dimension of Viscosity
We can derive the dimension of viscosity by applying the formula
η = F / (AV)
Where Force, F = Newtons = kgm/s2 = MLT-2
Area, A = m2 = L2
Velocity, V = m/s = LT-1
Hence, by using η = F / (AV) we will have
η = F / (AV) = MLT-2 / (L2 x LT-1) = ML-1T-1
Therefore, we have dimension as ML-1T-1.
5. Types of Viscosity
We have two types of viscosity: Dynamic and kinematic
a. Dynamic Viscosity: This is the resistance to the flow of one layer of fluid in relation to the other. This is a force that is required by the fluid to defeat the internal molecular friction so that it can encourage the flow of the fluid. Hence, fluids move in the opposite direction at different speeds
b. Kinematic Viscosity: This is the measure of the flow of the fluid’s internal resistance as a result of the influence of the force of gravity.
a. Dynamic Viscosity
Dynamic viscosity, also known as absolute viscosity, is one of the two key ways we measure viscosity. It’s the measure of a fluid’s resistance to shear or tensile stress. In other words, dynamic viscosity helps us understand how a fluid responds when force is applied to deform it. The SI unit used to quantify dynamic viscosity is the pascal-second (Pas). By calculating the dynamic viscosity of a fluid, we gain insight into its behavior under different conditions and can predict how it will respond to external forces.
Newton’s Equation
To quantify dynamic viscosity, we rely on Newton’s law of viscosity, a fundamental equation that describes the relationship between shear stress and shear rate in a Newtonian fluid. A Newtonian fluid is a fluid whose viscosity remains constant, regardless of the applied shear stress or shear rate. Mathematically, this relationship can be expressed as:
η = τ / du/dy
Here, η represents dynamic viscosity, τ is the shear stress applied to the fluid, and du/dy is the shear rate, which measures the change in velocity with respect to a change in distance. In essence, this equation helps us understand how a fluid’s viscosity responds to external forces.
b. Kinematic Viscosity
On the flip side, kinematic viscosity provides another perspective on a fluid’s behavior. It’s a measure of dynamic viscosity in relation to the fluid’s density. In other words, kinematic viscosity helps us understand how a fluid’s resistance to flow relates to its mass. The SI unit for kinematic viscosity is the stokes (St), but it can also be expressed in square meters per second (m²/s).
This measurement gives us valuable insights into the fluid’s internal behavior and how it interacts with its environment, taking into account its mass and dynamic viscosity. Understanding kinematic viscosity is key in various fields, particularly in the transportation industry, where it plays a significant role in the performance of fuels and lubricants.
6. Viscosity of Selected Materials
Now that we have covered the fundamental principles, we will now look at the viscosity of some selected materials. Therefore, we will examine a range of substances, from simple liquids to more complex materials, and observe how their viscosity changes with temperature.
- Water: Water serves as a reference point for understanding viscosity. At room temperature, water has a relatively low viscosity compared to more viscous liquids like honey. As we raise the temperature, water’s viscosity decreases, causing it to flow more easily.
- Honey: Honey, as mentioned, is significantly more viscous than water. Its high viscosity is due to the strong intermolecular forces between its molecules. Warming honey reduces these forces, making it less viscous.
- Motor Oil: Motor oil is a prime example of a fluid whose viscosity varies significantly with temperature. It is crucial for engine lubrication, and its viscosity must be tailored to the operating conditions. This is where the SAE numbering system for motor oils comes into play.
a. Viscosity of Water
Water viscosity refers to the fluid’s resistance to flow. This is caused by the frictional forces between the molecules of the liquid. It is a crucial characteristic of water. Therefore, we can say that it is relatively low when we compare it to other liquids. Therefore, liquids like honey, have higher viscosity values. This is because the molecules of water are relatively small. They also have weak intermolecular forces, which will make it easy for them to move past each other. At 200C, the viscosity of water is 0.01 Pascal second (Pas).
The viscosity of water can vary depending on several factors. These factors are temperature, pressure, and the presence of dissolved substances. Generally, as the temperature of water increases, its viscosity decreases, and the flow rate increases. Similarly, as the pressure increases, the viscosity of water also increases.
Furthermore, the presence of dissolved substances in water can also affect its thickness. For example, adding salt to water increases its viscosity, making it more resistant to flow. This is because the dissolved salt ions create more frictional forces between the water molecules. Thus, making it harder for them to move past each other.
b. Motor Oil Viscosity
Motor oil is a prime example of a fluid whose viscosity varies significantly with temperature. It is a critical component in the functioning of engines, where it serves to reduce friction and dissipate heat. Motor oil viscosity is not a fixed value; it changes with temperature and pressure. To help consumers select the right oil for their vehicles, the Society of Automotive Engineers (SAE) has established a numerical system for grading motor oil viscosity.
This system classifies motor oils based on their viscosity, taking into account their performance under different temperature conditions. When you see a label like “10W-40” on a bottle of motor oil, it represents the oil’s viscosity at both low and high temperatures. The “W” in the label stands for “winter,” indicating the oil’s low-temperature viscosity.
7. What is Viscosity Index
We can define the viscosity index as the measure of a fluid’s change in viscosity with variations in temperature. Additionally, viscosity Index, often abbreviated as VI, is a measure of how the viscosity of an oil changes with temperature. Specifically, it indicates how well an oil can maintain its viscosity as temperatures fluctuate. Oils with a high VI have relatively stable viscosities over a wide temperature range, making them suitable for varying operating conditions. In contrast, oils with a low VI experience significant viscosity changes with temperature, which can impact their effectiveness in lubrication. Viscosity Index is crucial in choosing the right oil for machinery and engines, ensuring they operate efficiently and with proper lubrication under different temperature conditions.
8. Viscosity Definition: Viscometer
Simply put, a viscometer is a device that can measure the viscosity of liquids. We can use a viscometer to measure the thickness of liquids in the medical field like syrups. We can also apply a viscometer to analyze the flow of milk and car lubricants. Additionally, we can use a viscometer to understand the thickness of ink during its production.
a. Capillary Viscometer
Understanding how to measure viscosity is very important in various scientific and industrial applications. One commonly used method for measuring viscosity is the capillary viscometer, which relies on the Hagen-Poiseuille equation.
The Hagen-Poiseuille equation relates the flow rate of a fluid through a cylindrical tube (such as a capillary) to the fluid’s properties and the pressure difference between the tube’s ends. This equation is particularly useful in calculating the viscosity of a fluid by measuring its flow rate through a capillary.
Hagen-Poiseuille equation describes the flow of a Newtonian fluid through a cylindrical tube, relating flow rate to the fluid’s properties and the pressure difference. It’s expressed as Q = (π * ΔP * r4) / (8 * η * L), where Q is the flow rate, ΔP is the pressure difference, r is the tube radius, η is dynamic viscosity, and L is the tube length.
9. Falling Sphere Method
Another method for determining a fluid’s viscosity is the falling sphere method. This technique involves dropping a sphere into the fluid and measuring its fall rate. The rate at which the sphere falls is directly related to the viscosity of the fluid it’s moving through. By analyzing the time it takes for the sphere to descend a specific distance, we can calculate the viscosity of the fluid.
10. Non-Newtonian Fluids
Up to this point, we’ve primarily discussed Newtonian fluids, where viscosity remains constant under varying shear stresses and shear rates. However, not all fluids follow this linear relationship between stress and rate of deformation. We now step into the fascinating world of non-Newtonian fluids, which exhibit diverse viscosity behaviors.
- Shear-Thinning: Some non-Newtonian fluids become less viscous as the shear rate increases. This means that, as you apply more force to them, they flow more readily. Ketchup is a classic example of a shear-thinning fluid. When you shake a ketchup bottle vigorously, the ketchup becomes less viscous and flows easily.
- Shear-Thickening: In contrast to shear-thinning fluids, shear-thickening fluids become more viscous as the shear rate increases. Silly Putty is a well-known example. When you quickly stretch or pull Silly Putty, it becomes firmer and exhibits higher viscosity.
- Pseudoplastic: Pseudoplastic materials display a decreasing viscosity with increasing shear rate. This behavior is typical of many polymer solutions and certain cosmetic products. Toothpaste, for instance, is a pseudoplastic fluid. It thickens in the tube but becomes less viscous when you apply shear by brushing your teeth.
- Dilatant: Dilatant fluids behave in the opposite manner of pseudoplastic ones. Their viscosity increases with higher shear rates. A classic example is a mixture of cornstarch and water. When you apply force to the mixture, it becomes thicker and more resistant to flow.
- Rheopectic: Rheopectic fluids become more viscous when subjected to prolonged shear. Unlike shear-thickening fluids, the increase in viscosity occurs over time rather than with a rapid change in shear rate. This property is found in materials like quicksand.
- Bingham Plastic: Bingham plastic materials have a threshold stress that must be exceeded before they start to flow. Below this threshold, they behave like a solid, but once the stress surpasses this point, they flow similarly to a Newtonian fluid. Mayonnaise is an example of a Bingham plastic.
11. Classification of Fluids According to Their Viscous Properties
Classification of fluids is based on high and low thickness depending on the frictional force:
- When frictional force is low: Examples of low viscous fluids are water, kerosene, petrol, and ethanol.
- When frictional force is large: Examples of highly viscous fluids are glue, syrup, grease, honey, blood, and glycerine.
12. Viscosity Definition: Effects
- It is responsible for the different rates of flow of fluids.
- It also affects the motion of the bodies in fluids.
13. Viscosity Definition: Application
- The viscous liquid is used as a lubricant
- The knowledge of viscous drag is used in the design of ships and aircraft.
- Particles of suspension in liquid fall with a steady terminal speed and this can be used to estimate the enlarged size of the particles.
- The effect of the viscosity of air on the bob of a swinging pendulum
14. Similarities Between Viscosity and Friction
- Both forces oppose relative motion between surfaces
- They all depend on the nature of the material in contact
15. Differences Between Viscosity and Friction
| Friction | Viscosity |
| Does not depend on areas of surfaces in contact | Depends on the areas of surfaces in contact |
| Depend on normal reaction | Does not Depend on normal reaction |
| Does not depend on the relative velocities between two layers | Depends on the relative velocities between two layers of fluid. |
| Occurs in solids. | Occurs in fluids (liquids and gases) |
16. Factors Affecting Viscosity
Now that we have seen the definitions and measurements of viscosity, let’s explore the factors that influence a fluid’s viscosity. Viscosity is not a static property; it can change depending on a variety of conditions. The main factors affecting viscosity are material, temperature, and pressure.
- Material: The nature of the fluid itself is a critical determinant of its viscosity. Different fluids, such as water, honey, and motor oil, exhibit varying levels of viscosity due to their unique molecular structures and intermolecular forces.
- Temperature Effects: Temperature has a significant impact on viscosity. As we increase the temperature of a fluid, its viscosity tends to decrease. This phenomenon can be observed in everyday life when, for instance, honey flows more easily when warmed.
- Pressure Effects: Pressure also influences the viscosity of a fluid. In certain cases, an increase in pressure can lead to an increase in viscosity. This effect is particularly important in understanding how fluids behave in deep-sea environments.
17. Viscoelasticity
Now, let us look at another intriguing aspect of fluid behavior: viscoelasticity. Some materials exhibit both viscous (fluid-like) and elastic (solid-like) properties, depending on the conditions to which they are subjected. This unique combination of properties defines viscoelastic behavior and is particularly important in understanding materials that exhibit both fluid and solid characteristics.
Viscoelastic materials can deform and flow like a fluid under stress, but they also return to their original shape when the stress is removed, similar to an elastic solid. This behavior is often found in materials such as rubber, human tissues, and even cheese. The viscoelasticity of cheese, for example, plays a vital role in its texture and the way it behaves when melted.
18. Rheology
To delve deeper into the study of the flow and deformation of matter, we encounter the field of rheology. Rheology is the science that focuses on understanding how materials flow, deform, and respond to stress. It is the discipline that helps us make sense of the diverse behaviors of materials like those mentioned above—Newtonian, non-Newtonian, viscoelastic, and everything in between.
Rheology provides scientists and engineers with the tools to predict and manipulate the flow and deformation of materials, allowing for advancements in fields as diverse as food processing, polymer manufacturing, and the design of transportation systems. In essence, rheology helps us comprehend how substances behave in response to forces and how we can harness this knowledge for practical applications.
19. Measurement Methods
To gain a comprehensive understanding of viscosity and rheology, scientists and engineers employ various measurement methods. Let’s briefly explore some of the common techniques used in the field.
- U-Tube Viscometer: The U-tube viscometer, also known as a glass capillary viscometer or Ostwald viscometer, is a widely used instrument for measuring viscosity. It consists of two reservoir bulbs and a capillary tube. One arm of the U-tube contains the capillary, a precise narrow bore that allows fluid to flow through. The upper bulb draws the liquid by suction, and the liquid flows down through the capillary into the lower bulb. By timing how long it takes for the liquid to pass between two marked points, we can determine the kinematic viscosity of the fluid.
The U-tube viscometer typically comes with a conversion factor that allows for accurate kinematic viscosity measurements. This method is particularly useful when precision measurements are required.
In real-world applications, viscosity measurements are crucial for various industries. They inform the design of products and systems, impacting fields such as chemical engineering, food production, pharmaceuticals, and more. The knowledge of viscosity and its measurement techniques empowers us to optimize processes and improve the performance of numerous products, from motor oils to foodstuffs.
You may also like to read:
Linear Expansivity: Definition and Calculations