1. What are Kinematic Equations in Physics?
In short, we can define kinematic equations in physics as a set of equations that describe the motion of objects, involving variables like distance, time, and velocity. Before we go deeper into the topic, we need to know the four basic kinematic equations which are as follows:
- First Kinematic Formula: v = v₀ + at
2. Second Kinematic Formula: Δx = (v + v₀)/2 * t
3. Third Kinematic Formula: Δx = v₀t + (1/2)at²
4. Fourth Kinematic Formula: v² = v₀² + 2aΔx
Where:
- Displacement = Δx
- Initial Velocity = v₀
- Final Velocity = v
- Time Interval = t
- Constant Acceleration = a
These equations express the relationships between the five key kinematic variables. Each equation serves a specific purpose and helps you calculate unknown quantities in a wide range of scenarios.
Kinematics allows us to describe how objects move and provides a foundation for understanding the principles governing motion.
a. The Purpose of Kinematic Equations
So, why do we need kinematic equations? These equations serve as a bridge between observations of motion and the mathematical representation of that motion. They allow us to express the relationships between different aspects of motion, helping us understand the “hows” and “whys” behind an object’s movement. Whether you’re tracking a rocket’s path through the sky or calculating the speed of a race car, kinematic equations provide the tools you need.
b. Equations of Motion
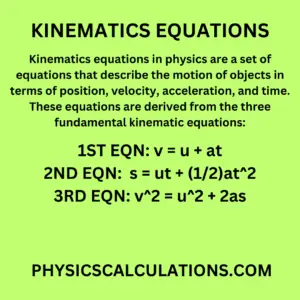
Additionally, kinematic equations of motion in physics are a set of equations that describe the motion of objects in terms of position, velocity, acceleration, and time. These equations are derived from the three fundamental kinematic equations:
First equation
v = u + at
Second equation
s = ut + (1/2)at2
Third equation
v2 = u2 + 2as
Fourth Equation
s = [ (u + v) / 2 ] x t
Where:
- v is the final velocity
- u is the initial velocity
- a is the acceleration
- s is the displacement
- t is the time
| First kinematic Equation | v = u + at |
| Second kinematic Equation | s = ut + (1/2)at2 |
| Third kinematic Equation | v2 = u2 + 2as |
The above equations are only valid for motion undergoing uniform acceleration. Hence, the acceleration remains constant throughout the motion. For non-uniformly accelerated motion, the equations of motion are more complex.
2. Explanation of Kinematic Equations
If you’ve ever played a sport, taken a car ride, or simply walked down the street, you’ve experienced motion. But have you ever stopped to consider the underlying principles of motion? Kinematics is an arm of physics that studies motion. On the other hand, kinematics equations are the mathematical formulas we use to describe and predict the behavior of moving objects.
At its most basic level, kinematics is the study of motion without considering the forces that cause it. Additionally, we also refer to kinematics equations as the equations of motion. These are mathematical formulas that describe the relationship between an object’s position, velocity, and acceleration over time. Therefore, these equations are fundamental to understanding and predicting the behavior of moving objects. In this article, we’ll explore the basics of kinematics equations, how they work, and why they’re important.
3. The Five Key Kinematic Variables
To understand kinematics equations, it’s important to first understand the concepts of displacement, velocity, and acceleration. Displacement is the change in an object’s position from its initial location to its final location. Velocity is the rate at which an object changes its position. Acceleration is the rate at which an object changes its velocity.
In kinematics, there are five key variables that play a pivotal role in understanding motion. Let’s delve into each of these variables to grasp their significance.
a. Displacement (Δx)
Displacement refers to the change in position of an object as it moves from one point to another. It provides essential information about how far an object has traveled and in which direction. Whether it’s a hiker on a trail or a bird in flight, understanding displacement helps us map out their journey.
b. Initial Velocity (v₀)
Initial velocity is the speed at which an object starts its motion. This value sets the stage for the entire journey. Whether you’re timing a sprinter’s start or calculating the launch speed of a rocket, initial velocity is a critical factor in kinematic equations.
c. Final Velocity (v)
As an object moves, it may accelerate or decelerate, ultimately reaching a different velocity. This final velocity tells us how fast the object is moving at a specific point in time. Think of a car coming to a stop or a parachutist reaching terminal velocity – their final velocities are vital in understanding their motion.
d. Time Interval (t)
The time interval represents the duration of the motion. In the world of kinematics, we frequently need to know how long an object has been in motion. Time is a fundamental variable, and its accurate measurement is key to solving kinematic problems.
e. Constant Acceleration (a)
Constant acceleration is a critical concept in kinematics. It denotes how an object’s velocity changes over time. Objects don’t always move at a consistent speed, and understanding how acceleration affects motion is central to kinematics.
4. How to Derive Kinematic Equations
The derivation of kinematics equations is based on the following assumptions:
- The object is moving in a straight line.
- The acceleration is constant.
- The velocity and acceleration are in the same direction.
Using these assumptions, we can derive the kinematic equations.
a. Deriving First Equation of Motion
We can derive the first equation by using the definition of acceleration, which is the rate of change of velocity with time. Therefore, we can now write:
Acceleration, a = change in velocity (v – u) / time (t)
Which will become
a = (v – u) / t [Remember that acceleration, a = change in velocity / time]
After rearranging the above equation and making v subject of the formula, we will obtain:
v = u + at [This is the first equation of motion]
b. Deriving the Second Equation of Motion
To obtain the second equation, we will apply the definition of displacement. Since we know that displacement is the change in the position of an object. Similarly, we can define displacement as a measure of the difference between two points in a specific direction. Consequently, the above statement will lead us to the equation below
Since average velocity, va = (u + v) / 2
Hence, the distance covered will become
s = [(u + v) / 2] t [Because v = s/t which shows that s = vt]
We can insert v = u + at into the above equation to obtain
s = [(u + v) / 2] t = [(u + u + at) / 2] t
Thus,
We will have s = [(2u + at)/2]t = ut + (1/2)at2
Therefore, our second equation of motion will be
s = ut + (1/2)at2
c. Deriving the Third Equation of Motion
The third equation is derived by using the first two equations and eliminating time. We can write:
v = u + at
Since s = ut + (1/2)at2
Hence,
By squaring both sides of v = u + at we will end up with
v2 = (u + at)(u + at) = u2 + 2uat + a2t2
Therefore,
v2 = u2 + 2a[ut + (1/2)at2]
and ut + (1/2)at2 is equal to s
Therefore, our third equation of motion will become
v2 = u2 + 2as
5. Applicability and Limitations of Kinematic Equations
Kinematics equations are used in various fields, including physics, engineering, and robotics. Some of the applications of kinematics equations are:
- Calculating the trajectory of a projectile: Kinematics equations can be used to calculate the path of a projectile, such as a cannonball or a rocket, under the influence of gravity.
- Designing roller coasters: Kinematics equations can be used to design roller coasters by calculating
While kinematic equations are powerful tools for analyzing motion, they are not a one-size-fits-all solution. There are specific situations in which kinematic equations are particularly useful, and others where they may not apply as neatly.
a. When to Use Kinematic Equations
Kinematic equations are your go-to when dealing with objects moving at a constant acceleration. These equations shine when you can confidently state that an object’s acceleration remains the same throughout its journey. In cases like free-fall, vehicles on the road, or any scenario with consistent acceleration, kinematic equations are your best friends.
b. Limitations of Kinematic Equations
Kinematic equations have their limits. They don’t work well when acceleration is not constant. If acceleration changes during an object’s journey, things get more complicated. You might need more advanced physics techniques to handle these situations. Also, when considering quantum or relativistic physics, kinematic equations are not the right tool for the job.
6. Inverse Kinematics
In physics and engineering, sometimes we find ourselves working backward – trying to figure out the initial conditions or the path of an object given its final state. This is where inverse kinematics comes into play.
a. Introduction to Inverse Kinematics
Inverse kinematics is a method used to solve for the input parameters that will result in a desired output. It’s especially important in robotics and animation. Imagine a robot arm – you want it to pick up an object at a specific location. Inverse kinematics helps you calculate the joint angles and movements needed to achieve that goal.
b. Complexity and Multiple Solutions in Inverse Kinematics
Inverse kinematics can be complex. In some cases, there might be multiple solutions, and determining which one to use is a challenge. Picture a human arm – there are multiple ways to reach for an object. Selecting the best solution depends on the context and objectives.
7. How to Use Kinematic Equations
The beauty of these equations lies in their simplicity. They allow you to calculate an unknown parameter as long as you know the other four. Let’s go through a quick example to illustrate how these equations work in practice.
8. Solved Problems Using Kinematic Equations
Here are some solved problems to help you understand how to apply kinematic equations (equations of motion):
Problem 1
A bus traveling at 60 km/h accelerates uniformly at 5 m/s2. Calculate its velocity after 2 minutes.
Answer
The final answer to this question is 617 m/s or 2221 km/h
Explanation
Data:
Initial velocity, u = 60 km/h = (60 x 1000) / 60 x 60 = 16.6 m/s = 17 m/s
Acceleration, a = 5 m/s2
Time, t = 2 min = 2 x 60 = 120 seconds
Unknown
Final velocity, v = ?
Formula
We will apply the first equation of motion v = u + at
Solution
We will insert our data into the formula
v = u + at = 17 + (5 x 120) = 617 m/s
We can convert the above answer into km/h as
v = (617 / 1000) x 60 x 60 = 2221.2 km/h = 2221 km/h
Problem 2
A car accelerates uniformly at a rate of 10 m/s2 from an initial velocity of 36 km/h for 30 seconds. Find the distance covered during this period.
Answer
The final answer to this question is 4.8 km
Explanation
Data
Initial velocity, u = 36 km/h = 10 m/s
Acceleration, a = 10 m/s2
Time, t = 30 seconds
Unknown
Distance covered, s = ?
Formula
We will use the second kinematic equation which says
s = ut + (1/2)at2
Solution
We will have
s = ut + (1/2)at2 = 10 x 30 + 0.5 x 10 x 302 = 300 + 4500 = 4800 m
We can convert the above answer (4800 meters) into km by saying
s = 4800 / 1000 = 4.8 km
Problem 3
A body moving with an initial velocity of 30 m/s accelerates uniformly at a rate of 10 m/s2 until it attains a velocity of 50 m/s. What is the distance covered during this period?
Answer
The final answer to this question is 80 meters
Explanation
Data
Initial velocity, u = 30 m/s
Acceleration, a = 10 m/s2
Final velocity, v = 50 m/s
Unknown
Distance covered, s = ?
Formula
From the third kinematic equation
v2 = u2 + 2as
and then make s subject of the formula to obtain the equation
s = (v2 – u2) / 2a
We will use the above equation to find the distance covered
Solution
By putting our data into the formula, we will have
s = (v2 – u2) / 2a = (502 – 302) / 2 x 10
Which implies that
s = 1600 / 20 = 80 m
Problem 4
Problem: A car accelerates from rest at a rate of 3 m/s² for 5 seconds. What is its final velocity?
Solution:
- Initial velocity (v₀) is 0 m/s (at rest).
- Acceleration (a) is 3 m/s².
- Time (t) is 5 seconds.
- We want to find final velocity (v).
Using the first kinematic equation (v = v₀ + at), we plug in the values: v = 0 m/s + (3 m/s² * 5 s) = 15 m/s
So, the car’s final velocity is 15 m/s.
9. Rotational Kinematics Equations
While we have been focusing on linear motion, it’s important to mention that kinematics also applies to rotational motion. Objects like wheels, gears, and planets undergo rotational motion, and they have their own set of rotational kinematics equations.
a. Introduction to Rotational Kinematics
Rotational kinematics is a branch of physics dealing with the motion of objects that rotate. In this context, we study variables like angular displacement, angular velocity, and angular acceleration.
b. Key Differences and Similarities
Linear and rotational kinematics have many similarities. Both use the concepts of displacement, velocity, and acceleration, but the way they’re calculated differs due to the circular nature of rotational motion. For example, the equations for rotational velocity and acceleration involve angular values (like radians) rather than linear measurements.
10. Frequently Asked Questions (FAQs)
What is the kinematics equation?
Kinematics equations are a set of equations that describe the motion of objects in terms of their position, velocity, and acceleration.
What are the three kinematics equations?
The three kinematic equations are:
1) v = u + at
2) s = ut + (1/2)at2
3) v2 = u2 + 2as
How do you solve kinematic equations?
To solve kinematic equations, you need to identify which equation(s) to use, plug in the known values, and solve for the unknown value(s) using algebra.
What is the kinematic formula for distance?
The kinematic formula for distance is s = ut + (1/2)at2, where s is distance, u is initial velocity, t is time, and a is acceleration.
What is the kinematic equation for velocity?
The kinematic equation for velocity is v = u + at, where v is final velocity, u is initial velocity, a is acceleration, and t is time.
What is the kinematic formula for acceleration?
The kinematic formula for acceleration is a = (v – u)/t, where a is acceleration, v is final velocity, vi is initial velocity, and t is time.
What is the difference between kinematics and dynamics?
Kinematics is the study of motion without considering the forces that cause the motion, while dynamics is the study of motion taking into account the forces that cause the motion.
What is kinematics used for?
Kinematics is used in many fields, including physics, engineering, and robotics, to describe and analyze the motion of objects and systems.
Test Your Understanding
Question: What is the kinematic equation for time?
Answer: There is no specific kinematic equation for time. Time is usually given in the kinematic equations as a known value that is used to solve for other variables such as distance, velocity, or acceleration.
- A car accelerates from 10 m/s to 30 m/s in 5 seconds. What is its acceleration? a. 2 m/s² b. 4 m/s² c. 6 m/s²
- Which of the following scenarios is best described using kinematic equations? a. A planet’s orbit around the sun b. A roller coaster ride with changing acceleration c. A swinging pendulum
Answers:
- b. 4 m/s²
- b. A roller coaster ride with changing acceleration
You may also like to read:
A body moves along a circular path
An object of weight 150 N moves with a speed of 4.5 m/s
Simple Harmonic Motion (SHM): Understanding the Basic Concepts
A car starts from rest and accelerates
Newton’s Second Law Practice Problems
A Cheetah is Crouched 20m to the East
Linear Expansivity: Definition and Calculations
A body moving at 20 m/s decelerates uniformly at 5m/s2