What is Maximum Height of a Projectile Motion?
Maximum Height Definition: The term “maximum height of a projectile motion” refers to the highest point reached by an object that is launched into the air and follows a curved path under the influence of gravity. It is the point where the object’s vertical velocity becomes zero before it begins descending back to the ground. When we throw a stone, we apply an external force on it and it will travel along a parabolic path. It will also undergo the influence of gravitational force, and air resistance before it finally falls down. This is what we called a projectile, and the path it follows is called a trajectory.

Understanding Maximum Height
When we throw an object vertically upward, it will accelerate vertically upward. The object will travel a certain distance (along the parabolic path) before it starts decelerating and finally falls to the ground. As the object is traveling along the parabolic paths, it follows a trajectory. We can describe the maximum height of an object as the highest vertical position attained by an object.
If you plot a graph to trace the motion and position of the object, you will observe that as soon as it reaches a certain height along the y-axis. The object will now start coming down to the ground. Initially, when we throw an object vertically upward, it will accelerate along the trajectory. The acceleration will start reducing until the body reaches a certain height where it can no longer move upward again. The body will then start coming back to the ground after reaching it is peak height which we call maximum height.
Maximum Height Formula
The symbol for maximum height is Hmax. The maximum height formula of an object undergoing projectile motion is:
Hmax = (U2Sin2θ) / 2g
Where
Hmax = Maximum height
U = Initial velocity
θ = Angle of projection
g = Gravitational acceleration = constant = 9.8 ms-2 or 10 ms-2
How to Derive the Formula for Maximum Height
We can apply two of the equations of motion to derive the formula for maximum height. The two equations are as follows:
s = ut + ½ at2 which is changed into H = ut – ½ gt2 due to the effect of gravitational force
or apply
v2 = u2 + 2as which is changed into v2 = u2 – 2gh due to the effect of gravitational force
and H = Hmax = maximum height
First Method of Deriving Maximum Height
If u is the initial velocity along the vertical component to reach the maximum height
Let us apply the formula v2 = u2 + 2as
when we substitute v = 0, a = -g and s = Hmax into the above equation, we now have
0 = u2 – 2gHmax
we now make take 2gHmax to the side of the zero to obtain
2gHmax = u2
By making the Hmax the subject of the formula, we now have
Hmax = u2 / 2g which is the formula for maximum height
For an angle θ
We have
Hmax = (u2sin2θ) / 2g where u = uy = usinθ
Second Method of Deriving Maximum Height
Let us apply the second formula s = ut + ½ at2 to derive the maximum height
when we change s = Hmax, a = -g (due to gravitational force). We will now obtain
Hmax = ut – ½ gt2
Assuming u = ux = usinθ
since t = (usinθ) / g
we now have
Hmax = usinθ[(usinθ) / g] – ½ g[(usinθ) / g]2
Hmax = [u2sin2θ / g] – [(u2sin2θ) / 2g]
The above equation can further be written as
Hmax = [u2sin2θ / g] [1 – ½ ]
The above equation will now become
Hmax = [u2sin2θ / g] [½ ]
Therefore, we can finally write our maximum height as
Hmax = (u2sin2θ) / 2g
Problems on How to Find Maximum Height
Here are solved problems to help you master how to find maximum height:
Problem 1
A ball is projected at an angle of elevation of 600 with an initial velocity of 100ms-1. Find the maximum height reached.

Solution
Data:
Initial velocity, u = 100ms-1
The angle of projection, θ = 600
gravitational acceleration is constant, and the symbol is g = 10ms-2
The formula for maximum height, Hmax = (u2sin2θ) / 2g
and we can substitute our data into the above formula as
Hmax = (1002sin2600) / (2 x 10)
This implies
Hmax = (10,000 x sin2600) / (2 x 10) = (10,000 x 0.75) / 20 = 7,500/ 20 = 375m
Problem 2
A bullet is fired at an angle of 450 to the horizontal with a velocity of 490 m/s. Calculate the maximum height reached by the bullet.

Solution
Data:
The angle of projection, θ = 450
Initial velocity, u = 490 m/s
gravitational acceleration is constant, and the symbol is g = 10ms-2
And the formula for maximum height, Hmax = (u2sin2θ) / 2g
we apply our data into the above formula
Hmax = (4902 x sin2450) / 2 x 10 = (240,100 x 0.5) / 20 = 120,050/20 = 6002.5 m = 6 km
Problem 3
A stone is shot out from a catapult with an initial velocity of 30 m/s at an elevation of 600. Find the maximum height attained.
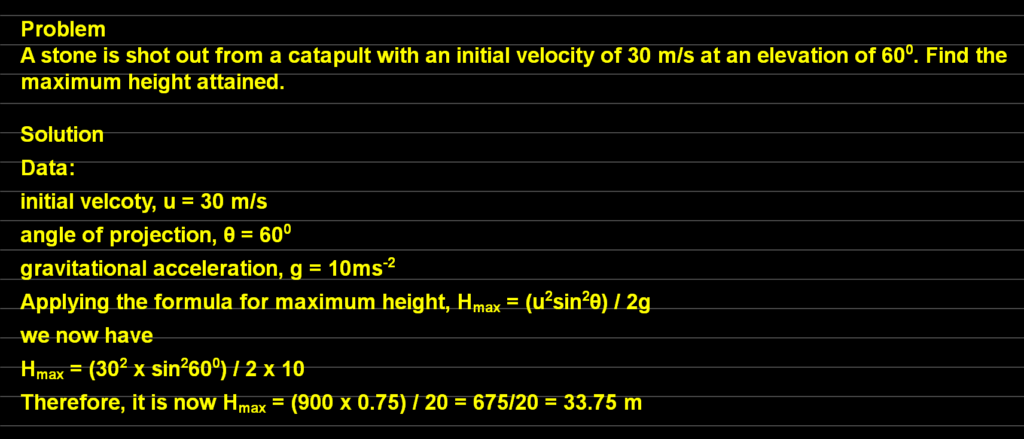
Solution
Data:
initial velcoty, u = 30 m/s
angle of projection, θ = 600
gravitational acceleration, g = 10ms-2
Applying the formula for maximum height, Hmax = (u2sin2θ) / 2g
we now have
Hmax = (302 x sin2600) / 2 x 10
Therefore, it is now Hmax = (900 x 0.75) / 20 = 675/20 = 33.75 m
Problem 4
A tennis ball is thrown vertically upwards from the front with a velocity of 50 m/s. Calculate the maximum height reached.

Solution
Data:
Initial velocity, u = 50m/s
gravitational acceleration, g = 10ms-2
Also, Hmax = u2 / 2g = 502 / 2 x 10 = 2500 / 20 = 125m
Problem 5
A bullet is fired from a point making an angel of 300 to the horizontal, H. The initial velocity of the bullet is 40 m/s. Calculate the greatest height reached.
Solution
Data:
Angle of the bullet = 300
u = 40 m/s
g = 10 ms-2
Hmax = (u2sin2θ) / 2g
Hmax = (402 x sin2300) / 2 x 10 = (1,600 x 0.25) / 20 = 400/20 = 20 m
Problem 6
A stone is projected upwards at an angle of 300 to the horizontal from the top of a tower of height 100m and it hits the ground at a point Q. If the initial velocity of projection is 100m/s, calculate the maximum height of the stone above the ground.
Data: The information from the question
Angle of projection, θ = 300
The maximum height of the stone above the ground, S, is equal to the maximum height, H from the point of projection and the height of the tower, 100m. Thus, S = 100 + H
Initial velocity, u = 100 m/s
We will now apply the formula: Hmax = (u2sin2θ) / 2g
Solution
We will now substitute our formula with our data to obtain
Hmax = (u2sin2θ) / 2g = (1002 x sin2300) / (2 x 10) = 125m
However, we have S = 100 + Hmax = 100 + 125 = 225 m
Therefore, the maximum height of the stone above the ground is 225 meters.
You may also like to read:
How to Calculate Cubic Expansivity with Examples
Reference