Concept of Gas Laws in Physics
Gas laws are a set of mathematical relationships that describe the behaviour of gases under various conditions. They provide a framework for understanding how gases respond to changes in temperature, pressure, volume, and the number of gas molecules. Gas is a state of matter that permeates our everyday lives. From the air we breathe to the fuel that powers our vehicles
2. The Basics of Gas Behavior
Gases consist of tiny particles, such as atoms or molecules, that are in constant motion and exhibit negligible intermolecular forces. These particles move randomly and collide with each other and the walls of their container, and thereafter exerting pressure on the walls of the container.
We can describe the behaviour of gases by using variables such as pressure (P), volume (V), temperature (T), and the number of moles (n) of gas molecules present. Gas laws provide relationships between these variables, which allow us to make predictions and understand how changes in one variable affect the other.
Gas Laws Formula
Here is a table of gas laws formulas along with their inventors, dates of invention, formulas, units, and respective dimensions based on the information available.
| Gas Law | Inventor | Date of Invention | Formula | Unit | Dimensions |
|---|---|---|---|---|---|
| Boyle’s Law | Robert Boyle | 1662 | PV = constant | P * V | Pressure * Volume |
| Charles’s Law | Jacques Charles | 1787 | V / T = constant | V / T | Volume / Temperature |
| Gay-Lussac’s Law | Joseph Gay-Lussac | 1802 | P / T = constant | P / T | Pressure / Temperature |
| Avogadro’s Law | Amedeo Avogadro | 1811 | V / n = constant | V / n | Volume / Amount of substance |
| Ideal Gas Law | Emil Clapeyron | 1834 (further developed by others later) | PV / nT = constant | P * V / (n * T) | Pressure * Volume / (Amount of substance * Temperature) |
| Combined Gas Law | (P1 * V1) / (T1 * n1) = (P2 * V2) / (T2 * n2) | Varies | Varies |
Properties of Gases
Gas is one of the states of matter. It is mostly obtained when a solid is heated to its melting point. Thereafter, its forces of attraction become weaker and will now break into liquid. When we add more heat to raise the temperature of the liquid to its boiling point, the liquid will start vaporizing and give up in the form of gases. Here are some of properties of gases you will need to know:
- The molecules possess a weak cohesive force
- The molecules of gases are negligible.
- Molecules of gases fill the entire container in which they are.
- They have the highest kinetic energy compared to solid and liquid
- The degree of randomness in the molecules of gases is high.
Kinetic Theory of Gases
According to the kinetic theory of gases, the following also apply:
- Gases are tiny particles that move in a straight line and collide with one another and also with the wall of the container.
- The collision between the gases is perfectly elastic.
- The cohesive force of the gases is also negligible
- The temperature of the gas is the measure of the average kinetic energy of the gas.
3. Boyle’s Law
Boyle’s Law, named after a physicist Robert Boyle, states that the pressure of a gas is inversely proportional to its volume when the temperature and the number of gas molecules are constant. Mathematically, it can be expressed as: V ∝ (1/P)
Therefore, Boyle’s Law Formula is:
P1V1 = P2V2
where P1 and V1 represent the initial pressure and volume, and P2 and V2 represent the final pressure and volume, respectively.
This law explains why squeezing a gas-filled balloon reduces its volume, causing an increase in pressure.
4. Charles’ Law
Charles’ Law, formulated by physicist Jacques Charles, describes the relationship between the temperature and volume of a gas when the pressure and the number of gas molecules are constant. According to Charles’s Law, as the temperature of a gas increases, its volume also increases, and vice versa.
Therefore, Charles’ Law states that the volume of a fixed gas is directly proportional to its absolute temperature, provided pressure is kept constant.
Mathematically, the charles’ law formula can be stated as: V ∝ T
Therefore, Charles’s Law Equation:
V1/T1 = V2/T2
Where V1 and T1 represent the initial volume and temperature, and V2 and T2 represent the final volume and temperature, respectively. As we can see, temperature and volume are involved in the case of Charles’ Law.
This law explains why a helium-filled balloon expands when exposed to heat.
5. Gay-Lussac’s Law
Gay-Lussac’s Law, named after chemist Joseph Louis Gay-Lussac, relates the pressure of a gas to its temperature when the volume and the number of gas molecules remain constant. According to this law, as the temperature of a gas increases, its pressure also increases, and vice versa. Mathematically, it can be expressed as:
Gay-Lussac’s Law Equation:
P1/T1 = P2/T2
where P1 and T1 represent the initial pressure and temperature, and P2 and T2 represent the final pressure and temperature, respectively.
This law helps in explaining why the pressure inside a car tire increases on a hot day.
6. The Combined Gas Law
The Combined Gas Law combines Boyle’s, Charles’s, and Gay-Lussac’s Laws into a single equation that describes the relationship between a gas pressure, volume, and a temperature. Thus, it allows us to calculate the changes in these properties when more than one variable is altered. The equation can be written as:
(P1V1)/T1 = (P2V2)/T2
The Combined Gas Law is particularly useful when studying gases undergoing complex changes.
7. Avogadro’s Law
Avogadro’s Law, proposed by the Italian scientist Amedeo Avogadro, states that equal volumes of gases, at the same temperature and pressure, contain an equal number of particles. This law helps us to understand the relationship between the volume and the amount (in moles) of gas. Mathematically, it can be expressed as:
Avogadro’s Law Equation:
V1/n1 = V2/n2
where V1 and n1 represent the initial volume and amount of gas, and V2 and n2 represent the final volume and amount of gas, respectively.
Avogadro’s Law is particularly relevant in stoichiometry calculations and understanding gas reactions.
8. Ideal Gas Law
The Ideal Gas Law combines all the previously discussed gas laws (Boyle’s, Charles’s, Gay-Lussac’s, and Avogadro’s Laws) into a single equation that describes the behavior of an ideal gas. The Ideal Gas Law equation is given as:
PV = nRT
where (P) represents pressure, (V) represents volume, (n) represents the amount of gas (in moles), (R) represents the ideal gas constant, and (T) represents temperature in Kelvin.
The Ideal Gas Law allows us to relate the macroscopic properties of a gas to its microscopic behaviour.
9. Dalton’s Law of Partial Pressures
Dalton’s Law of Partial Pressures states that the total pressure exerted by a mixture of non-reacting gases is equal to the sum of the partial pressures of each individual gas. Mathematically, it can be expressed as:
Dalton’s Law Equation:
Ptotal = P1 + P2 + P3 + ….
where Ptotal represents the total pressure of the gas mixture, and (P1 + P2 + P3 ) represents the partial pressures of each gas component.
Dalton’s Law is very important when it comes to dealing with gas mixtures, such as the composition of the Earth’s atmosphere.
10. Graham’s Law of Effusion and Diffusion
Graham’s Law of Effusion and Diffusion describes the rate at which gases effuse (escape through a small opening) or diffuse (mix with other gases).
According to Graham’s Law, the rate of effusion or diffusion of a gas is inversely proportional to the square root of Therefore, understanding these deviations is very important when studying gases in extreme conditions or when high precision is required.
12. Applications of Gas Laws in Everyday Life
Gas laws find practical applications in various fields and aspects of everyday life. Some notable examples include:
- Understanding and predicting weather phenomena, such as changes in atmospheric pressure and temperature.
- Designing and operating scuba diving equipment by considering gas solubility and pressure changes at different depths.
- Developing efficient fuel combustion strategies in engines by optimizing air-fuel ratios.
- Analyzing and interpreting gas data in industrial processes, such as chemical reactions and gas storage.
13. General Gas Law Formula
The general gas law in physics is the combination of Boyle’s, Charle’s, and Pressure laws for an ideal gas.
We can write the formula as
PV / T = Constant
Where
P = pressure
V = volume
T = Temperature
And because of the constant, we can now write the formula for the general gas law as
P1V1 / T1 = P2V2 / T2
Similarly, the Ideal Gas Law is written as PV = nRT
14. General Gas Law in Physics: Solved Problems
Here are a few examples to help you understand how to apply the formula for general gas law and calculate a problem.
Problem 1: General Gas Law
A mass of gas at 170c and 700mmHg has a volume of 1.2m3. Determine its volume at 270c and pressure at 750 mmHg.
Answer
Solution: To calculate the volume from general gas law, we need to first extract our data from the question

Data:
Initial Pressure = P1 = 700mmHg
Final Pressure = P2 = 750mmHg
Initial Temperature = T1 = 170c = 273 + 17 = 290
Final Temperature = T2 = 270c = 273 + 27 = 300
Additionally, initial Volume = V1 = 1.2m3
Also, Final Volume = V2 = ?
Using the general gas law,
( P1 V1) / ( T1 )= ( P2 V2 ) / ( T2 )
By Making V2 subject of the formula,we have
Thus, V2 = ( P1 V1T2) / (P2 T1 )
by substituting the above expression with our data, we have:
Final volume, V2 = ( 700 x 1.2 x 300 ) / ( 750 x 290 )
Therefore, V2 = ( 252,000 ) / ( 217,500 )
This will give us our final answer as V2 = 1.2 m3
Therefore, the final volume is 1.2 m3
Problem 2: General Gas Law
The volume and pressure of a given mass of gas at 270C are 76 cm3 and 80 cm of mercury respectively. Calculate its volume at standard temperature and pressure (s.t.p)
Answer
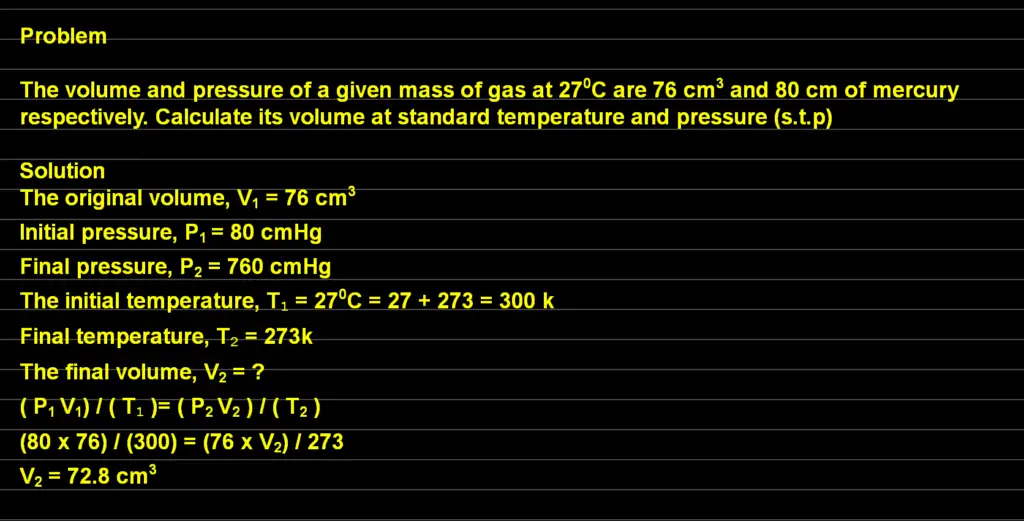
Data
The original volume, V1 = 76 cm3
Initial pressure, P1 = 80 cmHg
Final pressure, P2 = 760 cmHg [Because of the standard temperature and pressure]
The initial temperature, T1 = 270C = 27 + 273 = 300 k
Final temperature, T2 = 273k [Because of the standard temperature and pressure]
Unknown
The final volume, V2 = ?
Formula
We will now apply our formula to solve the problem
( P1 V1) / ( T1 )= ( P2 V2 ) / ( T2 )
after inserting our data, we will now have
(80 x 76) / (300) = (76 x V2) / 273
By making V2 subject of the formula, we will obtain our answer as
V2 = 72.8 cm3
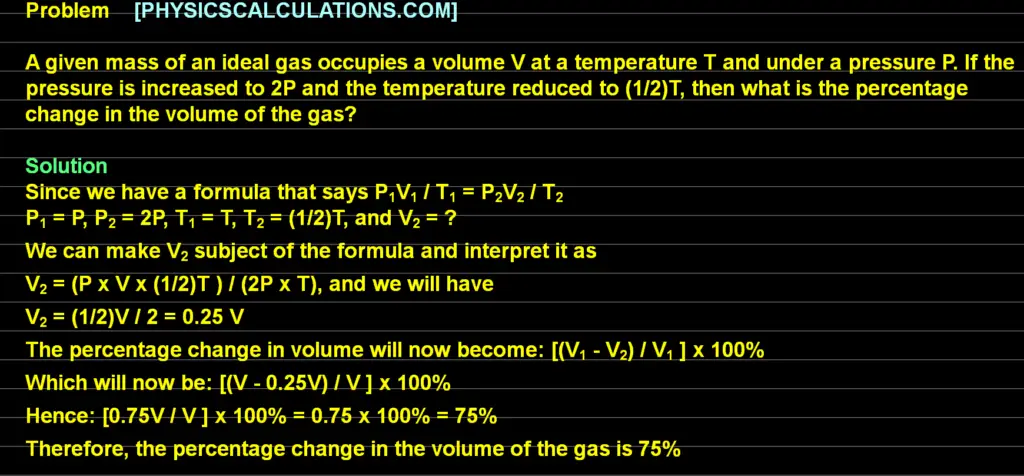
Problem 3: General Gas Law
A given mass of an ideal gas occupies a volume V at a temperature T and under a pressure P. If the pressure is increased to 2P and the temperature reduced to (1/2)T, then what is the percentage change in the volume of the gas?
Answer
The final answer to the above question is 75%
Explanation
Since we have a formula that says P1V1 / T1 = P2V2 / T2
and
V1 = V
P1 = P
P2 = 2P
T1 = T
T2 = (1/2)T
V2 = ?
We can make V2 subject of the formula and interpret it as
V2 = (P x V x (1/2)T ) / (2P x T)
And we will have
V2 = (1/2)V / 2 = 0.25 V
The percentage change in volume will now become
[(V1 – V2) / V1 ] x 100%
Which will now be
[(V – 0.25V) / V ] x 100%
hence
[0.75V / V ] x 100% = 0.75 x 100% = 75%
Therefore, the percentage change in the volume of the gas is 75%
Problem 4: General Gas Law
The pressure of a given mass of a gas changes from 300 Nm-2 to 120 Nm-2 while the temperature drops from 1270C to -730C. What is the ratio of the final volume to the initial volume?
Answer
The final answer to the question is V1 : V2 = 5 : 4

Explanation
P1 = 300 Nm-2
and P2 = 120 Nm-2
T1 = 127 + 273 = 400 K
we have T2 = -73 + 273 = 200 K
Formula
The formula we will use is P1V1 / T1 = P2V2 / T2
Solution
We will now insert our data into the formula
(300 x V1) / 400 = (120 x V2) / 200
To have a ratio of V1 to V2
Hence,
V2 : V1 = 60000 / 48000
This implies:
60 / 48 = V2 / V1
When we divide both 60 and 48 by 12, we will obtain
V2 / V1 = 5 / 4
Therefore, the ratio of V2 to V1 is 5 to 4
Problem 5: Boyle’s Law
The volume of a fixed mass of a gas is 200 cm3 at 270C. Calculate its temperature when the volume is reduced to (1/2) its original at the same pressure.
Answer
The final answer to the above question is -1230C

Explanation
Data:
V1 = 200 cm3
T1 = 273 + 27 = 300K
V2 = (1/2)V1 = 0.5 x 200 = 100 cm3
Unknown:
T2 = ?
Formula:
V1 / T1 = V2 / T2
Solution
Applying our data into the formula, we will have:
200 / 300 = 100 / T2
By making T2 subject of the formula, we will have
T2 = (300 x 100) / 200 = 150 K
Hence, T2 = 273 K + t
t = 150 – 273 = -1230C
Therefore, the temperature is -1230C
Problem 6: Boyle’s Law
If the volume of a fixed mass of hydrogen in a container is 30 cm3 at a pressure of 50 mmHg. Find the volume of the gas if the pressure is 60 mmHg at constant pressure.
Answer
The final answer to the question is 25 cm3

Explanation:
We need to follow the following steps to solve the problem
First step (Data): The available information from the question
Initial volume, V1 = 30 cm3
Initial pressure, P1 = 50 mmHg
Final Pressure, P2 = 60 mmHg
Unknown: What we need to find
Final volume, V2 = ?
Formula: The equation that will help us to solve the problem
We will apply Boyle’s law, P1V1 = P2V2
Since we are to find the final volume, we will now make V2 subject of the formula
V2 = (P1V1) / P2
Therefore, we will use the above equation to solve the problem
Solution:
We will start by substituting our formula with available data from the question
V2 = (P1V1) / P2 = (50 x 30) / 60 = 25 cm3
Problem 7: Boyle’s Law
If the final volume of a gas is one-fifth of the initial volume. Find the ratio of the final pressure to the initial pressure at a constant temperature.
Answer
Data: Available information from the question
Final volume, V2 = (1/5)V1
Formula:
We will use Boyle’s law, P1V1 = P2V2
Solution
Changing our formula with the values in our data, we will obtain:
P1V1 = P2 x [(1/5)V1]
We can clearly see that V1 will cancel each other, leaving us with
5P1 = P2
Therefore, to find the ratio of the final to the initial pressure. We divide both sides by P1 to obtain:
P2 / P1 = 5
Thus, we can equally write the above question as
(P2 / P1) = (5 / 1)
Therefore, the ratio is
P2 : P1 = 5 : 1
Thus, the ratio of the final pressure to the initial is 5 to 1
Problem 8: Charles’ Law
The volume of a fixed mass of a gas is 200 cm3 at 270C. Calculate its temperature when the volume is reduced 1/2 its original at the same pressure.
Answer
The final answer to the question is -1230C
Explanation:
Data: The information available from the question
Initial volume, V1 = 200 cm3
Initial temperature, T1 = 270C = 27 + 273 = 300 K
Final volume, V2 = (1/2) V1 = 0.5 x 200 cm3 = 100 cm3
Unknown: What we need to find
Temperature = ?
Formula: The equation to solve the problem
V1 / T1 = V2 / T2
Solution:
Making T2 subject of the formula, and then substituting our data into the formula, we will have
T2 = (V2T1) / V1 = (100 x 300) / 200 = 150 K
Thus, since T2 = 273 + t
We will have:
150 = 273 + t
t = 150 – 273 = -1230C
Problem 9: Gay-Lussac’s Law
A fixed mass of air at standard temperature pressure is heated such that its volume remains unchanged. Calculate its tmeperature when its pressure is increased to 114 cm of mercury (Hg).
Answer
The final answer to the question is 136.50C
Explanation:
We will commence by following these important steps below:
Data: The information available from the question
Initial temperature, T1 = 00C = 273 K
The initial pressure, P1 = 76 cm Hg
Final Pressure, P2 = 114 cm Hg
Unknown: What we need to find
Final temperature, T2 = ?
Formula: The equation that will help us solve the problem
P1 / T1 = P2 / T2
Solution:
We will now make T2 subject of the formula and then insert our data into the formula
T2 = (P2T1) / P1 = (114 x 273) / 76 = 409.5
However, T = 273 + t
Therefore,
409.5 = 273 + t
Which implies that
t = 409.5 – 273 = 136.50C
Problem 10: Gay-Lussac’s Law
A mass of gas at a pressure of 50 mmHg is heated from 270C to 970C. If the volume is maintained at a constant. Calculate the pressure exterted by the gas.
Answer
The final answer to the above question is 61.67 mmHg
Explanation:
Data:
Initial pressure, P1 = 50 mmHg
The initial temperature, T1 = 270C = 27 + 273 = 300 K
Final temperature, T2 = 970C = 97 + 273 = 370 K
Unknown:
Final pressure, P2 = ?
Formula:
P1 / T1 = P2 / T2
Solution:
We will make P2 subject of the formula and then insert our data into the formula
P2 = P1T2 / T1 = (50 x 370) / 300 = 61.67 mmHg
Therefore, the pressure exterted by the gas is 61.67 mmHg
15. Measurement of Gas Pressure
U-tube manometer which contains water is used in measuring gas pressure. One end of the tube is connected to the gas supply, while the other end is opened to the atmospheric pressure. Therefore, when gas is supplied, pressure from the gas causes the water level to rise to a height h, on the other side of the tube. Thus, pressure at A and h are equal since are at the same horizontal level.
Pressure at A = Atmospheric pressure = pressure due to the volume of water at height h.
Also, the Pressure of gas = Atmospheric pressure + ρgh
Where
ρ = density
g = Acceleration due to gravity
h = height
Note: In 1643, an Italian scientist named Torricelli invented an instrument called a barometer for measuring the pressure of the air.
16. Summary
Summarily, we have seen that general gas laws form the backbone of our understanding of gas behaviour. Boyle’s Law, Charles’s Law, Gay-Lussac’s Law, Combined Gas Law, Avogadro’s Law, the Ideal Gas Law, Dalton’s Law, and Graham’s Law provide valuable insights into the properties and relationships of gases.
By applying these laws, we can make accurate predictions and solve real-world problems across various disciplines related to gas.
17. Frequently Asked Questions
Q1: What are the units of pressure in gas laws?
A1: Pressure in gas laws is typically measured in units such as Pascals (Pa), atmospheres (atm), or millimetres of mercury (mmHg).
Q2: How do gas laws relate to weather phenomena?
A2: Gas laws, such as changes in pressure and temperature, helps us in understanding and predicting weather phenomena like air masses, fronts, and atmospheric stability.
Q3: Can you provide an example of a real gas?
A3:Yes, gases such as carbon dioxide (CO2) and water vapor (H2O) display real gas behavior under certain conditions, deviating from the ideal gas model.
Q4: What happens to gas molecules at absolute zero?
A4: At absolute zero (-273.15°C or 0 Kelvin), gas molecules theoretically cease all motion and exhibit no pressure or volume.
You may also like to read:
How to Calculate Cubic Expansivity with Examples
Linear Expansivity: Definition and Calculations
Density of Water at 20 Degrees Celsius
Also How to Calculate Specific Heat Capacity
And How to Calculate Relative Humidity Using a Formula
How to Calculate Cubic Expansivity with Examples
You can also check our website
Wokminer and search for any Job in any location
Reference
Tags
temperature and pressure, amount of gas, gas is directly proportional, gay lussacs, ideal gas law, remain constant, ideal gases, law of partial pressures, straight line, volume of the gas, kinetic theory, gas constant