Energy in Simple Harmonic Motion Equations
Below is a video explanation of the energy of simple harmonic motion equations
| You may also like to read: |
| Simple Harmonic Motion Formulae |
What is Energy in Simple Harmonic Motion?
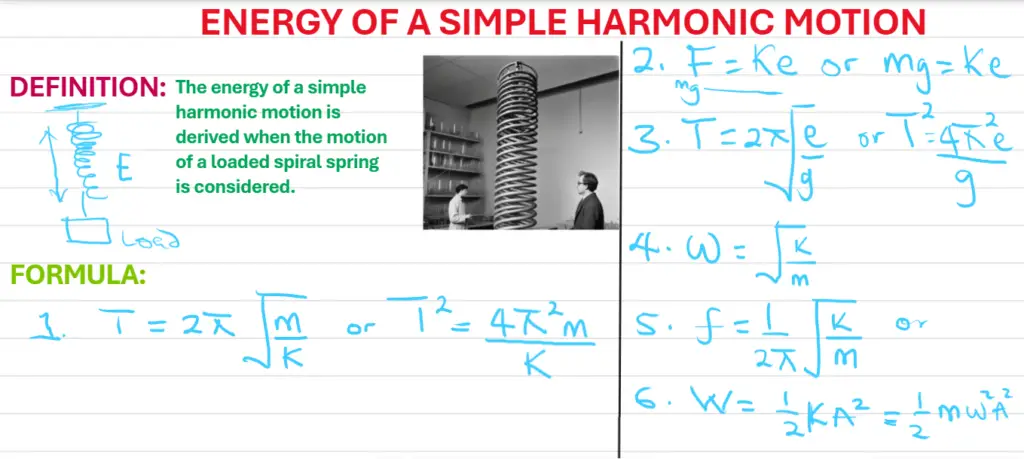
The energy in a simple harmonic motion is derived when the motion of a loaded spiral spring is considered. The following equations can be applied to solving problems involving loaded spiral spring and energy of simple harmonic motion:
- T = 2π √(m/K) or T2 = (4π2 m)/K
- F = Ke or mg = Ke
- T = 2π √(e/g) or T2 = (4π2 e)/g
- ω = √(K/m)
- f = (1/2π) √(K/m) or f2 = K/(4π2 m)
- W = (1/2) KA2 or W = (1/2)mω2A2
Where:
T = temperature
m = mass of body in kilograms
e = extension of spring in meters
g = acceleration due to gravity in meters per second
F = force, load or weight in Newton (N)
ω = angular speed or velocity in meter per second (m/s)
f = frequency of motion in s or Hz
W = total work done by spring or energy stored in spring and is measured in joule (J)
A = amplitude of motion (m)
| You may also like to read: |
| What is Simple Harmonic Motion in Physics |
| Simple Pendulum: Definition, Formula, and Calculations |
| what is the system’s potential energy when its kinetic energy is equal to 34e? |
Solved Problem
A body of mass 500g suspended from the end of the spiral spring which obeys Hooke’s law, produced an extension of 10 cm. If the mass is pulled down a distance of 5cm and released, calculate:
a. The force constant of the spring
b. The frequency of oscillation
c. The period of oscillation
d. The angular speed of the body
Solution
Mass (m) = 500 g = 0.5 kg, and extension (e) = 10 cm = 0.1 m
a. The force constant (K) of the spring is:
From Hooke’s law, F = Ke
Thus, K = F/e = mg/e = (0.5 x 10) / 0.1 = 50 N/m
b. The frequency of oscillation (f) is:
f = (1/2π) √(K/m) = (1/2π) √(50/0.5) = 1.59 s-1
c. The period of oscillation (T) is:
T = 1/f = 1/1.59 = 0.628 s
d. The angular speed (ω) of the body is:
ω = √(K/m) = √(50/0.5) = 10 rad/s
Important Points to Note:
What is Simple Harmonic Motion?
Simple Harmonic Motion (SHM) refers to the repetitive oscillatory motion exhibited by a system when the restoring force is directly proportional to the displacement from the equilibrium position and acts in the opposite direction to that displacement. This unique characteristic results in smooth, back-and-forth movements that are mathematically describable using sinusoidal functions.
In the context of energy, SHM involves the conversion of potential energy to kinetic energy and vice versa as the object oscillates. The energy exchange in SHM is a vital aspect that governs the dynamics of the system.
You may also like to read:
Understanding Potential Energy in SHM
In simple harmonic motion, potential energy is very important. When an oscillating object is at its maximum displacement from the equilibrium position, it possesses the highest potential energy. Thus, as the object moves towards the equilibrium position, its potential energy decreases, reaching a minimum when it passes through the center.
The potential energy in SHM can be mathematically represented as follows:
PE = (1/2)Kx2
Where:
PE = Potential Energy
K = Spring constant (a measure of the stiffness of the restoring force)
x = Displacement from the equilibrium position
Calculating Kinetic Energy in SHM
Conversely, kinetic energy is at its peak when the object is at the equilibrium position, and its velocity is at its maximum. As the object moves away from the center, its kinetic energy decreases, reaching zero at the maximum displacement.
The kinetic energy in SHM can be mathematically represented as:
KE = (1/2)mv2
Where:
KE = Kinetic Energy
m = Mass of the oscillating object
v = Velocity of the object
The Total Mechanical Energy of SHM
In a closed system, the total mechanical energy remains constant throughout the oscillations. As the object moves between its extreme points, it continually converts potential energy into kinetic energy and back, without any losses due to friction or other dissipative forces.
The total mechanical energy (E) in SHM is the sum of the potential energy (PE) and kinetic energy (KE):
E = PE + KE
Therefore, E = (1/2)Kx2 + (1/2)mv2
The conservation of mechanical energy is a fundamental principle that underlies the perpetuity of oscillatory motion in simple harmonic systems.
Applications of SHM and Its Energy Equations
There are different applications of simple harmonic motion and its energy equations in various fields, including:
- Engineering: In mechanical engineering, SHM helps us in designing and analyzing vibrating systems like bridges, buildings, and machines.
- Music and Sound: SHM is the basis of musical notes produced by vibrating strings and air columns in musical instruments.
- Electronics: In electronics, SHM is utilized in the functioning of oscillators and quartz crystals used in watches and electronic circuits.
- Astronomy: Celestial bodies often undergo SHM-like motions, such as the orbits of planets around the sun or moons around planets.
- Seismology: Studying the oscillations of the Earth after seismic events provides valuable insights into the structure of our planet.
- Harmonics Analysis: Analyzing the energy distribution in SHM helps identify and control resonant frequencies in complex systems.
- Medical Applications: We also use the SHM principles in medical devices, such as ultrasound machines and artificial pacemakers.
FAQs
- Q: What is the significance of simple harmonic motion equations in real-world applications?
A: Simple harmonic motion equations are fundamental in explaining and predicting oscillatory behaviour in various natural and engineered systems, making them valuable in fields like engineering, music, and astronomy. - Q: Can the total mechanical energy change in simple harmonic motion?
A: No, the total mechanical energy in SHM remains constant in a closed system, as there are no energy losses due to external forces. - Q: How is potential energy related to displacement in SHM?
A: The potential energy in SHM is directly proportional to the square of the displacement from the equilibrium position. - Q: Is the concept of SHM limited to linear systems?
A: No, while the equations presented here are for linear SHM, the concept can be extended to non-linear systems as well. - Q: How does damping affect the energy exchange in SHM?
A: Damping, which introduces energy dissipation, gradually reduces the amplitude of oscillations over time. - Q: Are there real-world examples of SHM in our daily lives?
A: Yes, examples include the swinging of a pendulum, the vibrations of guitar strings, and the motion of a child on a swing.
