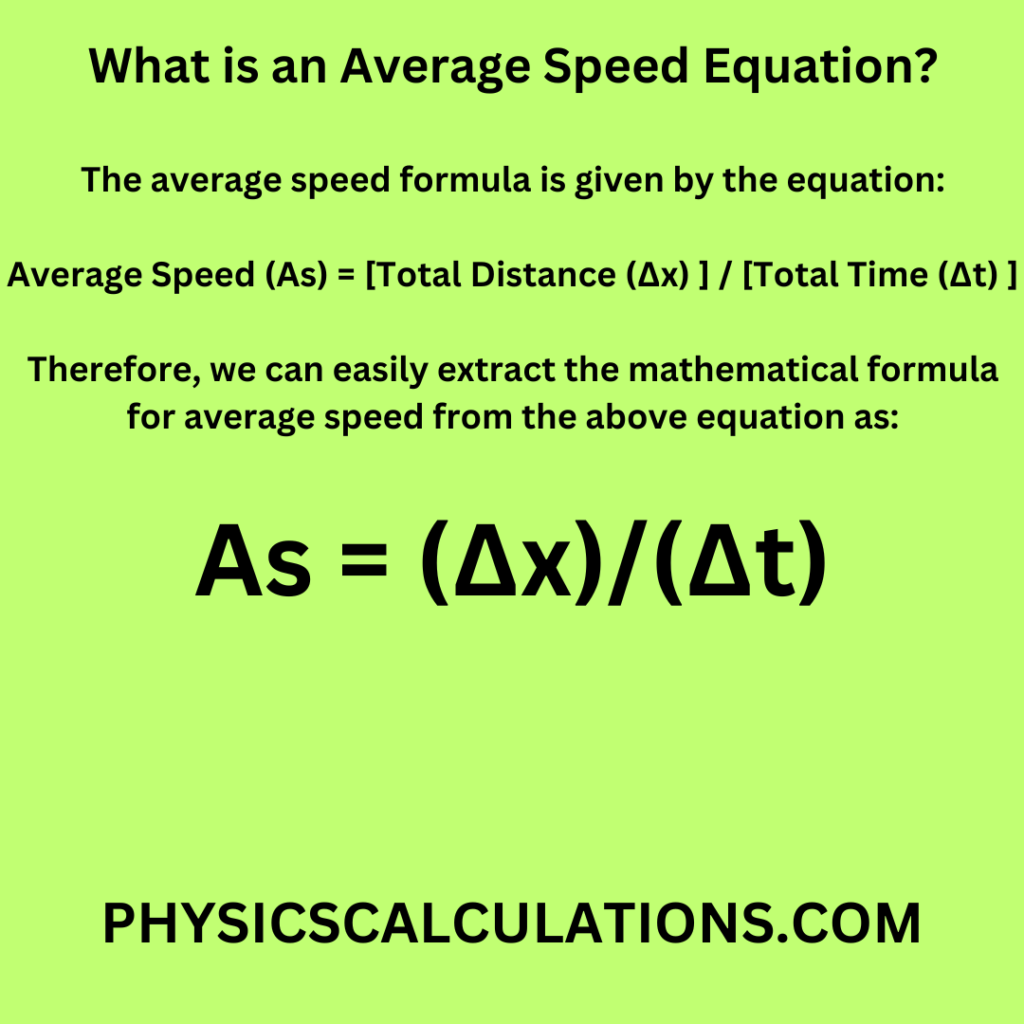
What is an Average Speed Equation?
The average speed formula is given by the equation:
Average Speed (As) = [Total Distance (∆x) ] / [Total Time (∆t) ]
Therefore, we can easily extract the mathematical formula for average speed from the above equation as:
As = (∆x)/(∆t)
This formula calculates the average rate at which an object travels over a certain distance during a specified time interval. The total distance travelled is divided by the total time taken to cover that distance, providing a single value representing the average speed.
Speed is a fundamental concept in physics and everyday life. Whether you’re driving a car, running a race, or measuring the velocity of an object, understanding how to calculate speed is essential. In this article, we will delve into the average speed equation, exploring its components, applications, and practical examples. By the end, you’ll have a solid grasp of how to calculate average speed and how it relates to various scenarios.
Average Speed Equation: Explained

To begin, let’s define the average speed. Average speed is the total distance travelled divided by the total time taken. Mathematically, it can be expressed as:
As = (∆x)/(∆t)
The average speed equation allows us to determine how fast an object is moving on average over a given distance and time period. It is important to note that average speed does not provide information about the object’s instantaneous speed at any specific moment.
Components of the Average Speed Equation
To gain a deeper understanding, let’s break down the average speed equation into its components:
- Total Distance: This refers to the entire length or distance travelled by an object. It can be measured in various units such as meters, kilometres, miles, or any other unit of length appropriate for the given context.
- Total Time: This represents the duration it takes for the object to travel the total distance. Time is typically measured in seconds, minutes, hours, or even days, depending on the scale of the movement.
The Importance of Average Speed Calculation
Calculating average speed is crucial in many real-life situations. By knowing how to determine average speed, we can analyze and compare the efficiency and performance of different objects, vehicles, or individuals. Some practical applications of average speed calculation include:
1. Automotive Industry
In the automotive industry, average speed is an important metric to evaluate vehicle performance and fuel efficiency. Manufacturers and researchers often conduct tests to determine the average speed of a vehicle under various driving conditions. This information helps in optimizing fuel consumption and designing vehicles that offer better mileage.
2. Sports and Athletics
Average speed plays a significant role in sports and athletics. Coaches, trainers, and athletes use average speed calculations to analyze performance, set goals, and track progress. It helps in identifying areas for improvement and measuring the overall effectiveness of training regimens.
3. Logistics and Delivery Services
In logistics and delivery services, knowing the average speed of vehicles and couriers is essential for planning routes, estimating delivery times, and optimizing operations. By understanding the average speed, companies can improve their scheduling, increase efficiency, and enhance customer satisfaction.
4. Scientific Research
Scientists and researchers rely on average-speed calculations when studying the movement of objects or organisms. Whether it’s tracking the migration patterns of birds or analyzing the motion of celestial bodies, average speed provides valuable insights into the dynamics of motion and the natural world.
Solved Problems Using Average Speed Equation
Let’s explore some practical examples to illustrate the application of the average speed equation:
Problem 1: Car Journey
Suppose you are travelling from City A to City B, a distance of 300 kilometres. The journey takes a total of 5 hours, including rest stops. To calculate the average speed, we divide the total distance by the total time:
From the average speed equation; As = (∆x)/(∆t)
We will now, substitute the above equation with our data from the question to obtain
Average Speed = 300 km / 5 hours = 60 km/h
In this case, the average speed of the car during the journey is 60 kilometres per hour.
Problem 2: Running Race
Consider a marathon where the total distance is 42.195 kilometres, and the winning time is 2 hours and 10 minutes. To determine the average speed of the winning runner, we convert the time to hours:
Total Time = 2 hours + (10 minutes / 60) hours = 2.167 hours
Then, we apply the average speed equation:
As = (∆x)/(∆t)
Average Speed = 42.195 km / 2.167 hours ≈ 19.49 km/h
Thus, the average speed of the winning runner in the marathon is approximately 19.49 kilometres per hour.
Frequently Asked Questions
Q: Can average speed be greater than the maximum instantaneous speed?
A: No, average speed is always equal to or less than the maximum instantaneous speed. The average speed considers the entire distance and time traveled, while the maximum instantaneous speed represents the highest speed achieved at any specific moment.
Q: Is average speed the same as average velocity?
A: No, average speed and average velocity are different. Average speed only considers the magnitude of the displacement, while average velocity takes into account both magnitude and direction. Velocity is a vector quantity, whereas speed is a scalar quantity.
Q: How can I calculate average speed if the object’s speed changes during the journey?
A: If the object’s speed varies during the journey, you can calculate the average speed by dividing the total distance traveled by the total time taken. To obtain a more accurate representation, you can break the journey into smaller intervals and calculate the average speed for each interval.
Q: What is the difference between average speed and instantaneous speed?
A: Average speed represents the overall speed over a given distance and time, while instantaneous speed refers to the speed at a specific moment or instant. Average speed provides an average value, whereas instantaneous speed captures the speed at a particular point in time.
Q: Can the average speed be negative?
A: No, average speed cannot be negative. Since average speed is calculated by dividing the total distance by the total time, both distance and time are considered positive quantities. Therefore, the average speed will always be positive or zero.
Q: How does average speed relate to acceleration?
A: Average speed and acceleration are distinct concepts. Average speed measures the rate of distance covered per unit of time, whereas acceleration measures the rate of change of velocity. While both concepts involve speed, acceleration takes into account changes in velocity, including speeding up, slowing down, or changing direction.
Conclusion
Understanding the average speed equation is essential for comprehending the dynamics of speed calculation. By applying the equation, we can determine the average speed of objects, vehicles, and individuals in various contexts.
Average speed provides valuable insights into performance, efficiency, and the dynamics of motion. Whether you’re a student, an athlete, or someone curious about the mechanics of speed, the average speed equation is a fundamental tool for your understanding.
You may also like to read: