What is Transverse Waves?
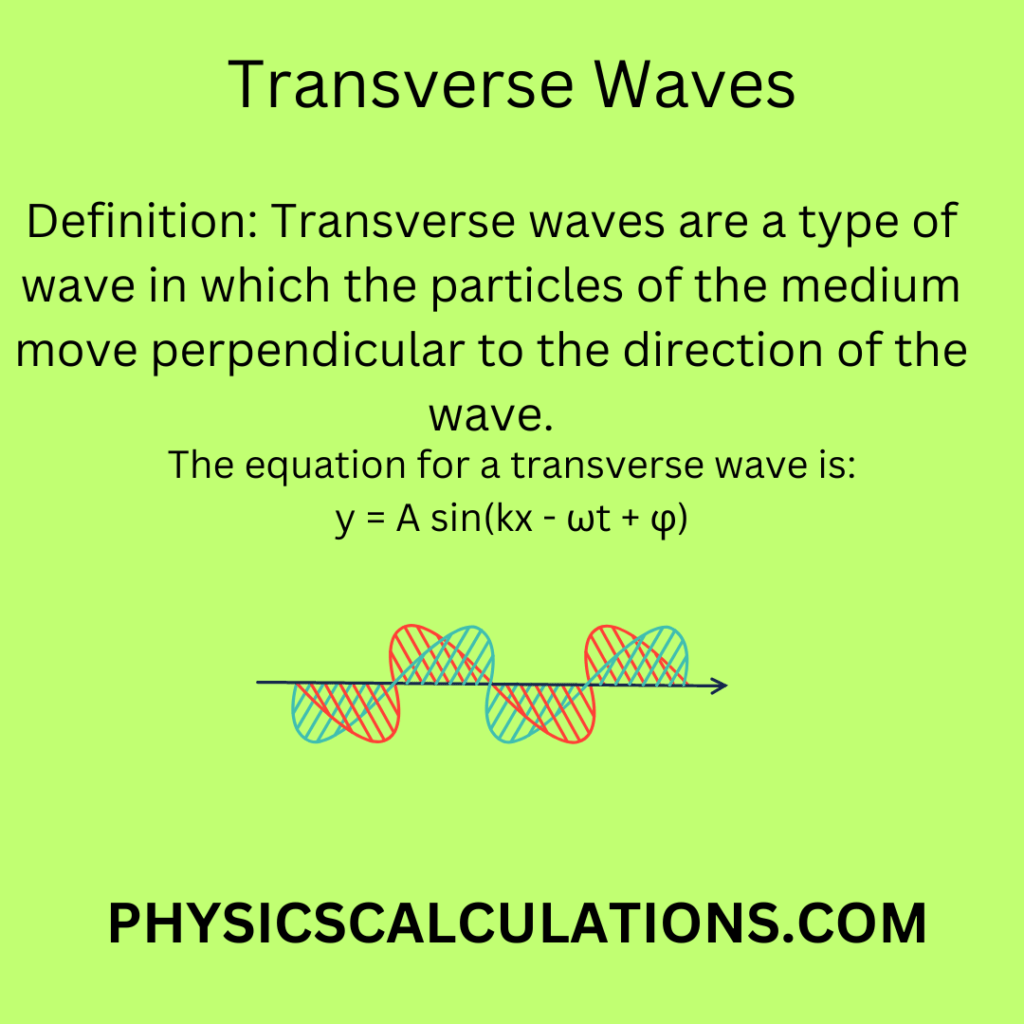
Transverse waves are a type of wave in which the particles of the medium move perpendicular to the direction of the wave. They occur when a disturbance, such as vibration, happens in a medium, causing the particles to move up and down or side to side. Therefore, this type of wave is characterized by the motion of the medium, which is perpendicular to the direction of the wave.
Waves are a fundamental concept in physics, and they come in various forms, each with its unique characteristics and behavior. In this article, we’ll delve into the intriguing world of transverse waves, exploring their fundamental nature, properties, and applications. To begin our journey, it’s essential to understand what sets transverse waves apart from their counterparts: longitudinal waves.
Characteristics of Transverse Waves
Transverse waves have several unique characteristics that differentiate them from other types of waves. These include wavelength, amplitude, frequency, and period.
Wavelength
The wavelength of a transverse wave is the distance between two consecutive points in the wave that are in phase with each other. We measure wavelength in meters, and it determines the distance the wave travels during one complete cycle. Additionally, the longer the wavelength, the lower the frequency of the wave.
Amplitude
The amplitude of a transverse wave is the maximum displacement of the medium from its rest position. We measure amplitude in meters and determine the intensity or strength of the wave. The larger the amplitude, the more energy the wave carries.
Frequency
The frequency of a transverse wave is the number of complete cycles that occur in one second. The unit of measurement for frequency is Hertz (Hz), and it determines the pitch or tone of the wave. The higher the frequency, the higher the pitch.
Period
The period of a transverse wave is the time it takes for one complete cycle to occur. The unit of the period is seconds and is the reciprocal of the frequency. The shorter the period, the higher the frequency.
Transverse Waves vs. Longitudinal Waves
Transverse waves are a type of mechanical wave in which the oscillations occur perpendicular to the wave’s direction. Imagine a rope attached to a wall, and you create a transverse wave by shaking it side to side. The waves move horizontally, but the particles of the rope oscillate vertically.
In contrast, longitudinal waves are characterized by oscillations that happen in the same direction as the wave’s propagation. Think of compressing and stretching a spring. Here, the waves travel back and forth, and the particles in the spring move along this same axis.
Despite these differences, both types of waves share a crucial commonality: they transfer energy from one place to another without transporting matter. This fundamental principle underpins the behavior of all waves in the universe.
Contrast with Longitudinal Waves
To better grasp the distinction between transverse and longitudinal waves, consider the example of sound waves. Sound waves are longitudinal in nature; they cause compression and expansion of air molecules in the direction of their propagation. This compression and expansion result in the transfer of sound energy through the medium.
Mathematical Formulation and Superposition
Mathematical Formulation
Mathematically, we can describe a plane linearly polarized sinusoidal transverse wave. This description includes parameters such as amplitude, period, speed of propagation, and phase. The displacement of the particles in the wave can be represented using vectors and inner products, allowing us to precisely analyze and predict the behavior of transverse waves.
Superposition Principle
One of the intriguing aspects of transverse waves is their ability to create complex oscillations through the superposition of simple sinusoidal waves. This principle means that we can understand the behavior of waves by considering the sum of individual wave components. For instance, when you pluck a violin string, the resulting sound is a combination of various transverse waves, each with its own amplitude and frequency.
Circular polarization is another fascinating concept that can be understood through the superposition of linearly polarized waves. By adding two orthogonal linearly polarized waves with a phase difference of 90 degrees, we create a circularly polarized wave. This phenomenon finds applications in various fields, including optics and telecommunications.
Circular Polarization and Power Calculation
The Circular Polarization
Circular polarization is a unique characteristic of transverse waves, and it is produced by circular motion. It involves the oscillation of wave components in a circular pattern. The circular polarization concept also gives rise to elliptical polarization, where the wave motion is elliptical in shape. Understanding elliptical polarization involves decomposing it into orthogonal linear motions.
Power Calculation
In the world of transverse waves, understanding the power carried by these waves is essential. We can mathematically express both kinetic and potential energy in a transverse wave. By calculating the energy for one wavelength and determining the total energy, we gain insights into the wave’s power. The formula for average power in a transverse wave allows us to quantify the rate at which energy is transmitted.
You may also like to read:
when two progressive waves y1=4 sin(2x-6t)
Transverse Wave Equation
The equation for a transverse wave is:
y = A sin(kx – ωt + φ)
where y is the displacement of the medium from its rest position, A is the amplitude of the wave, k is the wave number, x is the position of the particle in the medium, ω is the angular frequency, t is the time, and φ is the phase constant.
Speed of Transverse Waves
The speed of transverse waves refers to how fast a wave travels through a medium in a direction perpendicular to its propagation. We define it as the distance the wave covers within a given time frame. The speed depends on the characteristics of the medium, such as its density and elasticity.
To determine the wave speed, we need to consider its frequency and wavelength. The frequency measures the number of waves passing through a point per second, while the wavelength is the distance between two consecutive points on the wave.
The mathematical equation v = λf relates the wave speed, wavelength, and frequency. The speed of transverse waves is directly proportional to their frequency and wavelength.
Since f = 1 / T
We can rewrite v = λf as v = λ x 1/T
Which implies that v = λ / T [Where T = period]
The equation v = λf shows that the speed of a transverse wave is directly proportional to its wavelength and frequency. Therefore, if the wavelength or frequency of the wave changes, the speed of the wave will also change.
Additionally, we apply various techniques to measure the speed of transverse waves, such as using a stroboscope to measure the frequency and a laser to measure the wavelength of the wave.
Speed of a Vibrating Spring
The speed of a wave on a vibrating spring is a fundamental concept in physics that relates to the propagation of mechanical waves through a spring-like medium. Also, this speed is determined by several factors, including the mass of the spring, the spring constant, and the tension on the spring.
To understand the speed of a wave on a vibrating spring, we must first define the properties of the spring. We denote the mass of the spring by m, the spring constant by k, and the tension on the spring by F.
To calculate the speed of a wave on a vibrating spring, we can apply the following equation:
v = √(F/m)/√(k/m)
where v is the wave speed.
This equation shows that the speed of the wave is directly proportional to the square root of the tension on the spring and inversely proportional to the square root of the spring constant and the mass of the spring. This means that increasing the tension on the spring or decreasing its mass can increase the speed of the wave.
Important Points on Speed of a Vibrating Spring
In the equation, v = √(F/m)/√(k/m)
F, k, and m represent the physical properties of the spring through which the wave is propagating.
F refers to the tension or force applied to the spring, which affects its stiffness and elasticity. A greater force or tension on the spring can increase the wave speed.
k is the spring constant, which is a measure of the spring’s stiffness or how easily it deforms when a force is applied. A higher spring constant means that the spring is more rigid and harder to deform, which can decrease the wave speed.
m is the mass of the spring, which also affects its ability to deform and respond to a force. A lighter mass can allow the spring to vibrate more easily and increase the wave speed.
By using these variables in the equation, we can determine the speed of a wave that travels through a spring-like medium.
We can equally apply the formula v = √(T/μ) to find the speed of a vibrating spring
[where T = tension on the string, and μ = linear density of the spring]
Examples of Transverse Waves
There are several examples of transverse waves, including electromagnetic waves, surface waves, and sine waves. We also have water waves.
Electromagnetic Waves
Electromagnetic waves are transverse waves that propagate through space without the need for a medium. They are characterized by their frequency and wavelength and include radio waves, microwaves, infrared radiation, and visible light.
An important example of a transverse wave is the electromagnetic wave, which includes visible light, radio waves, and more. These waves don’t require a medium for propagation, as they can travel through a vacuum. The term “transverse” in this context means that the wave’s direction is perpendicular to the displacement of the particles it interacts with. In the case of electromagnetic waves, this displacement refers to the electric and magnetic fields oscillating perpendicular to the wave’s direction.
Surface Waves
Surface waves are transverse waves that occur at the boundary between two different media, such as water and air. They are characterized by their amplitude and wavelength and include ocean waves, seismic waves, and Rayleigh waves.
Sine Waves
Sine waves are a type of transverse wave that are characterized by their regular and repetitive oscillations. They are commonly used in mathematics, physics, and engineering to model periodic phenomena.
Shear Waves
Another fascinating example of transverse waves can be found in elastic solids. When a shear stress is applied to such materials, it generates transverse waves. These waves are characterized by the displacement of solid particles perpendicular to the direction of wave propagation. However, it’s worth noting that fluids are unable to support shear forces at rest, which prevents the propagation of transverse waves within them.
In the field of seismology, these transverse waves are often referred to as secondary waves or S-waves and play a crucial role in understanding the Earth’s interior.
Transverse Waves vs. Longitudinal Waves
Transverse waves differ from longitudinal waves in several ways. While transverse waves have a perpendicular motion of the medium, longitudinal waves have a parallel motion. Additionally, longitudinal waves have compressions and rarefactions, while transverse waves do not. Sound waves are an example of longitudinal waves.
Applications of Transverse Waves
Transverse waves have several practical applications in various fields, including communication, medicine, and engineering.
Communication
We use electromagnetic waves, such as radio waves and microwaves for communication purposes, including television broadcasting, cellular networks, and satellite communications.
Medicine
Transverse waves are used in medical imaging techniques such as ultrasound and MRI. Ultrasound waves are transverse waves that are used to create images of the body’s internal structures, while MRI uses magnetic fields and radio waves to create images of the body’s tissues and organs.
Engineering
Transverse waves are used in various engineering applications, such as in the design and manufacture of electronics, acoustic devices, and seismic equipment.
Summary
Summarily, transverse waves are a type of wave that occurs when the disturbance moves perpendicular to the direction of wave propagation. They have several unique characteristics that differentiate them from other types of waves, including wavelength, amplitude, frequency, and period. Transverse waves have many practical applications in various fields, including communication, medicine, and engineering.
FAQs
Question: What are transverse waves?
Answer: Transverse waves are a type of wave in which the particles of the medium move perpendicular to the direction of the wave.
Question: What is an example of a transverse wave?
Answer: Electromagnetic waves, surface waves, and sine waves are all examples of transverse waves.
Question: How do transverse waves differ from longitudinal waves?
Answer: Transverse waves have a perpendicular motion of the medium, while longitudinal waves have a parallel motion.
Question: What are the practical applications of transverse waves?
Answer: Transverse waves have practical applications in various fields, including communication, medicine, and engineering.
Question: What is the equation for a transverse wave?
Answer: The equation for a transverse wave is y = A sin (kx – ωt + φ), where y is the displacement of the medium from its rest position, A is the amplitude of the wave, k is the wave number, x is the position of the particle in the medium, ω is the angular frequency, t is the time, and φ is the phase constant