Question
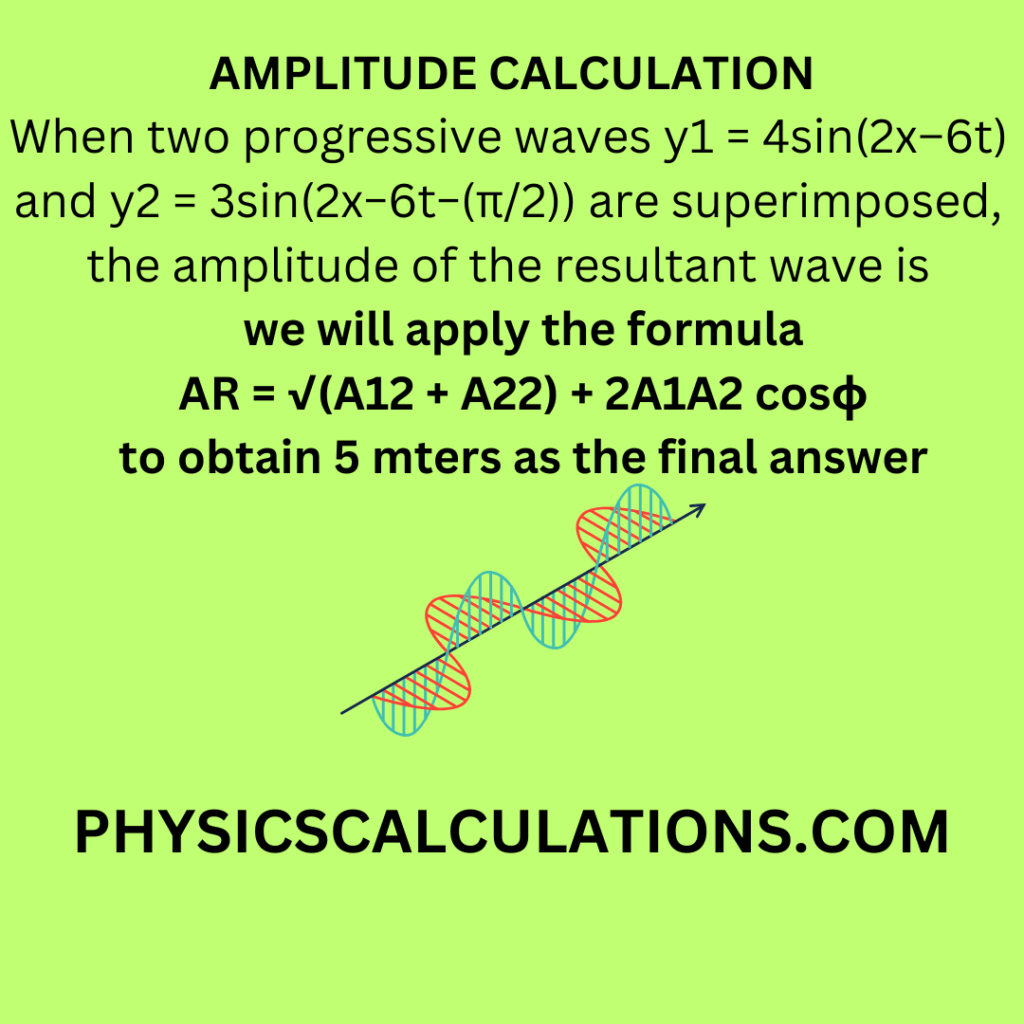
When two progressive waves y1=4 sin(2x–6t) and y2 = 3sin(2x−6t−(π/2)) are superimposed, the amplitude of the resultant wave is
Answer
The final answer to the above question is 5 meters (5m).
Explanation to the Above Answer
Data: Important information from the question
For us to be able to fully understand how to extract our data, we must remember the formula for amplitude is
y = A sin (ωt + ϕ)
Where y = displacement of the wave, A = amplitude, ω = angular velocity, t = time, and ϕ = phase angle
From the above question, when we compare y1 = 4sin(2x–6t), and y2 = 3sin(2x−6t−(π/2)) with the formula y = A sin (ωt + ϕ). We will have
y1 = 4sin(2x–6t) which implies that A1 = 4
y2 = 3sin(2x−6t−(π/2)) which also shows that A2 = 3
It’s also obvious that ϕ = π/2
But π = 1800
Therefore, π/2 = 1800 / 2 =900
Unrevealed value:
Resultant Amplitude, AR = ?
Formula:
We will use AR = √(A12 + A22) + 2A1A2 cosϕ
Solution
We will now insert our data into AR = √(A12 + A22) + 2A1A2 cosϕ to solve the problem
AR = √(A12 + A22) + 2A1A2 cosϕ = AR = √(42 + 32) + 2 x 4 x 3 x cos900
But cos900 = 0
Which implies that
AR = √(16 + 9) + 2 x 4 x 3 x 0
Thus
AR = √(25) + 0
Therefore
AR = √(25) + 0 = √(25) = 5 m
Therefore, the amplitude of the resultant wave is 5 meters.
y = A sin (ωt + ϕ)
You may also like to read: