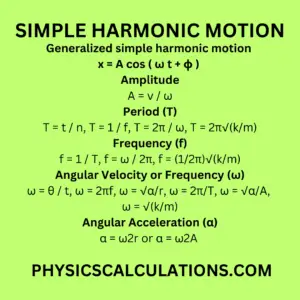
Simple Harmonic Motion Formulae
Simple Harmonic Motion (SHM) is a fundamental concept in physics that describes the repetitive, back-and-forth motion exhibited by various systems. The key formula related to simple harmonic motion include:
Video Explanation
Here is a video explanation of simple harmonic motion and how to apply its equations to solve a problem:
1. Generalized Equation of Simple Harmonic Motion
The formula for a generalized simple harmonic motion is
x = A cos ( ω t + ϕ )
Where x is the displacement
A = Amplitude
ω = Angular velocity
t = time
ϕ = Phase angle
We use a generalized equation for the simple harmonic motion of a body that undergoes oscillation around a stable equilibrium position. Furthermore, for us to apply this formula [x = A cos ( ω t + ϕ )], the system should exhibit a restoring force proportional to its displacement from the equilibrium position. Additionally, the motion should be periodic with a constant frequency and amplitude. The acceleration needs to be directly proportional to the displacement in the opposite direction.
| You may also like to read: |
| What is Simple Harmonic Motion in Physics |
| Simple Pendulum: Definition, Formula, and Calculations |
| what is the system’s potential energy when its kinetic energy is equal to 34e? |
2. Amplitude (A)
The formula for calculating the amplitude of a simple harmonic motion is:
A = v / ω
Where A is the amplitude
v = linear speed or velocity
ω = angular velocity
3. Period (T)
The period of a simple harmonic motion is the time taken to complete one cycle, oscillation or vibration. The formula to calculate the period of an oscillating body is
Period (T) = Time taken (t) / number of oscillations (n)
Which implies that T = t / n
We can also use the following formulae for period:
a. T = 1 / f
b. T = 2π / ω
c. T = 2π√(k/m)
Where f is the frequency of oscillations.
k = force constant
Period is measured in seconds (s).
4. Frequency (f)
Frequency of a simple harmonic motion is the number of complete oscillations per second. The formula for calculating frequency is
Frequency (f) = 1 / period (T)
which shows that
f = 1 / T
The other formulae for frequency is f = ω / 2π or f = (1/2π)√(k/m)
ω = angular frequency
k = force constant
m = mass of the body
The unit is a cycle per second (s-1) or Heartz(Hz).
5. Angular Velocity or Frequency (ω)
The formula for calculating angular velocity is
ω = θ / t, or ω = 2πf
Where ω = Angular velocity
θ = Angle
t = time
f = frequency
We can also use the formula below to calculate angular velocity
ω = √α/r, ω = 2π/T or ω = √α/A
The unit of angular velocity is radians per second (rads-1)
Another formula we can use for angular frequency is
ω = √(k/m)
where k = force constant
m = mass of the body
6. Angular Acceleration (α)
For angular acceleration, we use
α = ω2r or α = ω2A
The unit of angular acceleration is also radians per second (rads-1)
7. Linear Speed or Velocity (v)
The formula for linear speed is
v = ωr or v = ωA
We can also use the formula below to calculate linear speed
v = ω√(A2 – x2)
The unit for linear speed is meters per second (m/s)
8. Linear Acceleration (a)
The formula for calculating linear acceleration of an oscillating body is
a = αr
r = radius (measured in meters, m)
9. Meaning of 3600 in Simple Harmonic Motion
In a simple harmonic motion, 3600 = 2π rad
10. Restoring Force Exerted by a Spring
F = – kx
Where
k = force constant
x = displacement
11. Energy in Simple Harmonic Motion
The formula for energy in simple harmonic motion is
E = (1/2)mv2 + (1/2)kx2
Note:
If θ = Angle
r = A = radius of the circle
s = distance
Then
θ = s/r
You may also like to read:
The amplitude of SHM y=2(sin5πt+√2cosπt) is
A body moves along a circular path
If a wheel 1.2m in diameter rotates at one revolution per second