What is Resistivity?
Resistivity Formula and Definition: Resistivity is a physical property of materials that describes their ability to resist the flow of electric current. The si unit of resistivity is in ohm-meters (Ωm). It is determined by the material’s length, Area, and resistance or temperature.
Materials with high resistivity impede the flow of electric current more than materials with low resistivity. Resistivity is an important property in the design and use of electrical and electronic components and systems.
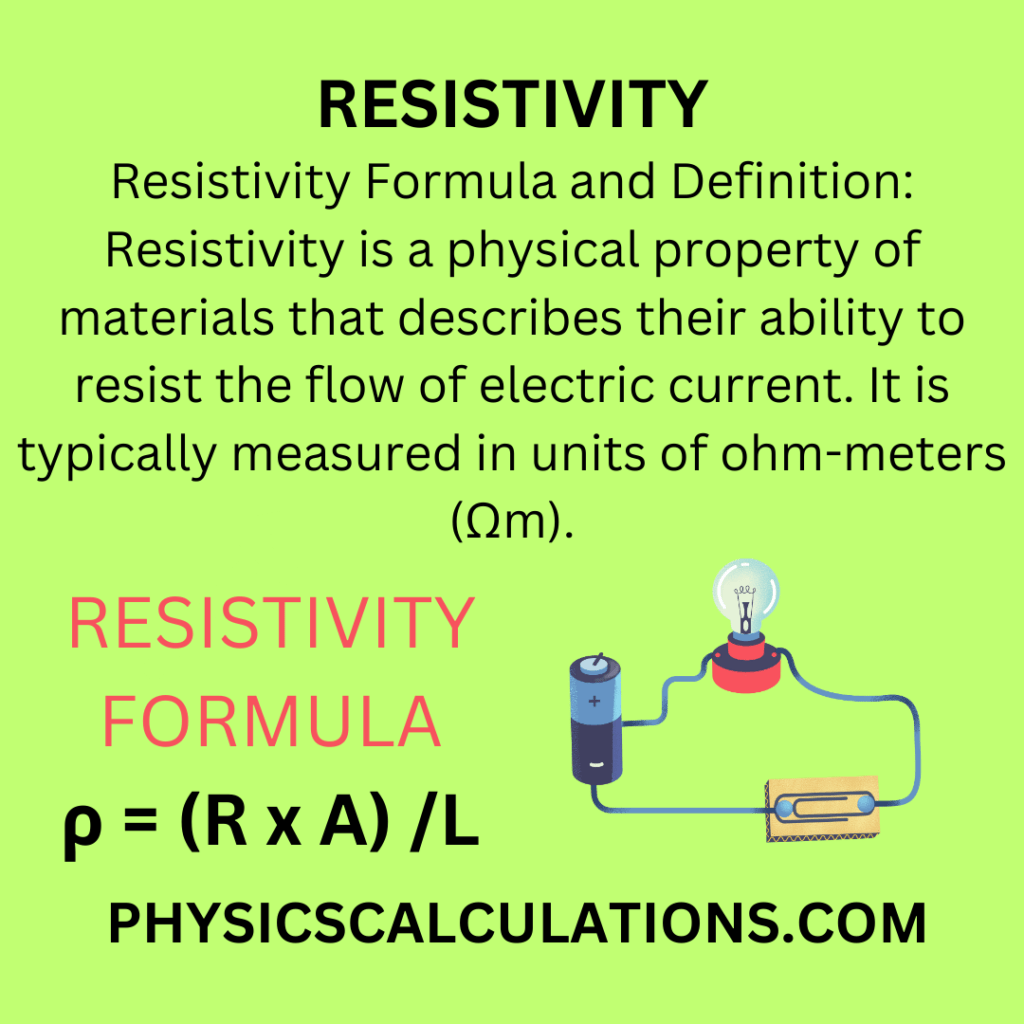
The above picture describes the formula we use to calculate resistivity. We will now discuss the resistivity formula in the section below.
Read: Resistance vs Resistivity
Resistivity Formula
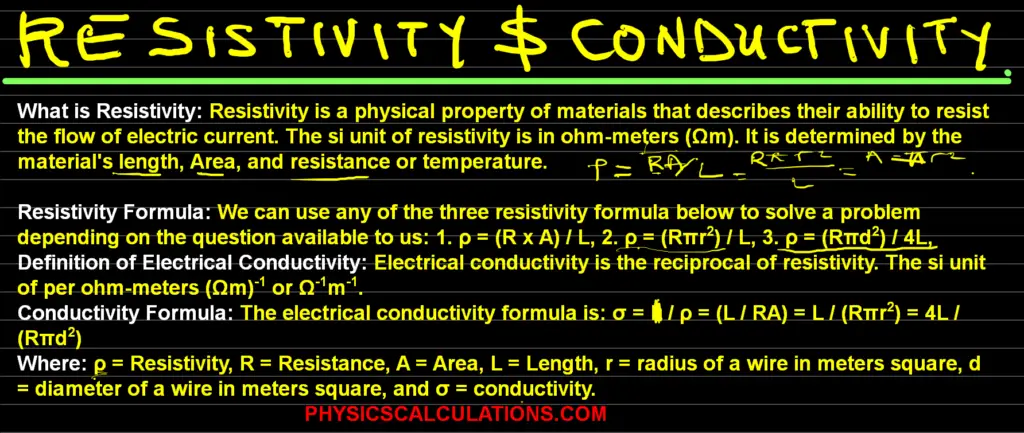
We can use any of the three resistivity formula below to solve a problem depending on the question available to us:
- ρ = (R x A) / L,
or
2. ρ = (Rπr2) / L,
or
3. ρ = (Rπd2) / 4L,
Where
ρ = Resistivity
R = Resistance
A = Area
L = Length
r = radius of a wire in meters square
d = diameter of a wire in meters square
Electrical Conductivity Formula
Definition of Electrical Conductivity: Electrical conductivity is the reciprocal of resistivity. The si unit of per ohm-meters (Ωm)-1 or Ω-1m-1.
The electrical conductivity formula is: σ = 1 / ρ = (L / RA) = L / (Rπr2) = 4L / (Rπd2)
Therefore, you can use any of the above formulas to calculate conductivity.
Additionally, you can simply find the resistivity and then divide it by one (1) to obtain the conductivity of the material.
Points to Note While Calculating Resistivity or Conductivity
Always make sure you do not your calculations in uniform units of measurement. Avoid mixing up m and cm or m and mm. You need to apply your knowledge of prefixes in physics.
Therefore, its preferable to express all measurements in si units.
You may also like to read:
Resistivity Formula: Practice Problems
Here are a few solved problems on resistivity:
Problem 1
Calculate the resistivity of a wire length 4m and cross-sectional area 4×10-7m2 if its resistance is 3.0 ohms.
[UTME 2019]
Solution
Data: To calculate the resistivity of a wire, the first step is to bring out the data from the question above
As we can see,
Length of the wire (L) = 4m
Cross-sectional Area (A) = 4×10-7m2
Resistance of the wire (R) = 3Ω
Resistivity of the wire (ρ) = ?
The formula is given as
Resistivity formula: ρ = (RA) / L
Therefore, by substituting the values of ρ, R and L into the above equation, we will have
ρ = ( 3 x 4×10-7) / 4
The above expression will give us
ρ = 0.0000012 / 4
Therefore, the resistivity of a wire is
ρ = 0.0000003
ρ = 3×10-7Ωm
Hence, the resistivity of the wire is 3×10-7Ωm
Problem 2
What is the resistivity of a cylindrical material wire whose 1.0 meters length has a resistance of 2.0 ohms? The diameter of the wire is 0.5 millimetres.
Answer
The final answer to the above question is 3.93 x 10-7 ohm – meter
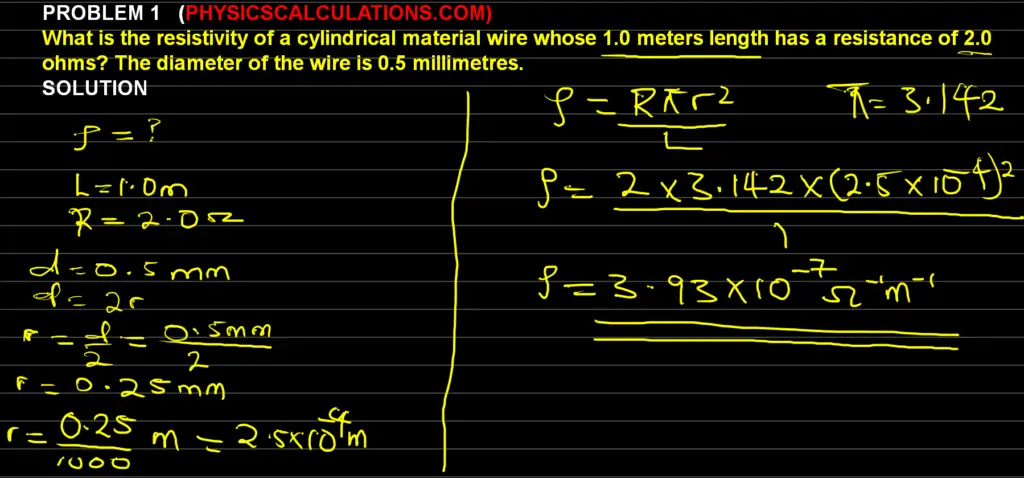
Explanation
L = 1.0 m
r = d/2 = 0.5 / 2 = 0.25 mm
We can now covert r into meters
r = 0.25 mm = 0.25/1000 = 2.5 x 10-4 m
A = πr2 = 3.142 x ( 2.5 x 10-4)2
Since the resistivity formula is ρ = (R x A) / L
ρ = (2 x 3.142 x 2.5 x 10-4)2 / 1
ρ = 3.93 x 10-7 Ωm
Therefore, the resistivity of the cylindrical material is 3.93 x 10-7 ohm – meter
Problem 3
A wire of length 100 cm and cross-sectional area 2.0 x 10-3 cm2 has a resistance of 0.10 Ω. Calculate its electrical conductivity.
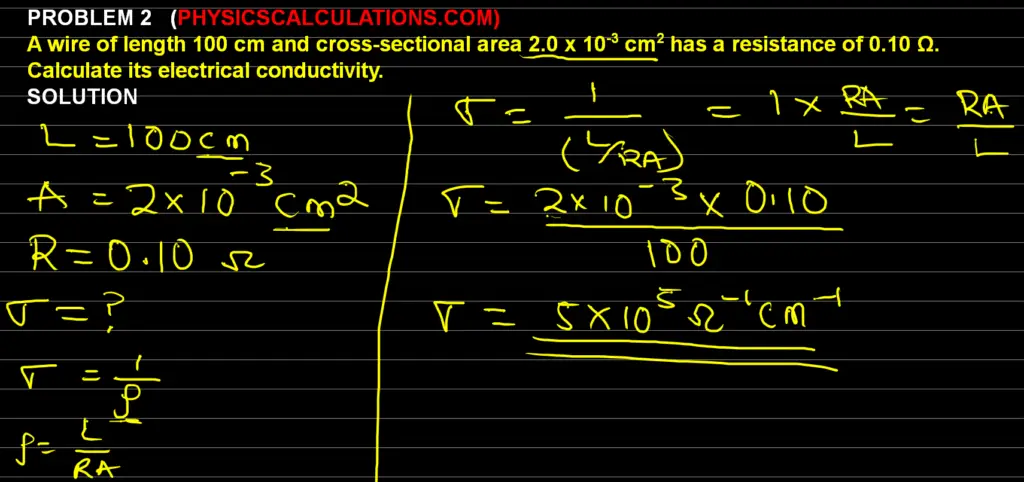
Solution
Length (L) = 100 cm, cross-sectional area (A)= 2.0 x 10-3 cm2, and Resistance (R) = 0.10 Ω
Applying the formula for conductivity, we will have:
σ = 1 / ρ = (100 cm / 0.10 Ω x 2.0 x 10-3cm2) = 5 x 105 Ω-1cm-1
Therefore, the electrical conductivity of the wire is 5 x 105 Ω-1cm-1
Problem 4
A constantan wire has a cross-sectional area of 4 x 10-8 m2 and a resisitivity of 1.1 x 10-6 Ωm. If a resisitor of resistance 11 Ω is to be made from this wire, calculate the length of the wire required.
Solution
A = 4 x 10-8 m2, ρ = 1.1 x 10-6 Ωm, and R = 11 Ω
We are to find L
Formula: L = RA / ρ = (11 x 4 x 10-8) / 1.1 x 10-6 = 0.4 m
Problem 5
A 2 meters wire of resistivity 5.5 x 10-7Ωm has a cross-sectional area of 0.50 mm2. Calculate its resistance.
Solution
L = 2 m, ρ = 5.5 x 10-7Ωm, and A = 0.50 mm2 = 0.5 x 10-6 m (1 mm2 = 1 x 10-6 m)
We are to find R
Formula: R = ρL / A = (5.5 x 10-7 x 2) / 0.5 x 10-6 = 2.2 Ω
Therefore, the resistance of the wire is 2.2 ohms.
Problem 6
A wire of length 15 m made of a material of resistivity 1.8 x 10-6 Ωm has a resistance of 0.27Ω. Determine the area of the wire.
Solution
A = ρL / R = (1.8 x 10-6 x 15) / 0.27 = 1.0 x 10-4 m2.
Problem 7
A wire of 5Ω resistance is drawn out so that its new length is two times the original length. If the resistivity of the wire remainsthe same and the cross-sectional area is halved, what will be the new resistance?
Solution
Original Wire – R1 = 5Ω, L1 = L, ρ1 = ρ, and A1 = A
Formula for the original wire => ρ1 = R1A1 / L1
New Wire – R2 = ?, L2 = 2L (twice the original length), ρ2 = ρ (resistivity remains the same), A = A/2 (cross-secrtional area is halved)
Formula for the new wire => ρ2 = R2A2 / L2
When we equate the original wire with the new wire, we will now obtain
ρ1 = ρ2
Therefore,
R1A1 / L1 = R2A2 / L2
Thus, we will now make R2 subject of the formula
R2 = R1A1L2 / A2L1
Putting the values of our data into the above equation, we will now have:
R2 = (5 x A x 2L) / ((A/2) x L) = (5 x 2 x A x 2L) / (A x L) = 5 x 2 x 2 = 20Ω
Therefore, the new resistance (R2) is 20 Ohms.
Resistivity vs Conducitivity
Below is a table summarizing the main differences between resistivity and conductivity:
| Property | Resistivity | Conductivity |
|---|---|---|
| Definition | A material’s resistance to the flow of electric current. | A material’s ability to conduct electric current. |
| Symbol | ρ (rho) | σ (sigma) |
| Unit | Ohm-meters (Ω·m) | Siemens per meter (S/m) or mhos per meter (mho/m) |
| Measurement | Indirectly measured using a resistivity meter or ohmmeter. | Directly measured using a conductivity meter. |
| Relationship | Inversely related to conductivity: σ = 1/ρ or ρ = 1/σ. | Inversely related to resistivity: σ = 1/ρ or ρ = 1/σ. |
| Material Types | Insulators have high resistivity. | Metals have high conductivity. |
| Temperature Effect | In most materials, resistivity increases with temperature. | In most materials, conductivity decreases with temperature. |
| Role in Electrical Engineering | Crucial for analyzing and designing electrical circuits. | Important for selecting suitable materials for electrical components. |
| Influence on Wire Thickness | Lower resistivity materials require thicker wires for the same current. | Higher conductivity materials can use thinner wires for the same current. |
| Impurity Effect | Impurities can increase resistivity. | Impurities can decrease conductivity. |
Please note that resistivity and conductivity are mathematically related, and as one property increases, the other decreases, and vice versa. This table above highlights their key differences. Therefore, it makes it easier to understand their distinct roles and characteristics.
Resistivity vs Resistance
Here is another table summarizing the main differences between resistivity and resistance:
| Property | Resistivity | Resistance |
|---|---|---|
| Definition | Intrinsic property of a material that describes how strongly it resists the flow of electric current. | Property of an object or material that measures the opposition to the flow of electric current. |
| Symbol | ρ (rho) | R |
| Unit | Ohm-meters (Ω·m) | Ohms (Ω) |
| Measurement | Measured for materials. | Measured for objects or components. |
| Dependent on | Material composition, temperature, and impurities. | Material’s resistivity and its physical dimensions (length and cross-sectional area). |
| Relationship | Related to conductivity: σ = 1/ρ or ρ = 1/σ. | Related to resistivity: R = ρ * (L / A), where “L” is length and “A” is cross-sectional area. |
| Application | Used to analyze material behavior under electric fields. | Used to analyze and design electrical circuits and systems. |
| Examples | Different materials have different resistivity values. | Components like resistors have specific resistance values. |
| Temperature Effect | Resistivity may increase or decrease with temperature, depending on the material type. | Resistance typically increases with temperature in conductors. In some cases, it may decrease in certain semiconductors. |
| Material Property | An intrinsic property of the material itself. | Depends on both the material and its physical dimensions. |
| Formula | No direct formula; related to conductivity. | R = ρ * (L / A) |
The table above highlights the key distinctions between resistivity, which is a material property, and resistance, which depends on both the material and its dimensions. Therefore, as resistivity describes how a material resists electric current flow, resistance quantifies the opposition to current flow in a specific object or component.
Frequently Asked Questions about Resistivity and Conductivity
Q1: What is Resistivity?
Resistivity, denoted by the symbol “ρ” (rho), is a fundamental property of a material that describes how strongly it resists the flow of electric current. It is an intrinsic characteristic of a material, independent of its shape or size. Resistivity is measured in ohm-meters (Ω·m) and is a crucial parameter in determining the electrical behavior of various materials.
Q2: What is Conductivity?
Conductivity is the reciprocal of resistivity and is represented by the symbol “σ” (sigma). It measures the ability of a material to conduct electric current. The higher the conductivity of a material, the better it can conduct electricity. Conductivity is also measured in siemens per meter (S/m) or mhos per meter (mho/m).
Q3: How are Resistivity and Conductivity related to each other?
Resistivity and conductivity are mathematically related through the equation: σ = 1/ρ or ρ = 1/σ. As one property increases, the other decreases, and vice versa. Highly conductive materials have low resistivity, while materials with high resistivity have low conductivity.
Q4: How does Temperature affect Resistivity and Conductivity?
In most materials, as the temperature increases, the resistivity also increases. This means that the conductivity decreases with rising temperature. However, in some materials known as “positive temperature coefficient” materials, the resistivity decreases with increasing temperature, leading to an increase in conductivity.
Q5: What are the units of Resistivity and Conductivity?
The units of resistivity are ohm-meters (Ω·m), while the units of conductivity are siemens per meter (S/m) or mhos per meter (mho/m).
Q6: What are some examples of materials with high Resistivity?
Materials like rubber, glass, ceramics, and most insulators have high resistivity. These materials are generally poor conductors of electricity due to the presence of tightly bound electrons, which hinder the flow of current
Q7: What are some examples of materials with high Conductivity?
Metals, such as copper, aluminum, silver, and gold, exhibit high conductivity due to their abundance of free electrons that can easily carry electric charges.
Q8: How is Resistivity different from Resistance?
Resistivity is an intrinsic property of a material, while resistance depends on both the resistivity of the material and its physical dimensions. Resistance (R) is given by the equation R = ρ * (L / A), where “L” is the length and “A” is the cross-sectional area of the material.
Q9: What factors influence the Resistivity of a material?
The resistivity of a material depends on its composition, temperature, and the presence of impurities. Crystalline structures tend to have lower resistivity than amorphous materials.
Q10: Why is the concept of Resistivity important in Electrical Engineering?
Resistivity plays a crucial role in the design and analysis of electrical circuits and systems. It helps engineers understand how different materials will behave under the flow of electric current, allowing them to choose the right materials for specific applications.
Q11: How is Resistivity measured experimentally?
Resistivity is measured using a device called a “resistivity meter” or “ohmmeter.” The measurement involves passing a known current through a sample of the material and measuring the voltage drop across it. The resistivity is then calculated using the dimensions of the sample.
Q12: Can Resistivity be used to predict a material’s behavior in an electric field?
Yes, resistivity is an essential parameter for predicting how a material will respond to an electric field. Materials with higher resistivity will experience more significant voltage drops and dissipate more power, whereas highly conductive materials will carry current with minimal losses.
Q13: How does Moisture affect the Resistivity of materials?
Moisture can significantly alter the resistivity of certain materials, particularly insulators. Water, being a good conductor, can create paths for current flow, reducing the overall resistivity of the material. This effect can lead to electrical failures in insulating materials in humid environments.
Q14: Are there materials with variable Resistivity?
Yes, certain materials exhibit a property called “variable resistivity” or “resistive switching.” These materials can change their resistivity under the influence of an electric field, allowing them to be used in devices like resistive random-access memory (RRAM) and memristors.
Q15: What is the role of Resistivity in determining the thickness of electrical wires?
Resistivity is taken into consideration when determining the appropriate thickness of electrical wires. Lower resistivity materials require thinner wires to achieve the desired current-carrying capacity without significant power losses, while high resistivity materials necessitate thicker wires for the same purpose.
I hope this article has helped you understand the general topic on resistivity definition, resistivity formula, resistivity units, and resistivity calculations. To simplify the topic, I added conductivity definition, conductivity unit, conductivity formula, and conductivity calculations to broaden your understanding. I wish you goodluck!
You may also like to read: