Concept of Refractive Index
Definition: Refractive index is a constant that is used to express the nature of the medium through which light ray is propagated. When a ray of light passes through a medium, the refractive index decides the length of it is permeability. Now, we will apply simple steps to help you understand how to calculate an apparent displacement of a refractive index.
The symbol for the refractive index is μ and is constant
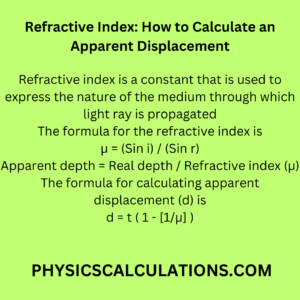
The formula for the refractive index is
μ = (Sin i) / (Sin r)
Also,
Refractive index (μ) = real depth / apparent depth
Therefore, we can now see that
Apparent depth = Real depth / Refractive index (μ)
Which is the formula for calculating apparent depth
The formula for calculating apparent displacement (d) is
d = t ( 1 – [1/μ] )
Where
d = apparent displacement
t = thickness
μ = refractive index
Refractive Index: How to Calculate an Apparent Depth and Displacement
Here are a few examples that will help you understand how to calculate an apparent displacement
Example 1
An ant is found under a regular glass slab of thickness 10.0cm and a refractive index of 1.7. Calculate the apparent displacement of the ant if viewed vertically from above the slab.
[UTME 2019]
Solution
To calculate the apparent displacement of the ant, we need to bring out the data from the question above
Data:
The thickness of the glass slab is the same as the Real depth ( t ) = 10.0cm
The refractive index of the glass slab is ( μ ) = 1.7
Now the last statement is telling us to calculate the apparent displacement ( d ) = ?
We can now use the formula below to calculate the apparent displacement
d = t ( 1 – [1/μ] )
Now we substitute the values of our data into the above equation
d = 10 ( 1 – [1/1.7] )
By dividing [1/1.7] inside the fraction we have 0.588
Now put [1/1.17] = 0.588 into the main d
d = 10 ( 1 – 0.588 )
Therefore
d = 10 x 0.412
which implies that our final answer is
d = 4.12cm
Therefore, the calculated apparent displacement of the ant vertically viewed from above the slab is 4.12cm
Example 2
A microscope is focused on a table when a mark is covered by a plate of glass, 3cm thick. The microscope has to be placed 1.18 centimeters for the mark to be on a small focus. Calculate the refractive index of the medium.
Solution:
Data
Let us go through the question and pick our data
d = 1.18 cm
t = 3 cm
μ = ?
Now we need to apply the formula for apparent displacement
d = t ( 1 – [1/μ] )
Now we substitute our values from our data into the above formula
1.18 = 3 ( 1 – [1/μ] )
This is equal to
1.18 / 3 = ( 1 – [1/μ] )
Now we have
0.39 = 1 – [1/μ]
By taking 1 to the side of 0.39, we have
0.39 – 1 = – [1/μ]
Now we multiply each side by -1 to get
-1 ( 0.39 – 1) = – 1 ( – [1/μ] )
since multiplying negative by negative will give positive and negative by positive will give negative, we now have
1 – 0.39 = 1/μ
Simple arithmetic will give us
0.61 = 1/μ
By cross multiplication to make μ subject of the formula, we have
μ = 1/ 0.61 = 1.64
You may also like to check:
Resistivity Formula: Definition, Unit and Calculations
You can also check our websites:
Wokminer – Search and apply for a job