What is a Position in physics?
Definition of position in physics: Position in physics simply means the location of an object in a plane or space. We can determine the position of an object by considering it is distance and direction from a specific frame of reference. Therefore, this is to say that we can find the position of an object, once we know its distance and direction from a specific point of reference. Watch the video please:
Additionally, position in physics refers to the specific location of an object in a given reference frame. It’s a fundamental concept that helps us describe the spatial coordinates of an object accurately. The position of an object is often represented using coordinates such as Cartesian coordinates (x, y, z) or polar coordinates (r, θ). These coordinates help us understand the object’s location within a given coordinate system.

Explanation of Position in Physics
The location of your television stand in your room is the position of that television stand. The location of your school from your home is the position of that school. A football coach wants his players to occupy a specific position on the field during a match competition. A man driving a car occupies a certain position as he continues to move.
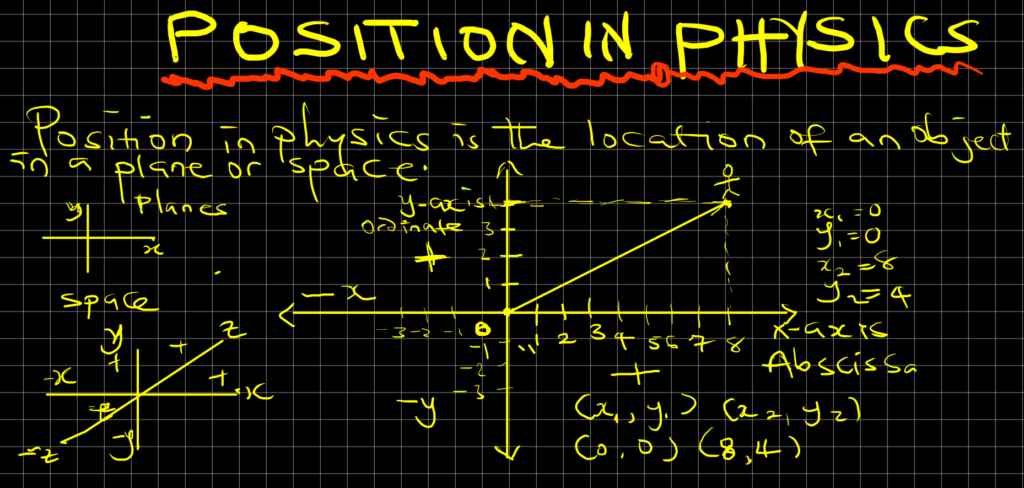
Therefore, finding the position of an object is very important. To find the position of an object, we need to consider the x and y coordinates on a plane. In simple terms, we need to draw two lines that meet each other in the middle (we call it origin), and make sure that the two lines are perpendicular to each other.
We start measuring the position of an object from the origin of that object on a plane. The origin of an object on an x-y plane is the point where it starts from zero, it is the reference point where the object is seen or observed. The vertical reference line is along the y-axis, while the horizontal reference line is along the x-axis. To find the position of an object, we need to understand whether it belongs to a space or a plane.
A Plane
A plane is due to two frames of reference, in form of an x and y-axis. We have two coordinates on a plane, the x-coordinate is known as abscissa, while the y-coordinate is known as ordinate. The horizontal component consists of the right and left sides starting from the origin. When you start from the origin and move to the left, it means that you are moving along the negative x direction. When you start from the origin of the horizontal plane and move to the right, it means that you are heading toward the positive direction of the x-axis.
We also have a vertical component of the plane called the y-axis. The origin intersects the y-axis at the middle making the upper side a positive side of the y-axis, and the lower side a negative y-axis. A plane is in the form of (x,y) coordinate.
A space
Space consists of a three-dimensional coordinate system. We can find the location of an object in space by considering (x,y,z) coordinates. We have three coordinates in space, and they are x, y, and z.
Position Time Graph
A position-time graph, often referred to as a distance-time graph, is a graphical representation that illustrates the relationship between an object’s position and the corresponding time elapsed. This type of graph provides a visual depiction of how an object’s location changes over a specific period, which makes it a valuable tool in the study of motion and kinematics.
In a position-time graph, the horizontal axis typically represents time, while the vertical axis represents the object’s position or distance from a reference point. As time progresses, the graph plots points that indicate the object’s position at various moments. By connecting these points with a continuous line, the graph offers insights into the object’s movement pattern.
Explanation of Position Time Graph
Different shapes of position-time graphs reveal distinct characteristics of an object’s motion. A straight horizontal line suggests that the object is stationary, maintaining a constant position over time. An upward-sloping line indicates uniform motion in one direction, where the object covers equal distances in equal time intervals. A steeper slope implies greater speed.
Curved lines on a position-time graph suggest non-uniform motion. A curve curving upward indicates acceleration, implying the object’s speed is increasing. Conversely, a downward-curving line signifies deceleration, implying a decrease in speed. Sharp peaks or valleys might suggest abrupt changes in position or sudden stops.
Position-time graphs are tools for understanding motion-related concepts, helping scientists, engineers, and students analyze and predict an object’s behaviour in various scenarios. They are fundamental in physics, enabling the calculation of velocities, accelerations, and other motion-related parameters. Additionally, these graphs aid in our daily applications such as designing transportation systems, predicting the trajectory of projectiles, and optimizing robotic movements. A position-time graph visually captures the complex interactions between an object’s position and time, which provides a comprehensive display of its motion. This graphical representation helps us in understanding kinematics.
How to Find Position in Physics
If we are to assume we are dealing with a plane in form of x-y coordinates. We write an ordered pair of values as (x,y). We measure values on a vertical line along the y direction, and we also measure values on the horizontal line which is the x direction. Assuming the position of an object is on a plane (5,7) as our values, here is how to find the position of the object on a plane.
- Starting from the origin (zero), count 5 units along the positive x-axis
- Additionally, count 3 units along the positive y-axis
- On the vertical line, draw a horizontal line starting from 7 units and move toward the right.
- on the horizontal axis, draw a line starting from 5 units and move upward until the two lines intersect
- Draw a line from both axis so that they meet at the center (5,7)
- Let’s assume we have A(x1,y1,z1) and B(x2,y2,z2)
- To calculate the distance between A and B
- We apply the formula AB2 = (x2 – x1 )2 + (y2 – y1 )2 + (z2 – z1 )2
- Additionally, to find the direction of AB. We apply [ tanθ=Opposite/Adjacent ]
Solved Problems: Position in Physics
Here are a few problems to guide you in finding an object’s position in a plane or a space.
Problem 1
Calculate the distance between two points M (5,6) and N (12,8) on a plane.
Solution:
Data
We can see that the coordinates belong to a plane in the form of (x,y).
Therefore, from the question: x1 = 5, x2 = 12, y1 = 6, y2 = 8
MN2 = (x2 – x1 )2 + (y2 – y1 )2
We now substitute our data into the above formula
MN2 = (12 – 5 )2 + (8 – 6 )2
After squaring the right-hand side, we have: MN2 = 72 + 22
which is equal to: MN2 = 49 + 4
Now, we sum up our right-hand side(RHS): MN2 = 53
By making MN the subject of the formula, and transferring the square to the RHS so that it can become the square root: MN = √53 = 7 units
our final answer is now: MN = 7 units
Problem 2
What is the distance between P(3,5,7) and Q(-4,4,-3)
Solution
Data
From the above question, we have our data as follows:
x1 = 3, x2 = -4, y1 = 5, y2 = 4, z1 = 7, z2 = -3
Now we apply the formula that says: PQ2 = (x2 – x1 )2 + (y2 – y1 )2 + (z2 – z1 )2
By substituting our values into the above equation, we now have: PQ2 = (-4 – 3)2 + (4 – 5)2 + (-3 – 7)2
This is now equal to: PQ2 = (-7)2 + (- 1)2 + (-10)2
Thus, it is now: PQ2 = 49 + 1 + 100
After summing the right-hand side, it becomes: PQ2 = 150
we now take the square root of both sides to eliminate the square on the left-hand side: √PQ2 = √150
This is now equal to: PQ = √150 = 12.3 units = 12 units
Problem 3
Two players were perfectly positioned at A(2,3,5) and B(6,0,8)
a. Determine the distances OA and OB if O is the point in a field represented by O(1,0,2)
b. Find the distance between the two players, BA
Solution
Data:
By extracting our data from the above question, we have
Point A(2,3,5) and B(6,0,8) with an origin O(1,0,2)
and if O(x1,y1,z1), A(x2,y2,z2) and A(x3,y3,z3)
Thus: x1 = 1, x2 = 2, and x3 = 6
y1 = 0, y2 = 3, and y3 = 0
z1 = 2, z2 = 5, and z3 = 8
a. To find OA, we apply the formula
OA2 = (x2 – x1 )2 + (y2 – y1 )2 + (z2 – z1 )2
By inserting our data into the above equation, we will have
OA2 = (2 – 1)2 + (3 – 0)2 + (5 – 2)2
This is equal to: OA2 = 12 + 32 + 32
After squaring the right-hand side(RHS), it becomes
OA2 = 1 + 9 + 9
We now add the values in the RHS and then apply the square root to both sides in order to make the OA subject of the formula
OA = √19 = 4.4 = 4 units
Therefore, the distance OA is 4 units or 4 meters
To calculate OB, we apply the same method for OA
Thus: OB2 = (x3 – x1 )2 + (y3 – y1 )2 + (z3 – z1 )2
after substituting our data into the above formula for calculating distance, we now have: OB2 = (6 – 1)2 + (0 – 0)2 + (8 – 2)2
This is equal to: OB2 = (5)2 + (0)2 + (6)2
we will now have: OB2 = 25 + 0 + 36
after summing up the RHS, we now have: OB2 = 61
by making OB the subject of the formula
OB = √61 = 7.8 = 8 units
Therefore, the distance between OB is 8 units or 8 meters.
b. To Find the distance between the two players A and B, we apply the formula
AB2 = (x2 – x3 )2 + (y2 – y3 )2 + (z2 – z3 )2
After substituting our data into the above equation, we will have: BA2 = (x3 – x2 )2 + (y3 – y2 )2 + (z3 – z2)2
Thus: BA = √[(6 – 2)2 + (0 – 3)2 + (8 – 5)2]
We now have: BA = √[(4)2 +(- 3)2 + (3)2]
it will now become: BA = √16 + 9 + 9
Thus: BA = √16 + 9 + 9 = √34 = 5.8 = 6 units
Therefore the distance between the two players, BA is 6 units or 6 meters
Examples of Position in Physics
Position forms the foundation for understanding the location of an object in space and time. Here are 10 examples of positions in physics:
- Displacement of a Car: The position of a car along a road, measured from a fixed starting point.
- Orbit of a Satellite: The position of a satellite in space, relative to a reference point on Earth’s surface.
- Pendulum’s Swing: The position of a pendulum bob at different points in its swing, relative to its resting position.
- Projectile Motion: The position of a projectile (like a baseball) at various points along its trajectory.
- Electron’s Location: The position of an electron in an atom, described by its distance from the nucleus.
- Elevator in a Building: The position of an elevator in a multi-story building, specified by the floor number.
- Position of Planets: The position of planets in our solar system, relative to their orbits around the Sun.
- Location of Particles in a Fluid: The position of particles in a fluid (like water molecules in a river), describing their distances from a reference point.
- Racecar on a Track: The position of a racecar on a racetrack, indicated by its distance along the track’s length.
- Position of a Climber: The position of a rock climber on a climbing wall, described by their height above the ground or floor.
These examples illustrate different ways in which position can be described in the context of physics, ranging from simple linear measurements to motion in different dimensions or systems.
Importance of Position in Kinematics
Position is important in kinematics. It allows us to describe an object’s motion accurately. Thus, by analyzing an object’s position over time, we can derive important parameters like displacement, velocity, and acceleration. These parameters provide us with insights into the object’s movement, speed, and changes in its motion.
What is a Reference Frame?
A reference frame, in physics, is a coordinate system used to describe the position, motion, and interactions of objects in space and time. It provides a framework for measuring and analyzing physical quantities such as position, velocity, acceleration, and forces. Reference frames play a key role in understanding the relative motion and interactions between objects and for formulating the laws of physics.
In simpler terms, you can think of a reference frame as an imaginary “camera” or viewpoint from which you observe and measure the positions and movements of objects. Different reference frames can yield different descriptions of the same events, especially when objects are in motion relative to each other. This concept is fundamental to understanding concepts like relativity and how observers in different states of motion might perceive the same event differently.
Coordinate Plane
A coordinate plane is a fundamental concept in mathematics, particularly in the field of geometry and algebra. It consists of two perpendicular number lines that intersect at a point called the origin. One line is horizontal and is called the x-axis, while the other is vertical and is called the y-axis.
The coordinate plane is used to represent points in a two-dimensional space. Each point on the plane is uniquely identified by a pair of numbers called coordinates. The first number in the pair represents the point’s position along the x-axis, and the second number represents its position along the y-axis.
Coordinates are usually written as (x, y), where ‘x’ is the horizontal coordinate and ‘y’ is the vertical coordinate. The origin is represented by the coordinates (0, 0), as it lies at the intersection of the x-axis and y-axis. The coordinate plane allows for precise visualization and analysis of geometric shapes, lines, curves, and their relationships. It is a foundation for graphing functions and equations. It aids in solving mathematical problems involving geometric figures, equations, and data analysis.
Relative Position and Reference Frames
The understanding position becomes more important when we consider reference frames. A reference frame is a coordinate system that allows us to define an object’s position. The concept of relative position comes into play when we analyze an object’s location concerning another object. Different reference frames can yield varying positions for the same object. It emphasizes the importance of choosing an appropriate frame for analysis.
Position in Different Fields of Physics
Astronomy and Astrophysics:
Position plays a key role in mapping celestial bodies in the whole of universe. Astronomers use precise coordinates to locate stars, galaxies, and other celestial objects. Accurate positions enable us to study the movements and interactions of these objects over time.
Fluid Dynamics:
In fluid dynamics, position helps us analyze the flow of fluids in various scenarios. The study of the behavior of fluids in pipelines helps in understanding the aerodynamics of aircraft and position. It aids in formulating accurate mathematical models.
Particle Physics:
Particle physicists have done deep research in the smallest constituents of matter and their interactions. The concept of position is important in particle detectors. It helps scientists pinpoint the trajectories and locations of subatomic particles during collisions.
You may also like to read:
SS1 Lesson Note: Introduction to Physics For First Term
Reference: