Explanation
Young’s modulus, also known as the Young modulus or the modulus of elasticity, is a fundamental concept in materials science and engineering. It quantifies the stiffness of a material and describes how it deforms under an applied force. Here is a step-by-step guide on how to calculate Young’s Modulus:
| Step | Description | Formula |
|---|---|---|
| 1 | Gather necessary data: | Length of the material (L) |
| Cross-sectional area (A) | ||
| Force applied (F) | ||
| Initial length (L₀) | ||
| 2 | Calculate stress (σ): | σ = F/A |
| 3 | Measure strain (ε): | ε = ΔL/L₀ |
| 4 | Calculate Young’s Modulus (E): | E = σ/ε or E = E = FL0 / AΔL |
Note:
- (ΔL) is the change in length.
- Young’s Modulus (E) is expressed in Pascals (Pa) or N/m².
Understanding Young’s modulus is very important for various applications, such as designing structures, analyzing material behaviour, and predicting the response of materials to different loads.
In this article, we will look into the steps involved in calculating Young’s modulus and provide you with a clear understanding of the methodology behind it. Whether you’re a student learning about materials science or an engineer working with different materials, this guide will help you master the calculation of Young’s modulus.
Methodology
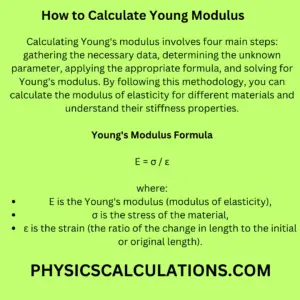
Calculating Young’s modulus involves a systematic approach, consisting of four key steps. Let’s delve into each step in detail.
Step 1: Data
The first step in calculating Young’s modulus is to gather the necessary data. You need to identify and collect the relevant information about the material and its behaviour under load. This includes the initial length of the material (L₀), the change in length (ΔL), and the applied force (F).
Step 2: Unknown
In this step, we determine the unknown parameter that we need to calculate. In the context of calculating Young’s modulus, the unknown is the stress (σ) experienced by the material.
Step 3: Formula
The Young’s modulus Formula is: E = σ / ε
where:
- E is the Young’s modulus (modulus of elasticity),
- σ is the stress of the material,
- ε is the strain (the ratio of the change in length to the initial or original length).
Step 4: Solution
Once we have the necessary data and the formula, we can substitute the values into the equation and solve for Young’s modulus. By rearranging the formula, we can solve for stress (σ) using the equation:
(Formula for stress σ = F / A)
where:
- F is the applied force, and
- A is the cross-sectional area of the material.
Additionally, ε is the strain which is the ratio of the change in length (ΔL)to the initial or original length (L0). Thus, the strain formula is;
ε = ΔL/L0
Substituting the calculated stress (σ) and the strain (ε) values into the formula for Young’s modulus, we can find the modulus of elasticity (E).
E = σ/ε = (F / A) / ΔL/L0 = FL0 / AΔL
Hence, the formula for calculating young modulus is: E = FL0 / AΔL
Solve Problems
Now, let us put our understanding of Young’s modulus calculation into practice by solving some problems.
Problem 1:
A steel rod with an initial length of 2 meters experiences a change in length of 0.02 meters when a force of 1000 newtons is applied. Calculate Young’s modulus of the steel rod.
Solution 1:
Given data:
- Initial length (L₀) = 2 meters
- Change in length (ΔL) = 0.02 meters
- Applied force (F) = 1000 newtons
To calculate the stress (σ), we use the formula:
σ = F / A
Since the cross-sectional area (A) of the rod is not available, we can assume it to be a circular cross-section. We can now calculate the area of a circle as:
A = πr2
We need to know the radius (r) of the rod to find the area. Let’s assume the radius to be 0.01 meters (or 1 centimetre).
Substituting the values into the equation for stress, we have:
σ = 1000 N / (3.142 x (0.01 m)2) ≈ 31830.99 N/m2
Now, let’s calculate the strain (ε) using the formula:
ε = ΔL / L₀ = 0.02 m / 2 m = 0.01
Finally, substituting the stress (σ) and strain (ε) into the formula for Young’s modulus, we get:
E = σ / ε = 31830.99 / 0.01 = 3,183,099 N/m2
Therefore, Young’s modulus of the steel rod is approximately 3.183099 x 106 N/m2.
Problem 2:
A rubber band with an initial length of 10 centimetres is stretched to a length of 12 centimetres by applying a force of 2 newtons. Calculate Young’s modulus of the rubber band.
Solution 2:
Given data:
- Initial length (L₀) = 10 centimeters = 0.1 meters
- Change in length (ΔL) = 12 centimeters – 10 centimeters = 0.02 meters
- Applied force (F) = 2 newtons
To calculate the stress (σ), we again need to determine the cross-sectional area (A) of the rubber band. Let’s assume the cross-section is rectangular, with a width (w) of 0.1 centimetres (or 0.001 meters) and a thickness (t) of 0.01 centimetres (or 0.0001 meters).
The area of the rectangular cross-section is given by:
A = w x t = 0.001 m x 0.0001 m = 0.0000001 m2
Substituting the values into the equation for stress, we have:
σ = 2 N / 0.0000001 m2 = 20,000,000 N/m2
Now, let us calculate the strain (ε):
ε = ΔL / L₀ = 0.02 m / 0.1 m = 0.2
Finally, substituting the stress (σ) and strain (ε) into the formula for Young’s modulus, we get:
E = σ / ε = (20,000,000 N/m2) / 0.2 = 100,000,000 N/m2
Therefore, Young’s modulus of the rubber band is 100,000,000 N/m2.
Summary
In summary, calculating Young’s modulus involves four main steps: gathering the necessary data, determining the unknown parameter, applying the appropriate formula, and solving for Young’s modulus. By following this methodology, you can calculate the modulus of elasticity for different materials and understand their stiffness properties.
Evaluation
The methodology provided in this article offers a clear and concise approach to calculating Young’s modulus. By breaking down the calculation into four distinct steps and providing examples of problem-solving, readers can easily grasp the concept and apply it to real-world scenarios. The use of mathematical expressions, formulas, and explanations ensures a comprehensive understanding of the topic.
Frequently Asked Questions
Q1: What is Young’s modulus used for?
Young’s modulus is used to quantify the stiffness of a material. It helps engineers and scientists understand how materials deform under stress and strain, enabling them to design structures and predict material behavior.
Q2: What are the units of Young’s modulus?
Young’s modulus is measured in pascals (Pa) or newtons per square meter (N/m^2). Other common units include gigapascals (GPa) and megapascals (MPa).
Q3: Can Young’s modulus be negative?
No, Young’s modulus cannot be negative. It is always a positive value since
it represents the ratio of stress to strain, both of which are non-negative quantities.
Q4: Is Young’s modulus the same for all materials?
No, Young’s modulus varies across different materials. Each material has its own unique modulus of elasticity, which depends on its composition and atomic structure.
Q5: What is the significance of a higher Young’s modulus?
A higher Young’s modulus indicates a stiffer material that requires a greater force to produce a given amount of deformation. Materials with high Young’s modulus are generally more rigid and less prone to elastic deformation.
Q6: How does temperature affect Young’s modulus?
Temperature can affect Young’s modulus by altering the atomic vibrations and bonding in a material. In general, an increase in temperature leads to a decrease in Young’s modulus, as the thermal energy disrupts the material’s structure.
Assignment
Now it’s time for you to apply what you’ve learned. Try solving the following problems and calculate Young’s modulus for each case. Don’t forget to include units in your answers.
- A copper wire with an initial length of 50 centimetres experiences an elongation of 0.5 millimetres when a force of 10 newtons is applied. Find Young’s modulus of the copper wire.
- A wooden beam with an initial length of 3 meters undergoes a compression of 2 centimetres when a force of 1000 newtons is applied. Find Young’s modulus of the wooden beam.
- A plastic ruler with an initial length of 30 centimetres stretches to a length of 30.5 centimetres when a force of 2 newtons is applied. Find Young’s modulus of the plastic ruler.
- An aluminium rod with an initial length of 2 meters is stretched to a length of 2.1 meters by applying a force of 5000 newtons. Determine Young’s modulus of the aluminium rod.
- A rubber ball with an initial diameter of 10 centimetres is compressed to a diameter of 9.5 centimetres when a force of 5 newtons is applied. Determine Young’s modulus of the rubber ball.
Answers:
- Copper wire: Young’s modulus ≈ [to be calculated]
- Wooden beam: Young’s modulus ≈ [to be calculated]
- Plastic ruler: Young’s modulus ≈ [to be calculated]
- Aluminium rod: Young’s modulus ≈ [to be calculated]
- Rubber ball: Young’s modulus ≈ [to be calculated]
By solving these problems, you will gain further practice and enhance your understanding of calculating Young’s modulus for different materials.
Conclusion
In conclusion, Young’s modulus is a fundamental concept in materials science that allows us to quantify the stiffness of materials. By following the four-step methodology described in this article, you can accurately calculate Young’s modulus and apply it to various engineering and scientific applications. Understanding the relationship between stress, strain, and Young’s modulus empowers us to analyze and predict the behaviour of materials under different conditions, leading to improved designs and better material selection.
You may also like to read: