What is Work Function?
The work function of a metal is the minimum amount of energy that is needed to eject an electron from the surface of a metal. The unit of work function electronvolt (eV) or Joules(J). The symbol for the work function is Φ or W.
Video Explanation: How to Calculate the Work Function
Watch the video below for more explanation and a few solved problems:
Work Function Formula and Video Explanation
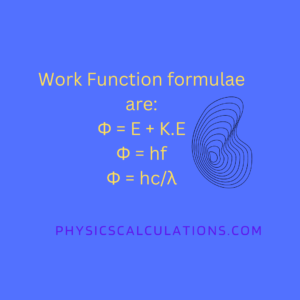
The formula for calculating the work function are:
- The first formula for calculating work function is Φ = E + K.E [where Φ = work function, E = total energy, and K.E = Maximum kinetic energy of liberated photoelectrons]
- We also have Φ = hf0 [where w = work function of the metal, h=Planck’s constant, and f0=Threshold frequency]
- The third formula for work function is Φ = hc/λ [where h=planck’s constant, c= speed of light in the vacuum, and λ=wavelength of the radiation]. This is because f=c/λ, and since Φ = hf, this implies that Φ = hc/λ
Photoelectric Equation
To comprehend the concept of work function better, We need to look into photoelectric effect. The photoelectric effect occurs when light, typically in the form of photons, strikes the surface of a material and causes the ejection of electrons.
Definition of Photoelectric Effect: The photoelectric effect is due to the emission of electrons from the surface of a metal when light is shown on it. We can write the photoelectric equation as
E = hf = Φ + (1/2)mv2
Where
E = energy required to liberate an electron from the surface of a metal
h = Planck’s constant
f = This is the frequency
Φ = This symbol represents the work function
m = mass of an electron
How to Calculate the Work Function of a Metal
Calculating the Work Function
To calculate the work function, you need to follow a step-by-step process:
Step 1: Determine the Energy of the Incident Photon
The energy of a photon is given by the equation E = hf, where E represents energy, h is Planck’s constant (approximately 6.626 x 10-34 J·s), and f denotes the frequency of the photon.
Step 2: Calculate the Kinetic Energy of the Ejected Electron
The kinetic energy (KE) of the ejected electron can be determined using the equation KE = hf – φ, where φ is the work function of the material.
Step 3: Calculate the Work Function
Rearranging the equation from Step 2, we can determine the work function as φ = hf – KE.
Applicable Methods of Finding Work Function of a Metal
Method 1: Calculating the Work Function of a Metal Surface
Suppose we have a metal surface that is struck by a photon with a frequency of 2.5 x 1014 Hz. The kinetic energy of the ejected electron is measured as 4.0 x 10-19 J. By substituting the given values into the equation φ = hf – KE, we can calculate the work function.
φ = hf – KE = (6.626 x 10-34 x 2.5 x 1014) – 4.0 x 10-19 = -2.3435 x 10-19
Example 2: Calculating the Work Function of a Semiconductor
In the case of a semiconductor material, the process of calculating the work function is similar to that of a metal surface. However, semiconductors exhibit unique characteristics due to their energy band structure, which affects electron behavior.
Solved Problems: How to Calculate the Work Function of a Metal
Here are a few examples to help you understand how to calculate the work function of a metal.
Problem 1
A metal is illuminated with radiation of energy 6.88eV. If the kinetic energy of the emitted electrons is 1.5eV. Calculate the work function of the metal.

Text Solution
Data:
The work function, Φ = Total energy + Kinetic energy
and the the incident radiation = total energy = 6.8eV
The kinetic energy of the emitted electron = 1.5eV
and the formula is Φ = E + K.E
Thus,
Work function, Φ = 6.8eV + 1.5eV = 8.4eV
Problem 2
A metal was exposed to photons at a frequency of 2.0 x 1015Hz, and electrons were emitted with a maximum kinetic energy of 4.00 x 10-19 J. Calculate the work function, of this metal.

Text Solution:
Data:
Φ = hf + K.E
and
Frequency of photons, f = 2.0 x 1015Hz
The maximum kinetic energy of the electron, K.E = 4.00 x 10-19 J
planck’s constant, h = 6.6×10-34Js
Hence, we can now apply the formula for the work function that says Φ = hf + K.E
Thus, we now have Φ = (6.6×10-34) x (2.0 x 1015) + (4.00 x 10-19)
This is equal to
Φ = (6.6×10-34) x (2.0 x 1015) + (4.00 x 10-19)
we now have
Φ = 1.32×10-18 + 4.00 x 10-19
Therefore, the work function Φ, of the metal is 1.72 x 10-18 J
Problem 3
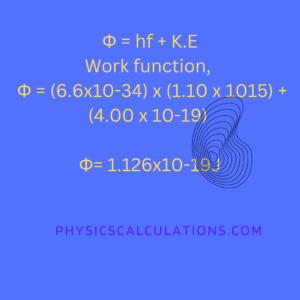
When metal was exposed to photons at a frequency of 1.10 x 1015Hz, electrons were emitted with a maximum kinetic energy of 4.00 x 10-19J. Calculate the work function of the metal.
Text Solution
Data:
frequency, f = 1.10 x 1015Hz
Maximum kinetic energy, K.E = 4.00 x 10-19J
The work function of the metal, Φ =?
Planck’s constant, h = 6.6×10-34Js
Therefore, by applying the formula for the work function that says Φ = hf + K.E
After substituting our data into the above formula for the work function, we will now have
Φ = (6.6×10-34) x (1.10 x 1015) + (4.00 x 10-19)
After multiplying (6.6×10-34) by (1.10 x 1015) the above expression will give us
Φ = (7.26×10-19) + (4.00 x 10-19)
We will now add (4.00 x 10-19) to (7.26×10-19), Which implies that the above expression is now
The work function of the metal, Φ = 1.126×10-19J
Therefore, the work function of the metal Φ is 1.126×10-19J
Problem 4
Calculate the work function of a metal in eV, if its threshold wavelength is 6,800Å [Take h = 6.6 x 10-27 ergs].

Text Solution
Data:
The wavelength, λ = 6,800Å
and from our knowledge of prefixes, the symbol Å is called angstrom, and 1Å = 0.0000000001 or 10-10m
Thus, the wavelength, λ = 6,800Å = 6,800 x 10-10m = 6.86 x 10-6m
Therefore, λ = 6.86 x 10-6m
From the above question, we were told that Planck’s constant, h = 6.6 x 10-27 ergs = 6.6 x 10-27 x 10-7 [This is because 1 erg is equal to 10-7J]
Therefore we can now write Planck’s constant, h = 6.6 x 10-27 x 10-7 = 6.6 x 10-27-7 = 6.6 x 10-34Js
Solution (applying data and the work function formula)
We will apply the formula for the work function, which is Φ = hc/λ [where h=planck’s constant, c= speed of light in the vacuum, and λ=wavelength of the radiation]
Speed of light in the vacuum, c = 3 x 108 m/s
After substituting our values into the above formula, we will have
Φ = (6.6 x 10-34 x 3 x 108)/6.86 x 10-6
We multiply the numerator (6.6 x 10-34 x 3 x 108) to obtain 1.98 x 10-25
Thus,
Work function, Φ = 1.98 x 10-25/6.86 x 10-6
Dividing 1.98 x 10-25 by 6.86 x 10-6 will give us 2.886 x 10-20
Work function, Φ = 2.886 x 10-20J
Which explains that the work function of the metal is 2.886 x 10-20J
To convert our answer into electronvolt, we consider 1eV = 1.6 x 10-19J
Hence, Work function, Φ = (2.886 x 10-20J x 1eV) / 1.6 x 10-19J = 0.179125eV
Therefore, our work function in electronvolt, Φ = 0.179125eV or approximately into 0.2 eV
More Videos: Work Function
Work Function
Photoelectric Effect
Drop a comment on what you don’t understand so that we can help you with more resources.
You may also like to read:
How to Calculate Threshold Frequency
How to Calculate Power in a Circuit
Reference