How to Calculate the Relative Density of a Liquid

What is Relative Density?

Definition and Concept of Relative Density: Relative density, also known as specific gravity, is a dimensionless quantity that describes the ratio of the density of a liquid to the density of a reference substance. It is a measure of how heavy a liquid is compared to an equal volume of a chosen reference material. Watch the video below for more clarification.

Relative Density of Liquid Formula

How to Calculate the Relative Density of a Liquid

The three relative density formula are as follows:

Relative Density (RD) of Liquid = U_L / U_W
RD = V_L / V_W
Relative of liquid (RD) = A_L / A_W

Where:

RD = Relative density

U_L = Upthrust of object in liquid

V_L = Volume of liquid displaced by object

A_L = Apparent loss of weight of a solid in liquid

U_W = Upthrust of object in water

V_W = Volume of water displaced by object

A_W = Apparent loss of weight of same solid in water

We applied the relative density formula above to solve example 5 and 6

How to Calculate Relative Density

Relative Density of Solids vs Relative Density of Liquids

Here is an expanded table with materials and their relative densities (liquid and solids):

Material	State	Relative Density (g/cm³)
Iron	Solid	7.87
Lead	Solid	11.34
Gold	Solid	19.32
Copper	Solid	8.96
Aluminum	Solid	2.70
Silver	Solid	10.49
Diamond	Solid	3.52 – 3.53
Ice	Solid	0.92
Granite	Solid	2.63 – 2.75
Wood (Oak)	Solid	0.6 – 0.9
Water	Liquid	1.00
Olive Oil	Liquid	0.91
Mercury	Liquid	13.59
Ethanol	Liquid	0.789
Milk	Liquid	1.02 – 1.05
Honey	Liquid	1.36 – 1.45
Vegetable Oil	Liquid	0.91 – 0.93
Glycerol	Liquid	1.26

You need to keep in mind that these values are approximate and can vary depending on various factors.

Explanation of How to Calculate the Relative Density of a Liquid

I will now walk you through two steps you can apply to calculate the relative density of a liquid

Step 1

I will use an empty dry bottle to explain in simple terms, how to calculate the relative density of liquids. Now, let us get started:

Get a dry empty bottle, record it is mass and name it M₁

Add liquid (for example oil) to the bottle and name it M₂. Now, mass of the liquid is added to the mass of the mass of the empty bottle and it is M₂.

The next step is to remove the liquid from the bottle.

Wash and dry the bottle again

Add water to the bottle, and record their total mass together. Tag this mass M₃

To Find only the mass of the liquid without the bottle, subtract the Mass of the empty bottle (M₁) from the Mass of the bottle and the liquid (M₂) which is

Mass of liquid only = M₂ – M₁

Additionally, you need to apply the following methods to find the Mass of an equal volume of water:

subtract the Mass of the empty bottle (M1) from the Mass of the bottle and that of the water together (M₃) which is:

Mass of equal volume of water = M₃ – M₁

Therefore, we can now say that the formula for how calculating the relative density of a liquid is

Relative density of liquid = Mass of the liquid / Mass of equal volume of water

which is

Relative density of liquid = (M₂ – M₁) / (M₃ – M₁)

You may also like to read:

Derivation of Bernoulli’s Equation

Step 2

In this step, I will apply Archimedes’ principle to explain how to calculate the relative density of liquid:

Attach a metal object to spring balance and suspend it in air. Record the mass of the object in air as M₁.

Immerse the metal object (that is already attached to the spring balance) in liquid, and record the apparent mass of liquid as M₂

Clean and dry the metal object

Immerse the object in the water and record the apparent mass of water as M₃

We will now find the weight of the liquid that is being displaced by the metal object, which is the upthrust in liquid.

Now, the upthrust in liquids is calculated by subtracting mass of the object in air as M₁ from apparent mass of liquid as M₂. This implies that Upthrust in liquids = (M₂ – M₁)

Upthrust in water is the same thing as the weight of the water displaced by the object

To find upthrust in water, we subtract apparent mass of water (M₃) from mass of the object in air (M₁). Therefore, Upthrust in water = M₁ – M₃

Hence, to calculate Relative density, we say that

Relative density = [ Upthrust in liquids = M₂ – M₁ ] / [Upthrust in water = M₁ – M₃]
Relative density = (M₂ – M₁) / (M₁ – M₃)

Solved Problems

Remember to follow the following steps in order to solve your examples at ease:

Underline all the figures you see in the question
Read the question, and extract your data
Underline the statement where you see the question and write the formula you need to apply
Check all the elements in the formula and assign their figures to them
Solve the problem by making the element in question the subject of the formula
Don’t forget to always include the s.i unit in your final answer

Problem 1

A body weighs 0.30N in air, 0.25N when fully immersed in water, and 0.27N when fully immersed in a liquid. Calculate (a) it is loss of weight in water, (b) the relative density, and (c) The relative density of the liquid

Solution:

(a) To calculate the loss of weight in water, we subtract the weight of the body when fully immersed in water (0.25N) from the weight of the body in the air (0.30N).

This implies that

Loss of weight in air = 0.30 – 0.25 = 0.05N

Therefore,

Loss of weight in air = Upthrust in water = Weight of equal volume of water displaced by the body = 0.05N

(b) Relative density of the body = Weight of the body in the air (0.30) / Weight of equal volume in water (0.05)

Now, we can see that

Relative density of the body = 0.30 / 0.05 = 6

This is because

Upthrust in liquid = mass of the body in air – mass of the body in liquid

and Upthrust in water = mass of the body in air – mass of the body in water

Thus,

Relative Density of the liquid = (0.30 – 0.27) / (0.30 – 0.25)

Therefore, the Relative density of liquid = 0.03 / 0.05 = 0.6

Now we can see that the Relative density of the liquid is 0.6

Problem 2

A relative density bottle weighs 20 grams when empty, 80 grams when filled with water, and 100 grams when filled with a liquid. Find the relative density of the liquid.

Solution:

Data

Weight of the bottle in the air, W₁ = 20 grams

Also, the weight of the bottle with water, W₂ = 80 grams

Additionally, the weight of the bottle with liquid, W₃ = 100 grams

Applying the formula

Relative density = (W₃ – W₁) / (W₂ – W₁)

Thus, we have

Relative density = (100 – 20) / (80 – 20) = 80 / 60 = 1.33

Problem 3

A density bottle has a mass of 0.05 kilogram when empty, a mass of 0.30 when some quantity of steel ball bearings is added to it, and a mass of 0.35 kilogram when the remainder of the bottle is filled with water. if the density of the bottle weighs 0.2 kilograms when filled with water. Calculate the relative density of the steel ball bearing.

Solution:

Data

Mass of an empty bottle, M₁ = 0.05 kg

Also, the Mass of the bottle with steel ball bearings, M₂ = 0.30 kg

Mass of the bottle, steel ball bearings, and water, M₃ = 0.35

we can also see that the Mass of the bottle with water, M₄ = 0.2 kg

The Relative density of the steel (R.D) = ?

Now, let us apply the formula relative density (r.d) to solve the above problem

Mass of the steel ball bearing M₅ = M₂ – M₁ = 0.30 – 0.05 = 0.25 kg

We can also see that

Mass of water and the bottle M₆= M₄ – M₁ = 0.2 – 0.05 = 0.15 kg

Additionally, mass of water, the bottle, and the steel ball bearing M₇= M₃ – M_{2 =}0.35 – 0.30 = 0.05 kg

We finally calculate the mass of water having the same volume as the ball bearing M₈ = M₆ – M₇ = 0.15 – 0.05 = 0.10

Therefore to find the relative density of steel ball bearings, we say that

Relative density (R.d) = M₅ / M₈ = 0.25 / 0.10 = 2.5

Problem 4

The mass of a bottle is 18 grams when it is empty, 44.0 grams when it is filled with water, and 39.84 gram when it is full of a second liquid. Calculate the density of the liquid.

Solution:

Data:

Mass of an Empty bottle M₁= 18.0 g

Also,

Mass of the bottle and that of water M₂ = 44.0 g

Therefore, we have mass of water, M₃ = M₂ – M₁ = 44.0 – 18.0 = 26 g

Also, the mass of the bottle and the liquid M₄ = 39.84 g

Now, mass of liquid, M₅ = M₄ – M₁ = 39.84-18.00 = 21.84 g

The relative density of the liquid = mass of liquid / mass of an equal volume of water = M₅ / M₃

R.d = 21.84/26 = 0.84

The density of the liquid = 0.84 g/cm³

Problem 5

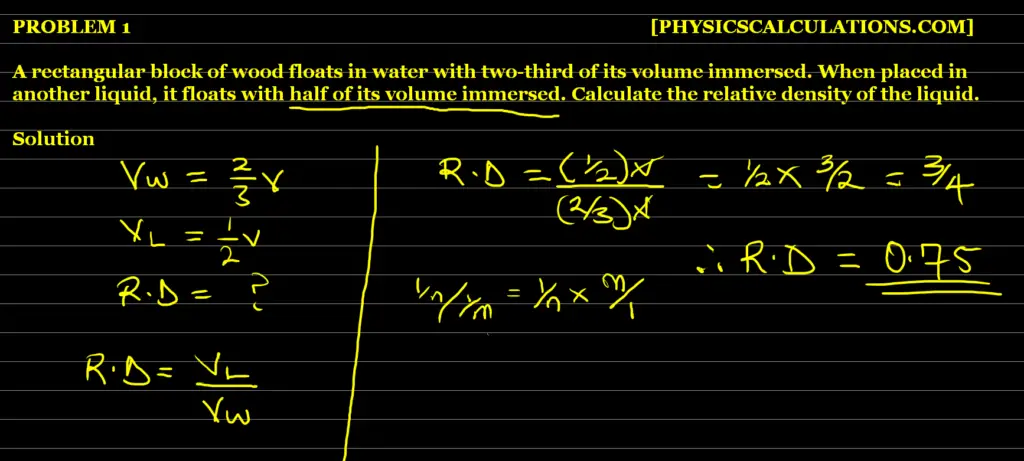
Problem 6

Note:

Relative density is simply comparing the density of substances (solid, liquid, or gaseous) to that of water (1 g/cm3). It does not have a unit and may be measured with a hydrometer. We use the symbol R.D to indicate relative density, and it’s a scalar quantity

Method 2: How to Calculate the Relative Density of a Liquid

To calculate the relative density of a liquid, here is another technique you apply to solve a problem:

Gather the Necessary Information: Before you begin, ensure you have the density of the liquid you want to measure and the density of water at a specific temperature. These values are typically provided in kilograms per cubic meter (kg/m³) or grams per cubic centimeter (g/cm³).
Identify the Formula: The formula to calculate relative density is: Relative Density = (Density of Liquid) / (Density of Water)
Plug in the Values: Substitute the known values into the formula. Make sure the units are consistent to get an accurate result.
Perform the Calculation: Use a calculator to divide the density of the liquid by the density of water. The result will be a dimensionless quantity, as both densities have the same units.
Interpret the Result: The calculated relative density will provide you with valuable information about the liquid’s density compared to water. If the relative density is less than 1, the liquid is less dense than water, and if it’s greater than 1, the liquid is denser.

Method 3: Measurement and Calculation of Relative Density

A. Basic Formula: Relative Density = Density of Liquid / Density of Reference Substance The relative density is calculated by dividing the density of the liquid under consideration by the density of the chosen reference substance.

B. Choice of Reference Substance The selection of an appropriate reference substance is critical. Common choices include water, air, or a specific standardized solution, depending on the nature of the liquid being studied.

C. Experimental Techniques for Determining Relative Density Several techniques are employed to determine the relative density of liquids:

Hydrometer Method: This involves using a hydrometer, a device that measures the buoyancy of a liquid. The depth to which the hydrometer sinks is related to the relative density of the liquid.
Pycnometer Method: A pycnometer is a container specifically designed to hold a known volume of liquid. By measuring the mass of the liquid it contains, and comparing it to an empty pycnometer, the relative density can be calculated.
Buoyancy Method: Archimedes’ principle is applied in this method, where the buoyant force exerted on an immersed object is used to determine the relative density of the liquid.

Importance of Relative Density in Various Applications

The concept of relative density finds application across different fields. From ensuring the quality of beverages and identifying unknown liquids to aiding engineers in selecting appropriate fluids for various processes, relative density is a cornerstone in understanding the behaviour and characteristics of liquids.

Relation to Density and Specific Gravity

While density measures the mass of a substance per unit volume, specific gravity is a dimensionless quantity that compares the density of a substance to the density of water at a specified temperature. Relative density and specific gravity are closely related, with the latter often being used interchangeably to express the former.

Factors Affecting Relative Density

A. Temperature Effects Temperature variations can influence the relative density of liquids due to changes in volume and density. Accurate measurements must account for temperature fluctuations.

B. Pressure Effects Pressure changes can also impact relative density, particularly in gases and compressible liquids. Corrections are necessary when working under non-standard pressure conditions.

C. Impurities and Composition of the Liquid Impurities and variations in composition can affect the relative density, making it important to consider the purity of the liquid under examination.

Applications of Relative Density

A. Quality Control in Industries Relative density is employed in industries such as food and beverages, where it aids in monitoring product consistency and detecting adulteration.

B. Identification of Liquids In forensic analysis and material identification, relative density can be a valuable tool for determining the nature of unknown liquids.

C. Selection of Fluids in Engineering Engineers rely on relative density to choose the appropriate liquids for hydraulic systems, cooling processes, and other engineering applications.

D. Determination of Concentration in Solutions Relative density assists in determining the concentration of solutes in solutions, such as in the pharmaceutical and chemical industries.

E. Monitoring Changes in Mixtures and Blends Relative density is used to track changes in mixtures and blends, aiding in quality assurance during production processes.

Comparison with Other Density-related Concepts

A. Absolute Density While relative density compares a liquid’s density to that of a reference substance, absolute density stands alone as the density of the substance itself.

B. Specific Gravity Specific gravity is essentially synonymous with relative density, often used in industrial contexts and practical applications.

C. Bulk Density Bulk density considers the density of a substance in bulk, accounting for voids and air spaces between particles.

Importance in Daily Life and Industry

A. Beverages and Food Industry Relative density ensures the consistency and quality of beverages and food products, preventing consumer health risks and ensuring accurate labeling.

B. Petroleum and Petrochemical Industry In the oil and gas sector, relative density is pivotal in classifying and evaluating petroleum products.

C. Pharmaceutical and Medical Applications Pharmaceutical companies rely on relative density for drug formulation and dosage determination.

D. Environmental Monitoring Relative density aids environmental scientists in analyzing pollutants, tracking water quality, and assessing ecosystem health.

Challenges and Limitations in Measuring Relative Density

A. Accuracy and Precision Accurate measurement of relative density requires advanced equipment and careful techniques to minimize errors.

B. Impact of Viscosity Viscous liquids may pose challenges, as their flow characteristics can affect measurement accuracy.

C. Compatibility with Different Reference Substances The choice of reference substance must be appropriate for the liquid under investigation.

Examples and Case Studies

A. Determination of Alcohol Content in Beverages Relative density is utilized to determine the alcohol content in alcoholic beverages, contributing to regulatory compliance and consumer safety.

B. Fuel Quality Testing in Automobile Industry The automotive sector employs relative density to assess fuel quality and efficiency.

C. Identification of Unknown Liquids in Forensics Forensic scientists use relative density to identify liquids found at crime scenes, aiding in criminal investigations.

Future Trends and Developments in Relative Density Measurement

A. Advances in Measurement Techniques Continued advancements in technology will likely lead to more precise and efficient methods for measuring relative density.

B. Integration with Automation and Technology Automation and digitalization will streamline data collection and analysis, enhancing accuracy and reducing human error.

FAQs about Relative Density of a Liquid

Is relative density the same as density?

No, relative density and density are not the same. Density is an absolute measure of how much mass is contained in a given volume, while relative density compares the density of a substance to the density of another substance, typically water.

Can relative density be less than 1?

Yes, a substance’s relative density can be less than 1. This means that the substance is less dense than water and will float on its surface.

How is relative density measured?

Relative density is measured using specialized instruments called hydrometers or densimeters. These devices are designed to measure the density of a liquid and compare it to the density of water.

Why is water used as the reference substance for relative density?

Water is commonly used as the reference substance for relative density because of its widespread availability and consistent properties. Additionally, water has a well-defined density of 1.0 g/cm³ at a specific temperature, making it a convenient reference point.

What is the importance of relative density in the beverage industry?

In the beverage industry, relative density is crucial for quality control and consistency. It helps ensure that beverages are properly concentrated and mixed, resulting in the desired taste and experience for consumers.

How does relative density affect sea life?

Relative density plays a role in the movement of marine organisms. Some species have specific adaptations that allow them to control their buoyancy by adjusting their internal gas volume, helping them rise or sink in the water.

Reference

First
Smith, J. D. (2008). Introduction to chemical engineering thermodynamics. McGraw-Hill.
Perry, R. H., Green, D. W., & Maloney, J. O. (1984). Perry’s Chemical Engineers’ Handbook (6th ed.). McGraw-Hill.
ASTM International. (2017). ASTM E100-17: Standard Specification for ASTM Hydrometers. West Conshohocken, PA.
Callister, W. D., & Rethwisch, D. G. (2017). Materials Science and Engineering: An Introduction (10th ed.). John Wiley & Sons.