What is Buoyant Force?
Buoyant force is the upward force exerted by a fluid—such as water or air—on an object immersed or floating in it. This force opposes the weight of the object and is a result of the pressure difference between the top and bottom of the submerged or floating object. The greater the volume of the displaced fluid, the greater the buoyant force.
This force is described by Archimedes’ principle, which states that an object submerged in a fluid experiences an upward force equal to the weight of the fluid it displaces. If the buoyant force is greater than the weight of the object, it will float; if it is less, the object will sink. The buoyant force plays a key role in the behaviour of objects in fluids, explaining phenomena such as why ships float and balloons rise. Buoyancy is a fundamental concept in fluid mechanics and is essential for understanding the equilibrium of objects in liquids and gases.
How to Calculate Buoyant Force: Explained
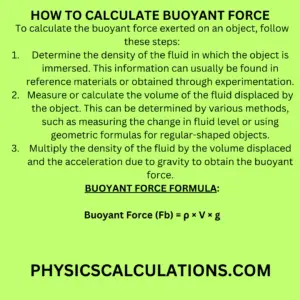
To calculate the buoyant force acting on an object submerged or floating in a fluid, follow these steps:
- Determine the Fluid Density (ρ): Identify the density of the fluid in which the object is immersed. This is typically denoted as (\rho) and is measured in kilograms per cubic meter (kg/m³).
- Determine the Volume of the Displaced Fluid (V): Find the volume of the fluid displaced by the object. This can be the volume of the object itself if it’s fully submerged or the volume of the part of the object immersed in the fluid.
- Use Archimedes’ Principle: Apply Archimedes’ principle, which states that the buoyant force (Fb) is equal to the weight of the fluid displaced:
[ Fb = ρ ⋅ g ⋅ V ]
where (ρ) is the fluid density, (g) is the acceleration due to gravity, and (V) is the volume of the displaced fluid. - Consideration of Fluid Properties: Ensure consistency in units; for example, if the density is in kg/m³, the volume should be in cubic meters.
- Understand Object Behavior: Recognize that if the buoyant force is greater than the weight of the object, the object will float; if it is less, the object will sink.
Calculating buoyant force is essential in understanding the stability and behaviour of objects in fluids, providing insights into phenomena such as floating and sinking.
Understanding Buoyancy
Buoyant force is a fundamental concept in physics that explains the upward force exerted by a fluid on an object immersed in it. This force is a result of the pressure difference between the top and bottom of the object in the fluid. The greater the volume of the displaced fluid, the greater the buoyant force.
In real life, buoyant force is responsible for various phenomena, notably the ability of objects to float. One common example is a ship floating on water. The ship’s hull displaces a volume of water equal to its weight, and the buoyant force acts upward, countering the force of gravity, allowing the ship to stay afloat.
Another example is a helium balloon. The helium inside the balloon is lighter than the air around it, creating a buoyant force that lifts the balloon against the force of gravity. Similarly, a swimmer floats in a pool due to the buoyant force exerted by the water on their body.
Understanding buoyant force is key for designing submarines, boats, and other vessels that navigate water. It also explains why certain objects float or sink and is a key principle in the construction of life jackets, providing buoyancy to keep individuals afloat in water.
Buoyant Force Formula
The equation for calculating buoyant force (buoyant force formula) is relatively straightforward. It can be expressed mathematically as follows:
Buoyant Force (Fb) = ρ × V × g
Where:
- Fb represents the buoyant force in Newtons (N).
- ρ denotes the density of the fluid in kilograms per cubic meter (kg/m³).
- V is the volume of the fluid displaced by the object in cubic meters (m³).
- g represents the acceleration due to gravity, approximately 9.8 m/s².
Example Calculation: Buoyant Force of a Submerged Object
Let us solve a problem to illustrate the process of determining the buoyant force. Suppose we have a solid iron sphere with a density of 7,800 kg/m³ submerged in water.
Step 1: Density of Water
The density of water is approximately 1,000 kg/m³.
Step 2: Volume Displaced
For a sphere, you can calculate the volume by using the formula: V = (4/3) × π × r³.
Let’s assume the radius of the sphere is 0.5 meters. Plugging this value into the formula, we find: V = (4/3) × 3.14 × (0.5)³ = 0.524 m³.
Step 3: Calculation
Now, we can calculate the buoyant force using the formula: Fb = ρ × V × g.
Substituting the values, we get: Fb = 1,000 kg/m³ × 0.523 m³ × 9.8 m/s² = 5,131.93 N.
Therefore, the buoyant force exerted on the iron sphere submerged in water is approximately 5132 Newtons.
Frequently Asked Questions (FAQs)
- What is the formula for calculating density?
- A: We calculate Density by dividing the mass of an object by its volume. The formula is Density (ρ) = Mass (m) / Volume (V).
2. Can the buoyant force ever be greater than the weight of the submerged object?
- A: No, the buoyant force can never exceed the weight of the object. If it did, the object would accelerate upwards and eventually escape the fluid.
3. Are there any real-world applications of buoyancy calculations?
- A: Absolutely! Buoyancy calculations are essential in various fields, including shipbuilding, aerospace engineering, and even everyday activities like swimming and hot air ballooning.
4. Does the shape of the object affect the buoyant force?
- A: Yes, the shape of an object plays a role in determining the buoyant force. Objects with larger volumes displace more fluid, resulting in a greater buoyant force.
5. Can you provide an example where buoyancy is not applicable?
- A: Buoyancy is not applicable in a vacuum or when dealing with gases, as they do not exert a buoyant force on objects.
6. How does the buoyant force change with the depth of immersion?
- A: The buoyant force remains constant regardless of the depth of immersion, as long as the volume of fluid displaced remains the same.
Conclusion
In conclusion, understanding how to calculate buoyant force is crucial for comprehending the principles of buoyancy. By following the simple equation and step-by-step process outlined in this article, you can determine the upward force exerted on an object submerged in a fluid.
Whether you are learning the physics of floating objects or applying buoyancy calculations to practical engineering problems, this knowledge will prove invaluable. So go ahead, apply your newfound expertise, and unlock the mysteries of buoyancy!
You may also like to read: