Introduction
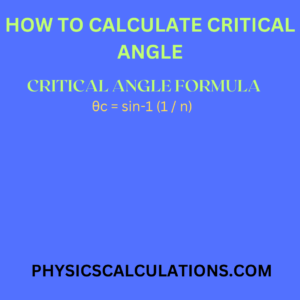
To understand how to calculate critical angle, we use any of the two formulas below:
C = sin-1 (1 / n)
or C = sin-1 (na / ng)
Where
C = critical angle
n = refractive index of the medium
Also
na = refractive index of air
and
ng = refractive index of glass
You may also like to read:
What is Critical Angle and its Formula?
And what is the critical angle for light traveling from crown glass (n = 1.52) into water (n = 1.33)?
Problem 1
If the refractive indices of glass and water are 1.5 and 1.3 respectively. What will be the critical angle when the angle of refraction in the water medium is 90 degrees?
Data
Refractive index of glass, ng = 1.5
The refractive index of water, nw = 1.3
Angle of refraction in the water medium, θw = 900
Unknown
Critical angle, C = ?
Formula
We will apply the formula
C = sin-1 (nw sinθw / ng)
Solution
We can now apply our data into the formula to obtain
C = sin-1 (nw sinθw / ng) = sin-1 (1.3 x sin900 / 1.5)
The above expression will become
C = sin-1 (1.3 x 1 / 1.5)
We will now have
C = = sin-1 0.9 = 640
Therefore, the critical angle is 64 degrees.
Problem 2
What is the critical angle for a light ray traveling in water with a refractive index of 1.33 that is incident on the surface of the water above which air with a refractive index of 1.00? Answer to the nearest degree.
Data
Refractive index of water, nw = 1.33
The refractive index of air, na = 1.00
Unknown
Critical angle, C = ?
Formula
We will apply the formula below to solve the problem
C = sin-1 (na / nw)
Solution
We will insert our data into the formula to get
C = sin-1 (na / nw) = sin-1 (1 / 1.33)
After dividing 1 by 1.33 we will obtain
C = sin-1 0.75 = 48.60 = 490
Therefore, the critical angle for the light ray is 49 degrees.
Problem 3
Light rays travel through a layer of kerosene floating on the surface of water that has a refractive index of 1.33. Light rays that are incident on the interface of kerosene and water at angles of 16.9° from the surface or less are totally internally reflected. What is the refractive index of the kerosene? Give your answer to two decimal places.
Data
The refractive index of water, nw = 1.33
Critical angle, C = 900 – 16.90 = 73.10
Unknown
The refractive index of the kerosene, nk = ?
Formula
We will use the formula
nk = nw / sinC
Solution
Insert your data into the above formula to obtain
nk = nw / sinC = 1.33 / sin73.10
We will now have
nk = 1.33 / 0.96 = 1.385 = 1.39
Therefore, the refractive index of the kerosene, nk is 1.39 in two decimal places.