What is Heisenberg’s Uncertainty Principle?
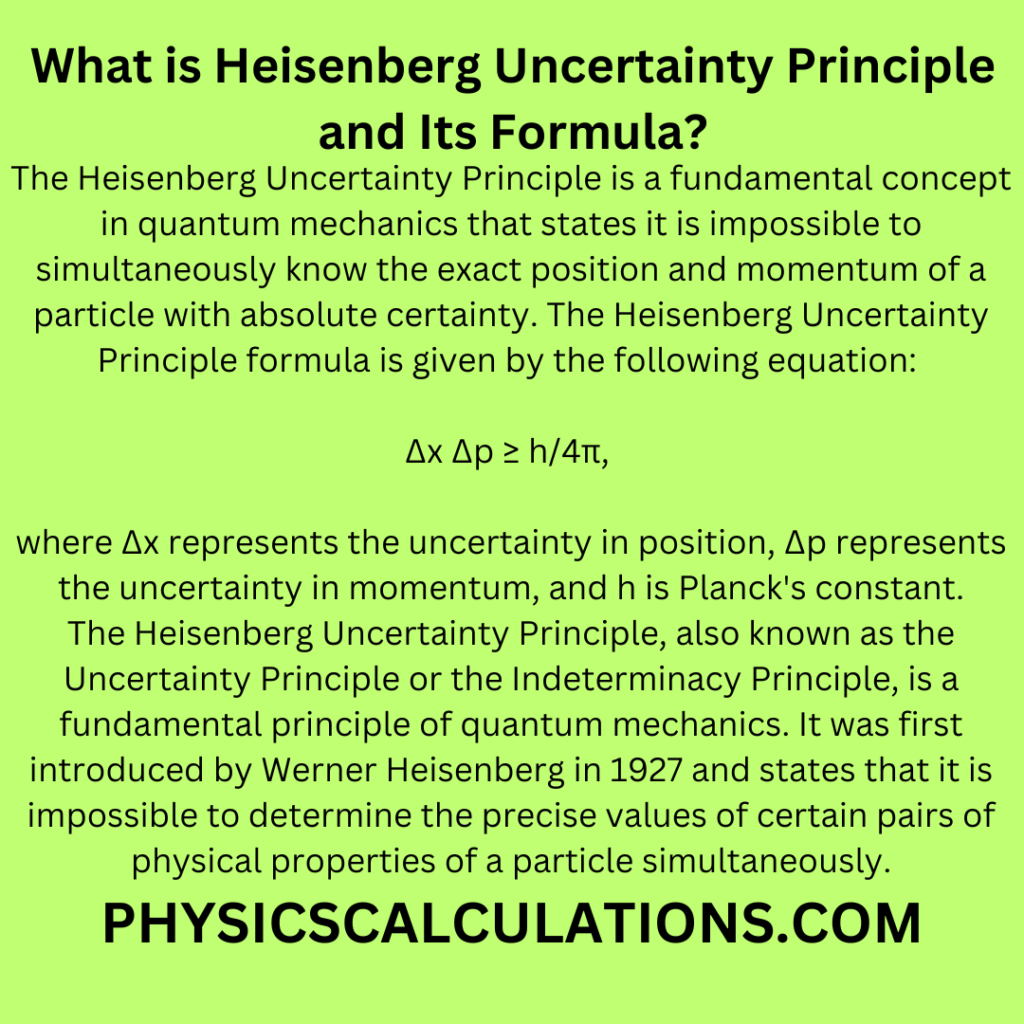
The Heisenberg Uncertainty Principle is a fundamental concept in quantum mechanics that states it is impossible to simultaneously know the exact position and momentum of a particle with absolute certainty. The Heisenberg Uncertainty Principle formula is given by the following equation:
Δx Δp ≥ h/4π,
where Δx represents the uncertainty in position, Δp represents the uncertainty in momentum, and h is Planck’s constant.
The Heisenberg Uncertainty Principle, also known as the Uncertainty Principle or the Indeterminacy Principle, is a fundamental principle of quantum mechanics. It was first introduced by Werner Heisenberg in 1927 and states that it is impossible to determine the precise values of certain pairs of physical properties of a particle simultaneously.
The Heisenberg Uncertainty Principle deals with the relationship between two fundamental physical properties of a particle – position and momentum. According to the principle, the more precisely we know the position of a particle, the less precisely we can know its momentum, and vice versa. This means that the position and momentum of a particle cannot be measured at the same time with absolute precision.
The uncertainty principle has important implications for the behavior of subatomic particles, which do not behave according to classical physics. It means that we cannot predict with absolute certainty the behavior of a particle, and it is impossible to measure both the position and momentum of a particle accurately at the same time.
The Heisenberg Uncertainty Principle has been experimentally verified countless times and is considered one of the fundamental principles of modern physics. It has played a crucial role in the development of quantum mechanics and has led to many important discoveries and applications in fields such as electronics, medicine, and materials science.
You may also like to read:
Historical Background
The Heisenberg Uncertainty Principle, also known as the Uncertainty Principle or the Indeterminacy Principle, is one of the fundamental principles of quantum mechanics. It was first introduced by Werner Heisenberg in 1927 and is considered one of the most significant discoveries in modern physics.
Heisenberg’s work on the Uncertainty Principle was a result of his attempts to understand the behavior of subatomic particles. At the time, scientists were trying to reconcile the behavior of particles with classical physics, but the observations of subatomic particles could not be explained using classical physics.
Heisenberg proposed that the behavior of subatomic particles could be explained using a new framework called quantum mechanics. He suggested that particles do not have precise values of properties like position and momentum, but instead have a range of possible values, each with a probability of occurrence.
The Uncertainty Principle arose from Heisenberg’s attempts to measure the position and momentum of particles. He showed that the more precisely we know the position of a particle, the less precisely we can know its momentum, and vice versa. This led to the mathematical expression of the Uncertainty Principle, which relates the uncertainty in position and momentum of a particle.
Heisenberg’s work on the Uncertainty Principle was met with skepticism from some physicists, but it was soon validated through experimental evidence. The principle played a crucial role in the development of quantum mechanics and has led to many important discoveries and applications in fields such as electronics, medicine, and materials science.
Importance and relevance to modern physics
The Heisenberg uncertainty principle is one of the most important and fundamental principles in modern physics. It states that the position and momentum of a particle cannot both be precisely measured at the same time. In other words, the more precisely we know the position of a particle, the less precisely we can know its momentum, and vice versa. This principle is a cornerstone of quantum mechanics, and it has far-reaching implications for the behavior of subatomic particles.
The importance of the Heisenberg uncertainty principle lies in its implications for our understanding of the universe. It shows us that the behavior of particles at the quantum level is fundamentally different from that of classical particles. It also has important implications for technological applications, such as the development of quantum computing.
The uncertainty principle has been used to explain a wide range of phenomena, from the stability of atomic nuclei to the behavior of superconducting materials. It has also led to the development of new technologies, such as the scanning tunneling microscope, which can image individual atoms and molecules with unprecedented resolution.
Perhaps the most significant implication of the uncertainty principle is that it places fundamental limits on the precision with which we can make measurements. This has important implications for the development of new technologies, such as quantum sensors and atomic clocks. It also has implications for our understanding of the universe, as it suggests that there may be inherent limits to our ability to observe and measure the behavior of particles at the quantum level.
Explanation of Wave-Particle Duality
The wave-particle duality is one of the most fundamental concepts in modern physics. It refers to the fact that particles, such as electrons and photons, can exhibit both wave-like and particle-like behaviour. We intimately connect this duality with the Heisenberg Uncertainty Principle.
The Heisenberg Uncertainty Principle states that there is a fundamental limit to our ability to measure the position and momentum of a particle. Specifically, the more precisely we measure the position of a particle, the less precisely we can measure its momentum, and vice versa. This is because the act of measuring the position of a particle disturbs its momentum, and vice versa.
This uncertainty in measurements is directly related to the wave-particle duality. In quantum mechanics, particles are described by wave functions that exhibit wave-like properties, such as interference and diffraction. The wave function represents the probability amplitude of finding the particle at a particular location, but it does not represent a definite position until it is measured.
When we measure a particle, its wave function “collapses” to a specific position, and the momentum becomes uncertain. This means that we can no longer measure the particle as purely as a wave, but we must treat it as a particle with a definite position.
In other words, the wave-particle duality is a result of the fact that particles can exhibit wave-like behaviour, but when we measure them, they behave like particles with a definite position. The Heisenberg Uncertainty Principle is the mathematical expression of this duality, and it places a fundamental limit on our ability to measure the properties of particles.
Impacts of the Principle in Macroscopic and Quantum Worlds
The Heisenberg Uncertainty Principle manifests differently in the macroscopic and quantum worlds. In the macroscopic world, we safely ignore objects with substantial mass, such as a basketball, because of the negligible uncertainties in position and momentum. However, as we venture into the quantum realm, where the masses of atoms and subatomic particles are minuscule, these uncertainties become significant.
In the quantum world, any attempt to increase the precision of measuring an object’s position is met with an increase in the uncertainty of its momentum. This inherent trade-off arises from the wave-particle duality, making precise measurements a challenging endeavour. For small particles like electrons, the limitations imposed by the Heisenberg Uncertainty Principle are unmistakable and have far-reaching consequences.
Measurement and Its Impact on Position and Momentum
To understand the Heisenberg Uncertainty Principle, it’s crucial to comprehend the intricate relationship between measurement, position, and momentum. Let’s illustrate this principle through a simple example. Consider measuring the position of an electron, a tiny and elusive particle. In this endeavour, we use photons to interact with the electron and determine its position.
Here’s where the magic—and the uncertainty—begins. Photons carry momentum, albeit small, and when they collide with the electron, they impart some of their momentum to it. This transfer of momentum causes the electron’s position to become uncertain, as its momentum has been altered by the collision. Thus, the act of measuring the position of a particle inevitably increases the uncertainty in its momentum.
The story is quite different in the macroscopic world. Imagine measuring the position of a basketball using light. While there is still a transfer of momentum from the photons to the ball, the effect is negligible because the ball’s mass will dwarf the photon’s mass. In this case, the uncertainties in position and momentum are practically insignificant.
Mathematical Derivation of Uncertainty Principle
The Heisenberg uncertainty principle is a fundamental principle in quantum mechanics that describes the limitations on our ability to measure certain properties of particles. It states that it is impossible to measure both the position and momentum of a particle with arbitrary precision at the same time.
We derive the uncertainty principle from the commutation relation between two quantum mechanical observables. In the case of position and momentum, the commutation relation is:
[x, p] = xp – px = iħ
Where x is the position operator, p is the momentum operator, i is the imaginary unit, and ħ is the reduced Planck constant. The brackets denote a commutator, which measures the degree to which two operators fail to commute.
Using the properties of the commutator, it is possible to derive the uncertainty principle. The derivation starts with the assumption that a particle’s position and momentum have well-defined values. It then uses the commutation relation to show that the product of their uncertainties is bounded from below by a non-zero constant.
The uncertainty principle is:
ΔxΔp ≥ ħ/2
Where Δx is the uncertainty in position, Δp is the uncertainty in momentum, and ħ/2 is the minimum value that their product can take. This means that the more precisely we know the position of a particle, the less precisely we can know its momentum, and vice versa.
The uncertainty principle has far-reaching implications for quantum mechanics and its applications in modern physics. It implies that there is a fundamental limit to the accuracy of our measurements and that the properties of particles are inherently uncertain. We can see this uncertainty in many quantum mechanical phenomena, such as the wave-particle duality of particles.
Uncertainty Principle, Energy and Time Equation
The Uncertainty Principle does not stop at momentum; it extends to energy and time as well. The equation is:
∆E ∙ ∆t ≥ ħ
Here, ∆E signifies the uncertainty in energy, ∆t symbolizes the uncertainty in time, and once again, ħ is the constant that ties it all together.
The Origin of the Uncertainty Principle
The roots of the Heisenberg Uncertainty Principle are deeply embedded in the dual nature of particles. As we mentioned earlier, particles exhibit both wave-like and particle-like behavior. The probability of finding particles is highest where their waveforms are most pronounced. However, when the waveform is distinct, the particle’s position becomes uncertain, and vice versa.
To put it simply, if we measure one aspect of a particle with great accuracy, it leads to significant uncertainty in measuring the other. This intrinsic relationship is a product of the dual nature of particles and forms the foundation of the Uncertainty Principle.
Equations Related to the Uncertainty Principle
In addition to the fundamental equations mentioned earlier, there are other equations closely related to Heisenberg’s Uncertainty Principle that offer insights into the behavior of quantum systems. These equations help us understand the relationship between position, momentum, energy, and time in the quantum realm.
One such equation connects the uncertainty in velocity (∆V) to the uncertainty in position (∆x) and mass (m). The formula is:
∆x ∙ ∆V ≥ ħ / 2m
Where ∆V is the uncertainty in velocity, ∆x is the uncertainty in position, ħ is the reduced Planck constant, and m is the mass of the particle. This equation reveals that as the mass of a particle increases, the impact of the Uncertainty Principle diminishes.
Another interesting aspect of the Uncertainty Principle is its impact on the design of scientific experiments. When conducting experiments in the quantum realm, scientists must carefully consider the effects of measurement on particles. The very act of measurement disturbs the particles under observation, making it impossible to precisely measure multiple variables simultaneously.
Implications for Quantum Mechanics
The Heisenberg Uncertainty Principle is a cornerstone of quantum mechanics, and its implications are profound. This principle sets a fundamental limit on the simultaneous measurement of certain quantum variables. Let’s explore some of these implications in more detail.
Firstly, the Uncertainty Principle implies that there is an intrinsic limit to the accuracy with which we can measure the energy of a quantum system in a finite amount of time. When we attempt to measure the energy of a system with high precision, it affects the measurement of time. Conversely, when we aim for precise time measurement, it increases the uncertainty in energy. This relationship challenges the notion of perfect measurements in the quantum world.
Consequences for Scientific Experiments
The Heisenberg Uncertainty Principle has direct consequences for the design and interpretation of scientific experiments in the quantum realm. It forces us to confront the reality that when we interact with particles to measure one of their properties, we inevitably affect the other properties.
Consider, for example, the field of spectroscopy, which plays a crucial role in understanding the behaviour of atoms and molecules. Spectroscopy relies on precise measurements of the energy levels of particles by analyzing the spectral lines they emit or absorb. However, the Uncertainty Principle tells us that in the act of measuring these energies, we disturb the very particles we are trying to study.
In spectroscopy, this results in the broadening of spectral lines. The Uncertainty Principle imposes a limit on how precisely we can determine the energy levels, leading to what we know as spectral line widths. These line widths provide valuable information about the uncertainties associated with the energy measurements.
Understanding the Wave-Like Nature of Particles
To truly appreciate the Heisenberg Uncertainty Principle, we must delve into the concept of particles as both discrete entities and wave-like phenomena. This wave-particle duality suggests that a particle’s properties, such as position and momentum, are not fixed but rather described by probability distributions. In other words, particles do not have definite positions or momenta until measured.
The Uncertainty Principle highlights the challenges of precisely measuring properties that inherently lack certainty. When we attempt to narrow down the position of a particle, we’re essentially trying to confine a wave-like phenomenon within a precise location. This results in an increase in uncertainty in the particle’s momentum.
Small Particles vs. Macroscopic Objects
One intriguing aspect of the Heisenberg Uncertainty Principle is its distinct impact on small particles when we compare it to macroscopic objects within the same system. The principle becomes far more noticeable in the quantum world, where particles like electrons, with their incredibly low masses, are subject to its constraints.
As an illustrative example, let’s consider the measurement of an electron’s position. If we accurately measure the electron’s position to within its size, the uncertainty in its momentum becomes substantial. In fact, the error in the momentum measurement can be significantly larger than the actual momentum of the electron. This demonstrates how the Uncertainty Principle imposes strict limits on the precision of position and momentum measurements for small particles.
In contrast, macroscopic objects, such as a basketball, experience a negligible impact from the Uncertainty Principle during measurements. Even though there is still a transfer of momentum from the photons used to measure the basketball’s position, the effect is minuscule because the photon’s mass is dwarfed by the basketball’s mass. Consequently, for large objects, we can safely ignore the uncertainties in position and momentum.
The Role of Position and Momentum in the Uncertainty Principle
The Heisenberg Uncertainty Principle is a fundamental concept in quantum mechanics that states that it is impossible to simultaneously measure the exact position and momentum of a particle. This principle has far-reaching implications and has led to a paradigm shift in our understanding of the behavior of subatomic particles.
The uncertainty principle arises from the fact that in quantum mechanics, the act of measuring a particle’s position or momentum disturbs the system under observation. The more precisely we try to measure the position of a particle, the less precisely we can determine its momentum, and vice versa.
The role of position and momentum in the uncertainty principle is central to its formulation. Position refers to the location of a particle in space, while momentum refers to the mass and velocity of the particle. In classical mechanics, it is possible to measure both the position and momentum of a particle with arbitrary precision. However, in the quantum world, this is no longer the case.
Mathematically, the uncertainty principle is ΔxΔp ≥ h/4π, where Δx is the uncertainty in position, Δp is the uncertainty in momentum, and h is Planck’s constant. This equation tells us that the product of the uncertainties in position and momentum is always greater than or equal to a constant value. Therefore, the more precisely we measure one variable, the less precisely we can measure the other.
We can understand the role of position and momentum in the uncertainty principle through the concept of wave-particle duality. In the quantum world, particles exhibit wave-like behavior and waves exhibit particle-like behavior. This means that particles do not have a precise position and momentum until we observe them. Therefore, the act of measurement disturbs the system we observe, and the uncertainty principle comes into play.
Application and interpretation of the uncertainty principle
The uncertainty principle is one of the fundamental concepts in quantum mechanics. It tells us that we cannot know the position and momentum of a particle precisely at the same time. This principle has far-reaching applications in various fields of science and technology, including physics, chemistry, engineering, and even finance.
One of the most significant applications of the uncertainty principle is in quantum mechanics itself. The principle sets a fundamental limit on the precision with which we can make measurements. This means that there will always be some degree of uncertainty in any measurement of the position and momentum of a particle. Thus, the uncertainty principle plays a critical role in understanding the behavior of particles at the quantum level.
The uncertainty principle also has significant implications in chemistry. For example, it helps explain why certain chemical reactions occur while others do not. It also helps predict the behavior of molecules in various chemical environments.
In engineering, we use the uncertainty principle to design systems that operate at the quantum level. For example, it plays a critical role in the development of quantum computers, which use the principles of quantum mechanics to perform calculations much faster than classical computers.