Half-life Problems And Answers Examples
Half-life Problems And Answers Examples: Here are 18 half-life problems and answers to help you understand how to solve a question about half-life:
Problem 1
In 24 days, a radioactive isotope decreased in mass from 64 grams to 2 grams. What is the half-life of the radioactive material?

Answer
The half-life of the radioactive material is 4.8 days.
Explanation
We are going to apply two methods to arrive at our answer
Method 1: Conventional method
64 g to 32 g = 1 half-life
32 g to 16 g = 2 half-life
16 g to 8 g = 3 half-life
8 g to 4 g = 4 half-life
4 g to 2 g = 5 half-life
If 5 half-life is equal to 24 days. Then 1 half-life will be T
Therefore, T x 5 half-life = 1 half-life x 24 days
Hence
T = (1 half-life x 24 days) / 5 half-life = 4.8 days
Method 2: Zhepwo Method
Decay time, t = 24 days
Half-life, T = ?
Initial mass, N1 = 64 g
Final mass, N2 = 2 g
We will use the formula
R = N1 / N2 = 64 / 2 = 32
T = t / (log2R) = 24 / (log232) = 24 / 5 = 4.8 days
Therefore, the half-life of the radioactive material is 4.8 days
Problem 2
The half-life of radioactive material is 6 hours. What quantity of 1 kg of the material would decay in 24 hours?
Answer
The final answer to the above question is (15/16) 0.9375 kg

Explanation
We will also apply two methods to solve the above question
Method 1: Conventional Method
After 6 hours, 1/2 kg decays, and 1/2 kg will remain
Another 6 hours, 1/2 of 1/2 kg decays, 1/4 kg remains
6 hours after, 1/2 of 1/4 kg decays, 1/8 kg remains
After another 6 hours, 1/2 of 1/8 kg decays, 1/16 kg remains
Therefore, the material decayed would be:
1 kg – 1/16 kg = (15 / 16) kg = 0.9375 kg
Method 2: Zhepwo Method
Half-life, T = 6 hrs
Initial mass, N1 = 1 kg
Mass of the decayed material, Nd = ?
Formula
T = t/n
This implies that
n = t / T = 24 / 6 = 4
R = 2n = 24 = 16
Nd = N1 (R – 1 / R) = 1 (16-1 / 16) = (15/16) kg
Therefore, the quantity of 1 kg of the material that would decay in 24 hours is 15/16 kilograms or 0.9375 kilograms.
Problem 3
A radioactive sample initially contains N atoms. After three (3) half-lives, what is the number of atoms that have disintegrated?
Answer
The final answer to the above question is (7/8) N

Explanation
We will use two methods to solve the above question
Method 1: Conventional method
First step: After 1 half-life, N/2 decay, N/2 remain
Second Step: After 2 half-life, 1/2 of N/2 decay, N/4 remain
Third step: After 3 half-life, 1/2 of N/4 decay, N/8 remains
The number of atoms disintegrated = Sum of the disintegrated atoms or fractions
This is now equal to
= N/2 + (1/2 x N/2) + (1/2 x N/4) = N/2 + N/4 + N/8 = (7/8)N
Method 2: Zhepwo Method
Alternatively, we can also apply the following steps to solve the problem using Zhepwo method:
Initial number of atoms, N1 = N
Number of half-lives, n = 3
Also, the number of atoms decayed, Nd = ?
Solution
R = 2n = 23 = 8
Nd = N1 (R – 1 / R) = N ( 8 – 1 / 8) = N x 7/8 = (7/8)N
Therefore, the number of atoms that have disintegrated is (7/8)N
Problem 4
After three half-lives, the fraction of a radioactive material that has decayed is
Answer
The final answer to the above question is 7/8

Explanation
We will use two methods
Method 1: Conventional Method
1 half-life implies 1/2 decays, ,1/2 remains
2 half-life shows that 1/4 decays, 1/4 remains
3 half-life, 1/8 decays, 1/8 remains
Hence, the fraction decayed = original fraction – remaining fraction = (1 – 1/8) = 7/8
Note that the original fraction is 1/1 which is equal to 1
Method 2: Zhepwo Method
Number of half-lives, n = 3
The fraction decayed fd = ?
Disintegration ratio, R = 2n = 23 = 8
fd = R – 1 / R = 8 – 1 / 8 = 7/8
Therefore, the fraction of radioactive material that has decayed is 7/8
Problem 5
The half-life of a radioactive element is 24 hours. Calculate the fraction of the original element that would have disintegrated in 96 hours.
Answer
The final answer to the above question is 15/16

Explanation
I will apply two methods to solve the problem
Method 1: Conventional Method
After 24 hrs, 1/2 disintegrate, 1/2 remain
Another 24 hrs, 1/4 decay, 1/4 remain
Next 24 hrs, 1/8 decay, 1/8 remain
The next 24 hrs, 1/16 decay, 1/16 remain
Fraction disintegrate = sum of decayed fractions = 1/2 + 1/4 + 1/8 + 1/16 = 15/16
Method 2: Zhepwo Method
Half-life, T = 24 hrs
Decay time, t = 96 hrs
Fraction disintegrated, fd = ?
Solution
T = t/n
This implies that
n = t/T = 96/24 = 4
R = 2n = 24 = 16
fd = (R – 1) / R = (16 – 1) / 16 = 15/16
Therefore, the fraction of the original element that would have disintegrated in 96 hours is 15/16.
Problem 6
A radioactive isotope has a half-life of 20 hours. What fraction of the original radioactive nuclei will remain after 80 hours?
Answer
The final answer to the above question is 1/16
Explanation
We will still use the two methods to find the answer to the problem
Method 1: Conventional Method
Let n be the original number of nuclei
After 20 hours, n/2 disintegrates and n/2 remains
The next 20 hours, 1/2 of n/2 disintegrates and n/4 remains
Next 20 hrs, 1/2 of n/4 decayed, and n/8 remains
After another 20 hrs, 1/2 of n/8 decayed, and n/16 remains
Method 2: Zhepwo Method
Half-life, T = 20 hrs
Decay time, t = 80 hrs
Number of half-lives, n = t/T = 80/20 = 4
Disintegration ratio, R = 2n = 24 = 16
Fraction remaining, fr = 1/R = 1/16
We can also use an alternative method
T = 20, t = 80
Fraction remaining, fr = 1 / (2t/T) = 1 / (280/20) = 1 / 24 = 1 / 16
Therefore, after 80 hours. The fraction of the original number remaining would be 1/16
Problem 7
Two radioactive elements A and B have half-lives of 100 and 50 years respectively. Samples of A and B initially contain equal number of atoms. What is the ratio of remaining atoms of A to that of B after 200 years?
Answer
The ratio of remaining atoms of A to that of B after 200 years is 4:1
Explanation
You can employ any of the two methods below to arrive at your answer:
1. Conventional Method:
When we allow n to be as the original number of the nuclei
Sample A: Half-life = 100 years
After 100 years, n/2 disintegrates and n/2 remains
Additionally, after 100 years, n/4 disintegrates and n/4 remains
Thus, after 200 years, the fraction of the original number that will remain is 1/4
Sample B: Half-life = 50 years
After 50 years, n/2 disintegrate, n/2 remain
Another 50 years, n/4 disintegrate and n/4 will remain
50 years more, n/8 will decay, n/8 remain
After 50 years, n/16 decay, n/16 remain
Therefore, after 200 years, fraction of atoms that will remain is 1/16
Ratio of A:B = 1/4 : 1/16
We will now multiply both sides by 16 to obtain:
The ratio of A:B = 4 : 1
2. Zhepwo Method
T is the Half-life for A and B which is 100 and 50 respectively
And t is the decay time for A and B which is 200 and 200 respectively
The number of half-lives n = t/T which is A = 200/100 = 2, and B = 200/50 = 4
Disintegrating ratio R = 2n and it will give us 22 = 4 and 24 = 16
The initial number of atoms, N1 = 1
Thus, we have A = 16 (1/4) = 4, and B = 16 (1/16) = 1
Hence we have a ratio of A:B = 4:1
Problem 8
A radioactive substance has a half-life of 80 days. If the initial number of atoms in the sample is 6 x 1010, how many atoms would remain at the end of 320 days?
Answer
The number of atoms that would remain at the end of 320 days is 3.8 x 109 atoms
Explanation
T = 80; t = 320; and N1 = 6 x 1010
We will also use the formula N2 = (N1 / 2t/T)
Therefore, by substituting our data into the above formula, we will have:
N2 = (N1 / 2t/T) = (6 x 1010) / 2320/80 = 6 x 1010 / 24 = 6 x 1010 / 16 = 3.8 x 109 atoms
Problem 9
A percentage of the original nuclei of a sample of a radioactive substance left after 5 half-lives is?
Answer
The percentage left is 3%
Explanation
Number of hlaf-lives (n) = 5
original amount or fraction (N1) = 1
Disintegrating ratio, R = 2n = 25 = 32
Fraction remaining, fr = 1/R
The formula we will apply is:
Percentage of the original left = [amount left (fraction remaining) / original amount] x 100
Therefore,
Percentage of the original left = (fr / N1) x 100 = [(1/32) / 1] x 100 = [1/32] x 100 = 3.125 = 3%
Problem 10
A radioactive substance of mass 768 grams has a half-life of 3 years. After how many years does this substance leave only 6 grams undecayed?
Answer
The answer is 21 years
Explanation
Half-life T = 3 years
Initial mass present, N1 = 768 g
Final mass remaining, N2 = 6 g
Decay time, t = ?
The disintegration ratio, R = N1 / N2 = 768 / 6 = 128
from the formula below:
T = t / (log2R)
Making t subject of the formula and substituting our values, we will have:
t = T x log2R = 3 x log2128 = 3 x 7 = 21 years
Problem 11
An element whose half-life is 10 days is of mass 12 grams. Calculate the time during which 11.25 grams of the element would have decayed.
Answer
The final answer to this question is 40 days
Explanation
Half-life, T = 10 days
Initial mass present, N1 = 12 g
The mass of the element decayed, Nd = 11.25 grams
Nd = N1 – N2
Thus, N2 = N1 – Nd = 12 – 11.25 = 0.75 grams
R = N1 / N2 = 12 / 0.75 = 16
Applying the formula T = t / (log2R) and making t the subject of the formula, we will have:
t = T x log2R = 10 x log216 = 10 x 4 = 40 years
Problem 11
A radioactive element decreases in mass from 100g to 15g in 6 days. What is the half-life of the radioactive material?
Answer
The half-life of the radioactive element is 2.2 days
Explanation
Initial mass present, N1 = 100g
Final mass present, N2 = 15g
Decay time, t = 6 days
Disintegration ratio, R = N1 / N2 = 100 / 5 = 6.67
The half-life formula T = t / (log2R) = 6 / log26.67
Therefore, we can now say that
T = (6 x log2) / log26.67 = (6 x 0.30103) / 0.824 = 2.2 days
Problem 12
The time it will take a certain radioactive material with a half-life of 50 days to reduce 1/32 of its original number is
Answer
The final answer to the above question is 250 days
Explanation
Half-life, T = 50 days
Decay time, t = ?
Disintegration ratio, R = 32
Fraction remaining, fr = 1/R = 1/32
Using the formula, we will obtain
t = T x log2R = 50 x log232 = 50 x 5 = 250 days
Problem 13
A radioactive substance has a half-life of 3 minutes. After 9 minutes, the count rate was observed to be 200, what was the count rate at zero time?
Solution
Note that count rate at zero time is the same as the initial count rate
9 min ===> count rate 200
6 min ===> count rate (200 x 2) = 400
3 min ===> count rate (400 x 2) = 800
0 min ===> count rate (800 x 2) = 1600
Therefore, count rate at zero time is 1600
Problem 14
The count rate of a radioactive material is 800 count/min. If the half-life of the material is 4 days, what would the the count rate be 16 days later?
Solution
Initial count rate = 800 count/min
Half-life = 4 days
After 4 days ==> (1/2) x 800 = 400 count/min
8 days after ==> (1/2) x 400 = 200 count/min
12 days after ==> (1/2) x 200 = 100 count/min
4 days after ==> (1/2) x 100 = 50 count/min
Therefore, the count rate 16 days later is 50 count/min
Problem 15
The half-life of a radioactive source is 1 minute. If a rate meter connected to the source registers 200μA at a given time, what would be its reading after 3 minutes?
A rate meter measures the count rate of radioactive of radioactive substance
Halff-life = 1 minute
1 minutes after, rate meter reads (1/2) x 200μ = 100μ
2 minutes after, rate meter reads (1/2) x 100μ = 50μ
3 minutes after, rate meter reads (1/2) x 50μ = 25μ
Therefore, rate meter reading after 3min is 25μ.
Problem 16
In 90 seconds, the mass of a radioactive element reduces to 1/32 of its original values. Determine the half-life of the element.
Solution
Decay time, t = 90 seconds
Fraction of initial mass remaining, Fr = 1/32
Additionally, Fr = 1/R
Therefore, by equating the above formulas, we will have
1/R = 1/32
This implies that R = 32
Hence, Half-life (T) = t / (log2R) = 90 / (log232) = 90 / 5 = 18 seconds
Problem 17
A radioactive substance has a half-life of 3 days. If a mass of 1.55 g of this substance is left after decaying for 15 days, determine the original value of the mass.
Solution
Half-life (T) = 3 days2
Decay time, t = 15 days
Final mass remaining, N2 = 1.55 g
T = t/n
Therefore, n = t/T = 15/3 = 5
Disintegrating ratio, R = 2n = 25 = 32
R = N1 / N2
Hence, N1 = RN2 = 32 x 1.55
Thus, the original mass N1 =49.6 grams
Problem 18
In 90 seconds, the mass of a radioactive element reduces to 1/16 of its original value. Determine the half-life of the element.
Solution
Decay time t = 90 seconds, Fraction of mass remaining, Fr = 1/16
But Fr = 1/R
Therefore, 1/R = 1/16, and the value of R = 16
Half-life (T) = t / (log2R) = t / (log216) = 90/4 = 22.5 seconds
Picture of Half-Life Formula
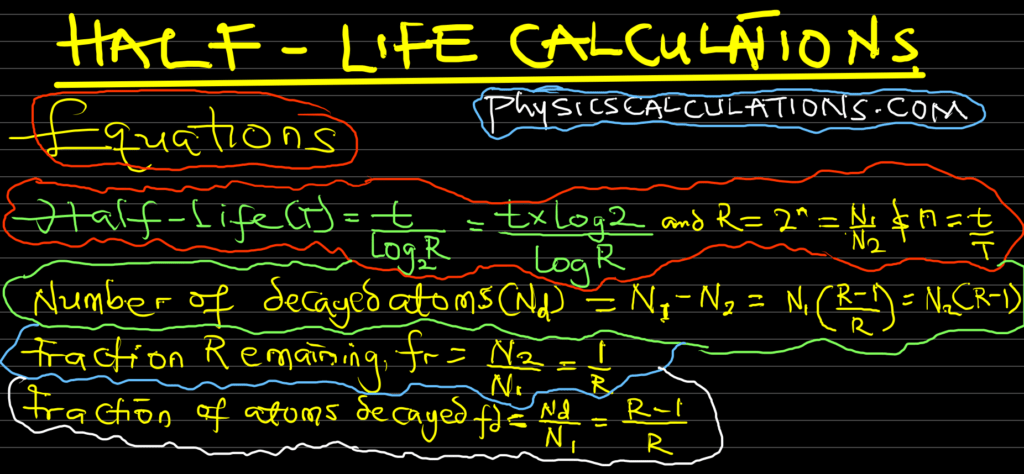
You may also like to read: