Solutions to the Problems of Projectile Motion
Here are 11 solved problems of projectile motion to help you understand how to tackle any related question:
Problem 1
A stone is shot out from a catapult with an initial velocity of 65 meters per second at an elevation of 60 degrees. Find
a. Time of flight
b. Maximum Height attained
c. The range
Data:
Initial velocity, u = 65 m/s
The angle of elevation, θ = 600
Gravitational acceleration, g = 10 m/s2
a. To find the time of the flight, we will apply the following methods:
Unknown
Time of flight, T = ?
Formula
The formula for solving a problem on time of flight, T is (2usinθ) / g
Solution
We will now insert our data into the above formula
Since T = (2usinθ) / g
T = (2 x 65 x sin600) / 10 = (130 x 0.866) / 10 = 112.58 / 10 = 11.258 = 11.3 seconds
Therefore, the time of flight is 11.3 seconds
b. Here is how to calculate the maximum height
Unknown
Maximum Height, Hmax = ?
Formula
The formula for calculating the maximum height is
Maximum height, Hmax = (u2sin2θ) / 2g
Solution
We can now substitute our formula with the data
The maximum height, Hmax = (652sin2600) / 2 x 10
Therefore,
Hmax = (4225 x (sin600)2) / 2 x 10
And the above equation will give us
Hmax = (4225 x 0.75) / 20
Hence
Hmax = 3168.75 / 20 = 158.4375 m
Our maximum height can be approximated into
Hmax = 158 m
Therefore, our maximum height is 158 meters.
c. We will find the range
Unknown
The range, R = ?
Formula
Here is the formula that will help us find the range
The range, R = (u2sin2θ) / g
Solution
The range, R = (652sin2(600) / g
And the above expression will give us
R = (4225sin1200) / 10 = (4225 x 0.866) / 10 = 3658.85 / 10 = 365.885 m
Therefore, the range of the stone shot out of the catapult is 365.885 meters.
Problem 2
A bullet is fired horizontally with a velocity of 40 meters per second from the top of a building 50 meters high. How far from the feet of the building will the bullet be assumed to touch the ground? (Take the gravitational acceleration, g = 10 m/s2).
Data
Horizontal velocity = Initial velocity = ux = 40 m/s
Height of the building, H = 50 m
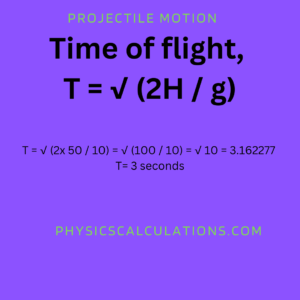
Time of flight, T = √ (2H / g)
Hence,
T = √ (2x 50 / 10) = √ (100 / 10) = √ 10 = 3.162277 = 3 seconds
The range, R = uT = 40 x 3 = 120 m
Therefore, the bullet will cover 120 meters from the foot of the building to the ground.
Problem 3
A tennis ball is thrown vertically upwards from the front with a velocity of 50 m/s. Calculate
a. The maximum height reached
b. The time it takes to reach the maximum height
c. The time of the flight
Data
Initial velocity, u = 50 m/s
a. Here is how to find the maximum height of the tennis ball
Formula
The formula for finding the maximum height is
Hmax = u2 / 2g
Solution
After inserting our data into the formula Hmax = u2 / 2g, we will have
Maximum height, Hmax = (50)2 / 2 x 10 = 2,500 / 20 = 125 m
Therefore, the maximum height reached by the tennis ball is 125 meters
b. The time taken to reach the maximum height can be calculated this way:
Unknown
The time it takes to reach the maximum height, t = ?
Formula
The formula to apply is t = u / g
Solution
We will apply our data to the formula t = u / g
Time, t = 50 / 10 = 5 seconds
c. Time of flight will be
T = 2u / g = (2 x 50) / 10 = 100 / 10 = 10 seconds.
Problem 4
A bullet is fired from a point making an angle of 36 degrees to the horizontal. The initial velocity of the bullet is 43 m/s. Find
a. The greatest height reached
b. The time taken to reach the maximum height
[Take gravitational acceleration, g = 9.8 m/s2]
Data
The angle made, θ = 360
Initial velocity, u = 43 m/s
Gravitational acceleration, g = 9.8 m/s2
a. We can calculate the maximum height, Hmax this way
Unknown
The maximum height, Hmax = ?
Formula
Maximum height, Hmax = (u2sin2θ) / 2g
Solution
Apply the data into Hmax = (u2sin2θ) / 2g to obtain
Hmax = (432sin2360) / 2 x 9.8
Thus
The maximum height, Hmax = (1849 x 0.34549) / 19.6 = 638.8 / 19.6 = 32.59 = 33 m
Therefore, the maximum height reached is 33 meters.
b. The time of flight will be
Time of flight, T = usinθ / g = 43sin360 / 9.8 = (43 x 0.59) / 9.8 = 25.37 / 9.8 = 2.589 s
Therefore, the time of flight is 2.6 seconds
Problem 5
A projectile is fired with an initial velocity of 100 m/s at an angle of 30 degrees with the horizontal. Calculate:
a. The time of flight
b. The maximum height attained
c. The range

Data
The initial velocity of the projectile, u = 100 m/s
Angle of inclination, θ = 300
Gravitational acceleration, g = 10 m/s2
Solution a
To find the time of flight, we will follow the method below:
Formula
we will apply
Time of flight, T = (2usinθ) / g
We will now put our data into the formula
T = (2 x 100 x sin300) / g = (200 x 0.5) / 10 = 100 /10 = 10 s
Therefore, the time of flight is10 seconds
Solution b
To find the maximum height attained, we will use
Formula
Maximum height, Hmax = (u2sin2θ) / 2g
We can now substitute our formula with our data
Hmax = (1002 x sin2300) / (2 x 10)
Hmax = (10,000 x (0.5)2) / 20 = (10,000 x 0.25) / 20 = 2,500 / 20 = 125 m
Therefore, the maximum height attained by the projectile is 125 meters
Solution c
Now, we are going to calculate the range
Formula
The range, R = (u2sin2θ) / g
After inserting our data into the above formula, we will get
R = (1002sin2 x 300) / 10 = (10,000 x sin600) / 10 = (10,000 x 0.866) / 10
And we will have
R = 8660 / 10 = 866 m
Therefore, our range is eight hundred and sixty-six (866) meters.
Problem 6
A bomber on a military mission is flying horizontally at a height of 3000 meters above the ground at 60 kilometers per minute. It drops a bomb on a target on the ground. Determine the acute angle between the vertical and the line joining the bomber and the target at the instant the bomb is released.

Data:
The horizontal velocity of the bomber aircraft = 60 km/min = (60 x 1000) / 60 = 60,000 / 60 = 1000 m/s
Initial velocity, u = 0
Gravitational acceleration, g = 10 m/s2
Height above the ground, h = 3000 m
Formula
The first formula is
h = ut + (1/2)gt2 to find t
The second formula
Distance covered in time t, s = horizontal velocity x time to find s
The third formula is
tanθ = s / h to find the angle of inclination (θ)
Solution
We will need to insert our data into the first formula to find t
h = ut + (1/2)gt2
Which can be rewritten as
3000 = (1/2) x 10 x t2 [Remember that u = 0]
By making t subject of the formula, we will have
(3000 x 2) / 10 = t2
t2 = 600
We can now take the square root of both sides to obtain
t = √600
And our final answer for the time will be
Time, t = 24.5 seconds
We will apply our second formula
Distance, s = horizontal velocity x time = 1000 x 24.5 = 24,500 meters
We will now proceed with our third formula
tanθ = s / h = 24,500 / 3000 = 8.2
Thus,
tanθ = 8.2
Hence, we divide both sides by tan to obtain a tan inverse of 8.2
θ = tan-18.2 = 83.0470
We can now approximate our answer into
θ = 830
Therefore, the angle of inclination is eighty-three (83) degrees.
Problem 7
A ball is projected horizontally from the top of a hill with a velocity of 30 meters per second. If it reaches the ground 5 seconds later. The height of the ball is
Data:
The initial velocity of the ball, u = 0
Time it takes to reach the ground, t = 5 s
Gravitational acceleration, g = 10 m/s2
Formula
Maximum height of the ball, h = ut + (1/2)gt2
Solution
To solve the problem, insert your data into the above formula
h = ut + (1/2)gt2 = 0 x 5 + (1/2) x 10 x 52 = (1/2) x 10 x 25 = (1/2) x 250 = 250 / 2 = 125 meter
Therefore, the height of the hill is 125 meters
Problem 8
A stone is projected at an angle of 60 degrees and an initial velocity of 20 meters per second. Determine the time of flight.
Data:
The angle of inclination of the stone, θ = 600
Initial velocity of the stone, u = 20 m/s
Gravitational acceleration, g = 10 ms-2
Unknown
Time of flight, T = ?
Formula
The formula for calculating the time of flight, T = (2usinθ) / g
Solution
We will put our data into the formula T = (2usinθ) / g
Now,
T = (2usinθ) / g = T = (2 x 20 x sin600) / 10 = (40 x 0.866) / 10 = 34.64 / 10 = 3.464 s
Therefore, the time of flight is 3.46 seconds.
Problem 9
A body is projected upward at an angle of 30 degrees with the horizontal at an initial speed of 200 meters per second. In how many seconds will it reach the ground? How far from the point of projection will it strike?
Data
Angle of projection, θ = 200 m/s
Horizontal initial speed, u = 200 m/s
Unknown
The question wants us to solve
Number of seconds for the body to reach ground = Time of flight, T = ?
We were also asked to find out how far from the point of projection will the body strike the ground. Hence, we are going to find the range.
Formula
First formula to find the time of flight, T = (2usinθ) / g
Second formula to find the range, R = (u2sin2θ) / g
Solution
To find the time of flight, we will insert our data into the first formula T = (2usinθ) / g
Time of flight, T = (2usinθ) / g = (2 x 200 x sin300) / 10
Hence
T = (400 x 0.5) / 10 = 200 / 10 = 20 s
Therefore, the time of flight is 20 seconds.
By applying the second formula R = (u2sin2θ) / g and inserting our data, we will have
R = (u2sin2θ) / g = (2002 x sin (2×300)) / 10
Which implies that
R = (40,000 x sin600) / 10 = (40,000 x 0.866) / 10
Thus,
The range, R = 34,640 / 10
Hence
R = 3464 m
Therefore, the range covered is 3,464 meters
Problem 10
A tennis ball projected at an angle θ attains a range of R = 78 meters. If the velocity imparted to the ball by the racket is 30 meters per second, calculate the angle θ.
Data
Range, R = 78 m
The velocity imparted to the ball by racket = initial velocity, u = 30 m/s
Gravitational acceleration, g = 10 m/s2
Unknown
The angle θ = ?
Formula
We will use the formula R = (u2sin2θ) / g
Solution
Substitute your formula with the data
78 = (302sin2θ) / 10
Hence
780 = (900sin2θ)
Sin2θ = 780 / 900
Thus,
Sin2θ = 0.867
After dividing both sides by sin, will now have
2θ = sin-10.867
Which is equal to
2θ = 60.110
After approximating 60.110 to 600. Therefore,
θ = 600 / 2 = 300
Therefore, the angle θ is 30 degrees
Problem 11
An aeroplane, flying in a straight line at a constant height of 500 meters with a speed of 200 meters per second drops a food package. The package takes a time t to reach the ground and travels a horizontal distance d in doing so. Taking g as 10 meters per second square, and ignoring air resistance, what are the values of t and d?
Data
The height, h = 500 m
Speed of the aeroplane = 200 m/s
Gravitational acceleration, g = 10 ms-2
Unknown
The time it takes the package to reach the ground, t = ?
Distance covered by the package to reach the ground, d = ?
Formula
First formula: We will apply t = √2h/g to find time (t)
Second Formula: We will use s = speed of the aeroplane x time it takes the package to drop on the ground [where s = distance covered by the package to reach the ground]
Solution
By applying our first formula t = √2h/g and inserting our data, we will obtain
t = √((2×500) / 10) = √1000/10 = √100 = 10 seconds
Therefore, the time it takes for the package to reach the ground is 10 seconds.
We will now apply the second formula to calculate the distance covered
Remember that s = speed of the aeroplane x time
Thus,
s = 200 x 10 = 2000 m
Therefore, the distance covered by the package to reach the ground is 2000 meters
You may also like to read:
How to Calculate Maximum height
How to Find Displacement in Physics