What is Linear Expansivity?
In this article, you will learn about linear expansivity: definition and calculations. I will also acquaint you with the symbols, and units of linear expansivity.
Definition: Linear expansivity of a substance can be defined as the fractional increase in length per unit length per degree rise in temperature.
The symbol for linear expansivity is α.
Also, the unit of linear expansivity is per degree celsius (0C-1) or per kelvin (K-1).
Video 1: Watch the comprehensive video explanation of linear expansivity below
The above video explained the definition of linear expansivity, its formula and few solved problems.
Additionally, Linear expansivity, often referred to as thermal expansion, is a material’s tendency to change its dimensions in response to temperature variations.
When a substance is heated, its particles gain energy and move more vigorously, causing them to expand and increasing the material’s volume.
Video 2: Watch the video explanation of solved problems of linear expansivity below:
When heat is applied to metal, the change in temperature would cause the object to expand. Different metals have different expansion rates when the same quantity of heat is applied to them. Some of these metals tend to expand more the others at the same temperature.
Linear expansivity is also known as the coefficient of linear expansion.
The coefficient of linear expansion is the reason these metals have different rates of expansion at the same temperature.
Linear Expansivity Formula and Increase in Length (Expansion) Formula
The linear expansivity formula is α = e/L0Δθ

But since e = extension = L – L0
Hence,
α = (L – L0 )/L0Δθ
We can also write the above formula as
α = (L – L0 )/L0(T2 – T1) or α = e/L0(T2 – T1)
and Δθ = T2 – T1
The formula for calculating the increase in length is L = L0(1 + αΔθ).

Where
α = Linear expansivity
L = change or increase the length
Also, L0 = original length
e = extension
Δθ = Change in temperature = T2 – T1
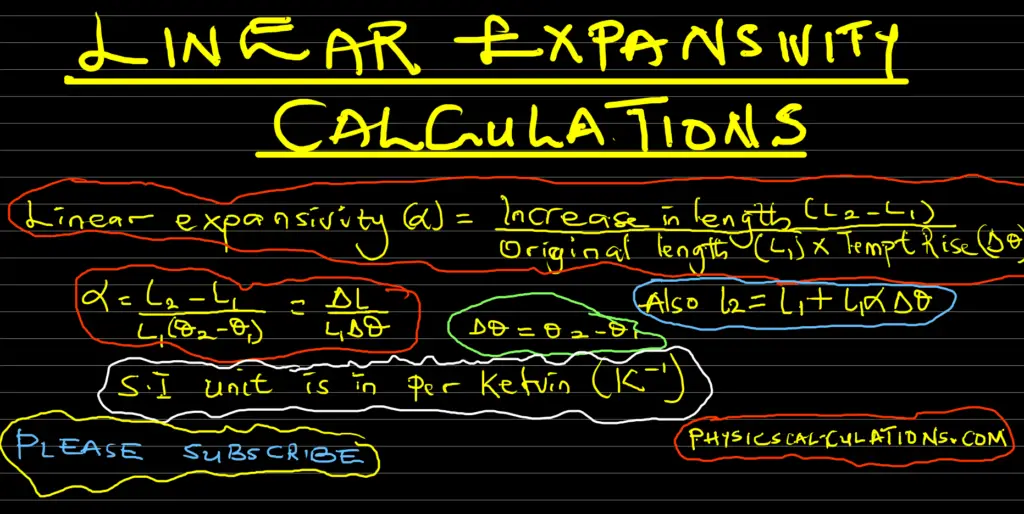
Table for Substances and their Linear Expansivity
Here is a table for materials, their symbols, and linear expansivity:
| The material | Symbol | Linear expansivity | Unit |
| Aluminium | Al | 0.000023 | k-1 |
| Iron | Fe | 0.000012 | k-1 |
| Copper | Cu | 0.000017 | k-1 |
| Brass | 0.000018 | k-1 | |
| Lead | Pb | 0.000029 | k-1 |
| Platinium | Pt | 0.000009 | k-1 |
| Zinc | Zn | 0.000030 | k-1 |
| Alloy (Invar) | 0.000001 | k-1 | |
| Glass | 0.0000085 | k-1 | |
| Silica | SiO2 | 0.0000004 | k-1 |
Linear Expansivity Calculations
Here are a few solved problems to help you understand how to calculate linear expansivity:
Question 1:
An invar of length 50 meters increases to 50.5 meters when heated from 200C to 700C. Calculate its linear expansivity.
Data
Original length, L0 = 50 m
The increase in length, L = 50.5 m
Initial temperature, T1 = 200C
Final temperature, T2 = 700C
Unknown value to find
Linear expansivity, α =?
Formula
α = (L – L0 )/L0(T2 – T1)
Solution
α = (L – L0 )/L0(T2 – T1) = (50.5 – 50)/50(70-20) = 0.5/(50 x 50) = 0.5/2,500 = 0.0002 K-1
Which implies that
α = 2 x 10-4 K-1
Therefore, the linear expansivity of the invar is 2 x 10-4 per kelvin.
Question 2:
A wire of length 5 meters is heated from a temperature of 200C to 700C. If it undergoes a change in length of 2 centimeters, find the linear expansivity of the wire.
Data
Original length, L0 = 5 m
Change in length = extension = 2 cm = (2/100) m = 0.02 m
Initial temperature, T1 = 200C
Final temperature, T2 = 700C
Unknown value to find
Linear expansivity, α =?
Formula
α = e/L0(T2 – T1)
Solution
α = e/L0(T2 – T1) = 0.02/5(70-20) = 0.02/(5 x 50) = 0.02/250 = 0.00008 K-1
Which implies that
α = 8 x 10-5 K-1
Therefore, the linear expansivity of the invar is 8 x 10-5 per kelvin.
Question 3:
When 15 meters of a metallic rod is heated from 100C to 1100C, its length becomes 15.03 meters. What is the linear expansivity of the metal?
Data
Original length, L0 = 15 m
The increase in length, L = 15.03 m
Initial temperature, T1 = 100C
Final temperature, T2 = 1100C
Unknown value to find
Linear expansivity, α =?
Formula
α = (L – L0 )/L0(T2 – T1)
Solution
α = (15.03 – 15)/15(110 – 10) = 0.03/15(100) = 0.03/150 = 0.0002 K-1
Which implies that
α = 2 x 10-4 K-1
Therefore, the linear expansivity of the metal rod is 2 x 10-4 per kelvin.
Question 4:
The length of copper is 50 meters when it’s 200C. By how much will it expand when the temperature increases to 300C? [Take the linear expansivity of a copper to be 1.7 x 10-5 K-1]
Data: Extract your data from the above question.
Original length, L0 = 50 m
Linear expansivity, α = 1.7 x 10-5 K-1
Initial temperature, T1 = 200C
Final temperature, T2 = 300C
Unknown value to find
The increase in length, L =?
The formula we need to apply
L = L0(1 + αΔθ)
Solution
L = L0(1 + αΔθ) = L0[1 + α (Δθ = T2 – T1)] = 50 [1 + 1.7 x 10-5 (30 – 20 )]
And we will now have
L = 50 [ 1 + 0.000017 x 10 ]
Which will become
L = 50 [ 1 + 0.00017]
After adding 1 to 0.00017 you will then have
Increase in length, L = 50 x 1.00017 = 50.0085 m
Thus,
L = 50.0085 m
Therefore, the increase in length of the copper rod is 50.0085 meters. Thus, the rod added 0.0085 of length to its original size.
Question 5:
Compute the increase in length of 500 meters of a copper wire, when its temperature changes from 120C to 320C. The linear expansivity of a copper wire is 17 x 10-5 per degree celsius.
Data: Always extract your data from the question before you start solving the problem.
Original length, L0 = 500 m
Linear expansivity, α = 17 x 10-5 0C-1
Initial temperature, T1 = 120C
Final temperature, T2 = 320C
Unknown value to find
The increase in length, L =?
The formula to solve the problem is
L = L0(1 + αΔθ)
Solution
L = L0(1 + αΔθ) = L0 [1 + α (T2 – T1)] = 500 [1 + 17 x 10-5 (32 – 12)]
The above expression will give us
L = 500 [ 1 + 0.00017 x 20 ]
We will then obtain
L = 50 [ 1 + 0.0034]
we will then add 1 to 0.0034 to get
Increase in length, L = 500 x 1.0034 = 50.0034 m
Therefore,
L = 50.0034 m
Therefore, the increase in length of the copper wire is 50.0034 meters. Thus, the rod added 0.0034 of length to its original size.
Question 6
If an iron rail of 8 meters long are laid close up end to end when the temperature is 300C. What gap will be provided between consecutive rails when the temperature rises to 600C?
(Take linear expansivity of iron = 1.2 x 10-5 K-1)
Solution
Linear expansivity of iron, α = 1.2 x 10-5 K-1
Change in Temperature, Δθ = (60 K – 30 K) = 30 K
Original length of rail, L1 = 8 m
The increase in length due to an increase in temperature, = αL1Δθ
and by inserting our data into the above formula, we will have
The increase in length due to increase in temperature, = 1.2 x 10-5 x 8 x 30 = 0.003 m = 0.3cm
Hence, the gap that will be provided between consecutive rails is 0.3 centimetres
Question 7
Steel bars, each of length 3m at 290C are to be used for constructing a rail line. If the linear expansivity of steel is 1.0 x 10-5 K-1. Calculate the safety gap that must be left between successive bars if the highest temperature expected is 410C.
Answer:
The final answer to the above question is 3.6 x 10-4 m
Explanation
We will make ΔL subject of the formula from the equation α = ΔL /L0Δθ to obtain
ΔL = αL0Δθ
where ΔL = >
α = 1.0 x 10-5 K-1
L0 = 3m
Δθ = (θ2 – θ1) = (41 – 29) = 120C
Hence by inserting our data into the main formula ΔL = αL0Δθ, we will have
ΔL = αL0Δθ = 1.0 x 10-5 x 3 x 12 = 0.00036 m = 3.6 x 10-4 m
Therefore, the safety gap that must be left is 3.6 x 10-4 m.
Question 8
A metal rod of length 50 centimeters is heated from 400C to 800C. If the linear expansivity of the material is α, calculate the increase in length of the rod (in meters) in terms of α.
Answer
The final answer to the above question is 20α
Explanation
We will use the formula ΔL = αL0(θ2 – θ1) to solve the problem
Therefore, since
L0 = 50 cm = 0.5 m
θ1 = 400C
θ2 = 800C
Thus,
ΔL = αL0(θ2 – θ1) = α x 0.5 x (80 – 40) = α x 0.5 x 40 = 20α
Therefore, the increase in length of the rod is 20α
Question 9
A metal rod of length 100 cm, is heated through 1000C. Calculate the change in length of the rod. (Linear expansivity of the material of the rod is 3 x 10-5 K-1).
Answer
The final answer to the question is 0.3 cm
Explanation
We will apply the formula ΔL = αL0Δθ to find the change in length
ΔL = αL0Δθ = 3.0 x 10-5 x 100 x 100 = 0.3 cm
Question 10
A bridge made of steel is 600 m long. What is the daily variation in its length if the night-time and day-time temperature are 100C and 350C respectively. The linear expansivity of steel is 0.0000120C-1.
Answer
The final answer to the above question is 18 cm
Explanation
We will also use the same formula in question 9 (ΔL = αL0Δθ ) to solve this problem
ΔL = αL0Δθ = 0.000012 x 600 x 25 = 0.18 m = 18 cm
Question 11
A metal rod of length 40.00 cm at 200C is heated to a temperature of 450C. If the new length of the rod is 40.05 cm. Calculate its linear expansivity.
Answer
The final answer to this question is 5 x 10-5 K-1
Explanation
The formula α = (L – L0 )/L0(θ2 – θ1) will help us to find the linear expansivity of the metal rod.
Data
L = 40.05 cm
L0 = 40.00 cm
θ1 = 200C
θ2 = 450C
Thus, by putting our data into the formula
α = (L – L0 )/L0(θ2 – θ1) = (40.05 – 40.00) / 40.00 (45 – 20)
The above expression will become
α = 0.05 / (40 x 25) = 0.00005 = 5 x 10-5 K-1
Therefore, the linear expansivity of the metal rod is 5 x 10-5 per kelvin
Question 12
A brass rod is 2 m long at a certain temperature. What is the length for a temperature rise of 100K, if the expansivity of brass is 18 x 10-6 K-1
Answer
The final answer to the above question is 2.0036 meters
Explanation
We will make L subject of the formula from the formula α = (L – L0 )/L0Δθ
Thus, by inserting our numerical information from the question into the formula, we will get
18 x 10-6 = (L – 2)/ 2 x 100
Will give us
L – 2 = 18 x 10-6 x 200
The above expression will now become
L – 2 = 36 x 10-4
By collecting like terms, we will now have
L = 2 + 36 x 10-4 = 2.0036 m
Therefore, the length for a temperature rise of 100k will be 2.0036 meters.
Question 13
The linear expansivity of a metal P is twice that of another metal Q. When these materials are heated through the same temperature change, their increase in length is the same. Calculate the ratio of the original length of P to that of Q.
Answer
The final to the above question is Q = 1:2
Explanation
Data
The linear expansivity of Q = α
Linear expansvity of P = 2α
Original length for P = Lp
The original length at Q = LQ
Formula
We will use the formula α = ΔL /L0Δθ
Solution
At P, we will have
2α = ΔL /LPΔθ
At Q, we will have
α = ΔL /LQΔθ
The ratio of LP to LQ will be
LP : LQ
2α = ΔL /LPΔθ : α = ΔL /LQΔθ
Hence, the above expression will left us with
ΔL /2αΔθ : ΔL /αΔθ
Thus, we now have
1/2 : 1
After multiplying both sides by 2, we will be left with
Ratio of P to Q = 1:2
Question 14
The ratio of the coefficient of linear expansion of two metals α1/α2 is 3:4. When heated through the same temperature change, the ratio of the increase in lengths of the two metals, L1/L2 is 1:2, the ratio of the original lengths L1/L2 is
Answer
The final answer to the above question is 2:3
Explanation
Data
α1 = 3
α2 = 4
The change in temperature for the two rods is the same = Δθ = θ
L1 = 1
L2 = 2
α = ΔL /L0Δθ
Therefore,
L0 = ΔL /αΔθ
Therefore
At rod 1
L1 = 1 /3θ
At rod 2
L2 = 2 /4θ
The ratio of L1 to L2 = (1 /3θ) : (2 /4θ)
Therefore, our final answer is 2/3
How Different Materials Respond to Linear Expansivity
Metals and Linear Expansivity Metals typically have a higher linear expansivity compared to non-metals. This property is harnessed in various applications. For instance, bimetallic strips, which consist of two different metals bonded together, are used in thermostats to regulate temperature. As temperature changes, the two metals expand at different rates, causing the strip to bend and operate the thermostat.
Concrete and Linear Expansivity Concrete, a widely used construction material, also exhibits linear expansivity. Understanding this property is essential to prevent structural issues due to temperature changes. Bridges, for example, incorporate expansion joints that allow the material to expand or contract without causing damage.
Liquids and Gases Unlike solids, liquids and gases exhibit minimal linear expansivity. Their particles are more loosely held, and their volume changes with temperature are relatively larger than dimensional changes.
Applications of Linear Expansivity
Engineering and Infrastructure Linear expansivity plays a pivotal role in designing structures like bridges, roads, and buildings. Engineers consider how materials will respond to temperature variations to ensure long-term stability and safety.
Thermal Stress Management In industries where materials are subjected to extreme temperature changes, such as aerospace and manufacturing, understanding linear expansivity helps manage thermal stress. Components can be designed to accommodate expansion and contraction, reducing the risk of mechanical failure.
Cooking Utensils and Culinary Science In the kitchen, linear expansivity is employed in cooking utensils. For instance, metal spatulas can be used to flip pancakes because the metal expands with heat, creating a gap between the spatula and the food.
Frequently Asked Questions: Linear Expansivity
Q: Does every material expand the same way when heated?
A: No, different materials have varying linear expansivity coefficients, causing them to expand at different rates.
Q: Is linear expansivity always noticeable?
A: Not always. In many everyday scenarios, the changes in size due to linear expansivity are negligible and not easily observable.
Q: How is linear expansivity related to temperature?
A: Linear expansivity is directly proportional to temperature changes. As temperature increases, the material’s dimensions also increase.
Q: Can linear expansivity be negative?
A: Yes, in some cases, materials can contract with increasing temperature, resulting in a negative linear expansivity coefficient.
Q: Are there materials with zero linear expansivity?
A: No, all materials exhibit some degree of linear expansivity, although the extent may vary.
Q: Why is linear expansivity important in scientific research?
A: Linear expansivity is a critical factor when studying material behavior, especially when it comes to predicting how substances will respond to temperature fluctuations.
I hope you are now familiar with linear expansivity: definition and calculations. Drop a comment if there is anything you would like me to explain further.
You may also like to read:
How to Derive the Formula For Increase in Volume
Also How to Calculate Cubic Expansivity with Examples
How to Calculate the Relative Density of a Liquid
Linear Expansivity Definition and Calculations
Sources: