Skip to content

The amplitude of a lightly damped oscillator decreases by 3.0% during each cycle.

Question

The amplitude of a lightly damped oscillator decreases by 3.0% during each cycle. What percentage of the mechanical energy of the oscillator is lost in each cycle?

Solution

[Answer: The percentage of the mechanical energy of the oscillator lost in each cycle is 6%]

We can use two different methods (explanations) to arrive at our answer.

Below is the video explanation of the first method:

Explanation 1:

Below is a picture explanation of the first method:

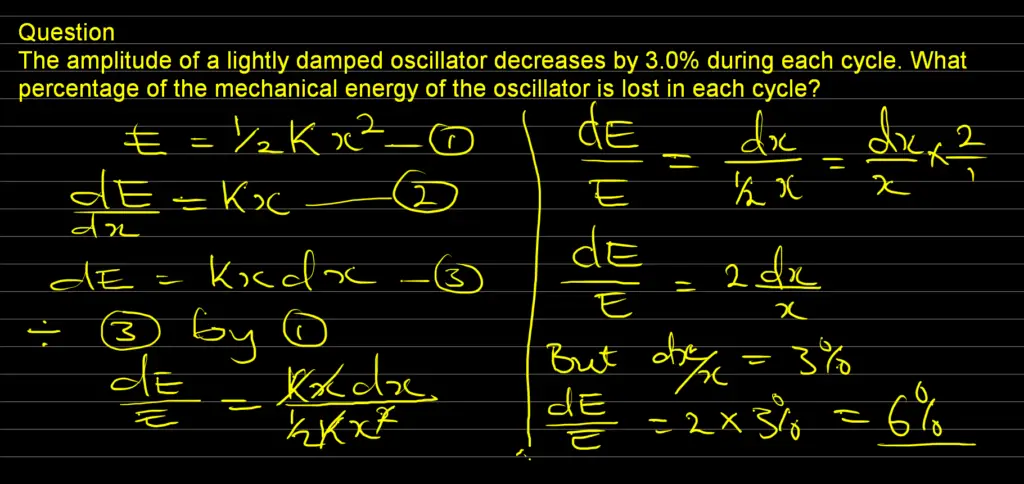
The amplitude of a lightly damped oscillator decreases by 3.0% during each cycle. What percentage
The amplitude of a lightly damped oscillator decreases by 3.0% during each cycle. What percentage

This is the simplest method of solving the problem. We need to understand that the energy of a damped oscillator is defined by the formula, E = (1/2)Kx2

Therefore, by differentiating with respect to x, we will have:

dE/dx = Kx

After cross multiplication, we will have dE = Kxdx

Hence, we will now divide dE by E to obtain dE/E = [kxdx] / [(1/2)Kx2]

The above equation will now give us: dE/E = 2dx / x = 2 (dx/x)

and dx/x = 3%

Therefore dE/E = 2 * 3% = 6%

This shows the percentage loss of the mechanical energy of the oscillator in each cycle is 6%

Explanation 2:

To determine the percentage of the mechanical of the oscillator that is lost, we need to understand the damping ratio. The damping ratio is the decrease in the percentage of the amplitude of the oscillator for each cycle.

Mechanical energy is of two types. We have kinetic and potential energy.

The formula for calculating the Potential energy of a damped oscillator is

P.E=(1/2)kx2

Since we are talking about the percentage of mechanical energy lost in each cycle, we are going to consider applying the formula below to solve the problem.

[ P.Ef=(1/2)kxf2 ] / [ P.Ei=(1/2)kxi2 ]

where

P.Ef = final potential energy

k = Constant

xf = final displacement

P.Ei = Initial potential energy

xi = initial displacement

Therefore, the expression [ P.Ef=(1/2)kxf2 ] / [ P.Ei=(1/2)kxi2 ] will become

P.Ef / P.Ei = xf2 / xi2

Which can also be rewritten as

P.Ef / P.Ei = (xf / xi)2

and if we assume xi = 100% while xf = 97% [we subtracted 47 from 50 due to the 3% decrease]

We will have

P.Ef / P.Ei = (97/100)2 = (0.97)2 = 0.9409% and can be approximated into 0.94%

and we can see that

P.Ef / P.Ei = 1 – 0.94 = 0.06

Therefore, after multiplying 0.06% by 100. We will obtain

P.Ef / P.Ei = 6%

Hence, the percentage of the mechanical energy of the oscillator lost in each cycle is 6%

Reference:

How to Calculate Kinetic Energy

How to Calculate Work Done in Physics

Sources:

Studysmarter