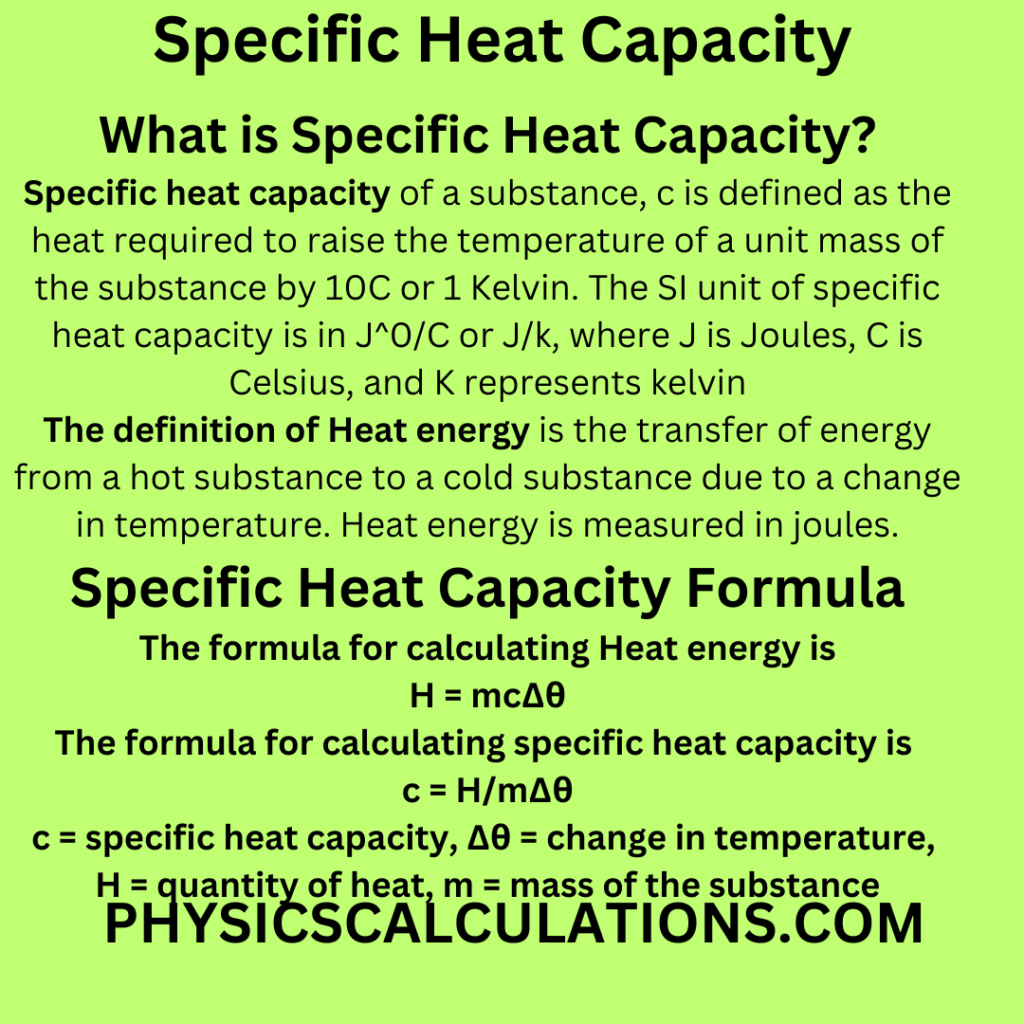
What is Specific Heat Capacity?
Definition of Specific Heat Capacity (SHC): Specific heat capacity of a substance, c is defined as the heat required to raise the temperature of a unit mass of the substance by 10C or 1 Kelvin. The SI unit of specific heat capacity is in J0/C or J/k, where J is Joules, C is Celsius, and K represents kelvin. We can equally measure SHC Joules per kilogram per kelvin (Jkg-1K-1). In this article, you will learn how to calculate specific heat capacity.
Additionally, we can equally define specific heat capacity as the quantity of heat that is needed to increase the temperature of a unit mass of a substance by one kelvin or one-degree Celsius.
A video on How to calculate specific heat capacity by electrical method
The definition of Heat energy is the transfer of energy from a hot substance to a cold substance due to a change in temperature. Heat energy is measured in joules.
Heat Capacity Formula
The formula for calculating Heat energy is
H = mcΔθ
And we can also write it as
H = mc(θ2 – θ1) because Δθ = (θ2 – θ1)
Where H = quantity of heat
m = mass of the object
Δθ = change in temperature [we measure the change in temperature in degree Celsius or kelvin]
c = specific heat capacity = constant
Specific Heat Capacity Formula
The formula for calculating specific heat capacity is
c = H/mΔθ
Additionally, since the quantity of heat, H = mcΔθ
and the formula for thermal capacity, Tc = mc
Thus,
Quantity of heat, H = TcΔθ [Where Tc = Thermal capacity]
and Δθ = θ2 – θ1
H = Tc (θ2 – θ1)
Additionally,
Thermal capacity is measured in K-1
Table for Specific Heat Capacity of Substances
Below is a table for the specific heat capacity of substances to help understand how to calculate specific heat capacity:
| Material | Specific Heat Capacity (c) |
| Aluminum | 900 Jkg-1K-1 |
| Brass | 380 Jkg-1K-1 |
| Copper | 390 Jkg-1K-1 |
| Glass | 670 Jkg-1K-1 |
| Iron | 460 Jkg-1K-1 |
| Lead | 129 Jkg-1K-1 |
| Platinum | 135 Jkg-1K-1 |
| Silver | 234 Jkg-1K-1 |
| Tin | 226 Jkg-1K-1 |
| Zinc | 384 Jkg-1K-1 |
| Alcohol | 2520 Jkg-1K-1 |
| Glycerine | 2430 Jkg-1K-1 |
| Ice | 2100 Jkg-1K-1 |
| Mercury | 140 Jkg-1K-1 |
| Paraffin oil | 2130 Jkg-1K-1 |
| Turpentine | 1760 Jkg-1K-1 |
| Water | 4200 Jkg-1K-1 |
Solved Problems: How to Calculate Specific Heat Capacity
Here are solved problems of how to calculate specific heat capacity
Problem 1
A copper rod with heat capacity 585JK-1 is heated until its temperature changes from 350C to 800C. Calculate the quantity of heat supplied to the rod. If the specific heat capacity of copper is 390 Jkg-1K-1, find the mass of the rod.
Solution
Data:
We start solving the problem by reading the question twice, and extracting our data while reading for the third time. This method will help us to understand the question, and apply the best formula that can help us to solve the problem.
Thermal capacity, Tc = 585JK-1
Initial temperature, θ1 = 800C = 273K + 80 = 353K
Final temperature, θ2 = 350C = 273K + 35 = 308K
The specific heat capacity of copper, c = 390 Jkg-1K-1
Now we apply the formula which says:
H = Tc (θ2 – θ1)
After substituting our data into the above formula, we will now have:
H = 585 (353 – 308) = 585 x 45 = 26,325J = 26KJ
To find the mass of the rod, we apply the formula Tc = mc
Now, we substitute our data into the above formula
585 = m x 390
We can go ahead to make the m subject of the formula by dividing both sides by 390
585/390 = (m x 390)/390
And the above expression will become
m = 585/390 = 1.5 kg
Therefore, the mass of the rod is 1.5 kg
Problem 2
What is the quantity of heat required to raise the temperature of 300 g of the aluminum cube from 300C to 700C? (Specific heat capacity of aluminum = 900Jkg-1K-1).
Solution:
Data:
We start solving the problem by reading the question twice, and extracting our data while reading for the third time. This method will help us to understand the question, and apply the best formula that can help us to solve the problem.
Mass of aluminium cube, m = 300 g = (300/1000 ) kg = 0.3 kg
Initial temperature, θ1 = 300C = 273K + 30 = 303K
Final temperature, θ2 = 700C = 273K + 70 = 343K
Specific heat capacity of aluminum = 900Jkg-1K-1
We can now apply the formula, H = mcΔθ
H = mc(θ2 – θ1) [where Δθ = (θ2 – θ1) ]
Apply your data to the above formula to get
H = mc(θ2 – θ1) = 0.3 x 900 x (343 – 303) = 0.3 x 900 x 40 = 10,800J or 11KJ
Therefore, the quantity of heat required to raise the temperature of the aluminum cube is 11 – Kilojoules
Problem 3
Calculate the change in temperature when 3000-Joules of heat is supplied to 500 grams of water
Solution
Data:
We start solving the problem by reading the question twice, and extracting our data while reading for the third time. This method will help us to understand the question, and apply the best formula that can help us to solve the problem.
Quantity of heat, H = 3000J
Mass of water, m = 500g = (500/1000)kg = 0.5kg
The specific heat capacity of water, c = 4,200 Jkg-1K-1
Change in temperature, Δθ =?
We can now apply the formula, H = mcΔθ and then make Δθ subject of the formula by dividing both sides by mc
H/mc = mcΔθ/mc
and mc will cancel each other on the left-hand side (LHS) leaving us with
Δθ = H/mc
Therefore, when we apply our data to the above formula, we will have
Δθ = 3000 / (0.5 x 4,200) = 3000 / 2,100 = 1.430C
and 1.430C can be converted into kelvin by performing the following operation = 273k + 1.43 = 274.43K
Therefore, the change in temperature is 1.430C (degree-celsius) or 274.43-Kelvin
Problem 4
Calculate the quantity of heat required to raise the temperature of 5kg of copper from 250C to 1000C. [The specific heat capacity of a copper = 390Jkg-1K-1]
Solution
Data:
We start solving the problem by reading the question twice, and extracting our data while reading for the third time. This method will help us to understand the question, and apply the best formula that can help us to solve the problem.
Mass of the copper, m = 5kg
Initial temperature, θ1 = 250C = 273K + 25 = 298K
Final temperature, θ2 = 700C = 273K + 100 = 373K
Specific heat capacity of aluminum = 390Jkg-1K-1
We can now look into the formula that can help us solve the problem
H = mcΔθ
and we can now write the above formula as
H = mc(θ2 – θ1) [where Δθ = (θ2 – θ1) ]
By substituting our data into the above formula, we will get
H = 5 x 390 x (373 – 298) = 5 x 390 x 75 = 146,250J = 146kJ
Therefore, the quantity of heat required to raise the temperature of copper is 146 – Kilojoules.
Problem 5
An electric heater of 60 Watts is used to heat a metal block of mass 20-kilogram for 5 minutes. Calculate the metal block’s specific heat capacity if the temperature rise is 200C.
Solution
Data
Power of the electric heater, P = 60 Watts
Mass of the metal block = 20kg
Time, t = 5 minutes = 60 x 5 = 300 seconds
Rise in temperature, Δθ = 200C
Specific heat capacity of the metal block, c =?
The formula for calculating the specific heat capacity of a substance by an electrical method is IVt = mcΔθ
And in the case of the question, we are solving now, we are dealing with the power of 60W.
Since the formula for electric Power = current x voltage = IV [ Power, P = IV]
We can now rewrite our formula IVt = mcΔθ as Pt = mcΔθ
Therefore, the right formula that fits into our data, and can help us solve the problem is Pt = mcΔθ
Now, we substitute our data into Pt = mcΔθ
Thus,
60 x 300 = 20 x c x 20
And we have
18,000 = 400 x c
we can now divide both sides by 400 to get
c = 18,000/400 = 45Jkg-1K-1
The specific heat capacity of the metal block, c is 45Jkg-1K-1
Problem 6
A waterfall is 600m high, if the water retains 65% of the heat generated at the end of the fall, calculate the change in temperature due to the fall. [Specific heat capacity of water, c = 4,200Jkg-1K-1].
Solution
Data:
Height of the waterfall = 600m
Specific heat capacity of water, c = 4,200Jkg-1K-1
The change in temperature, Δθ =?
and we can apply the formula mgh = mcΔθ to solve the problem
Therefore, after making Δθ subject of the formula, we will have
Δθ = gh/c [ where g = gravitational acceleration = 9.8ms-2]
and we can substitute our data into the above formula to get
Δθ = (9.8 x 600)/4,200 = 5,880/4,200 = 1.40C
Therefore, the change in temperature is 1.40C
Problem 7
The hot water tap of a bath delivers water at 750C at a rate of 10 kg per minute. While a cold water tap of the birth delivers water at 250C at the rate of 22 kg per minute. If both taps are left on for 4 minutes, calculate the final temperature of the bath water, ignoring heat losses to the environment.
Solution
Data:
For the heat of a hot water tap
mass in 5 minutes = 10kg x 5 = 50kg
Initial temperature, θ1 = θ
Final temperature θ2 = 750C
Specific heat capacity of water, c = 4,200Jkg-1K-1
Therefore, the quantity of heat for hot water, H = mc(θ2 – θ1) = 50 x 4,200 x (75 – θ)
For the heat of a cold water tap
mass in 5 minutes = 22kg x 5 = 110kg
Initial temperature, θ1 = 250C
Final temperature θ2 = θ
Specific heat capacity of water, c = 4,200Jkg-1K-1
Therefore, the quantity of heat for cold water, H = mc(θ2 – θ1) = 110 x 4,200 x (θ – 25)
We can now see that the quantity of heat for hot water is equal to the quantity of heat for cold water
Hence, 50 x 4,200 x (75 – θ) = 110 x 4,200 x (θ – 25)
We can simplify the above expression by making θ subject of the formula
Therefore, our problem now becomes
(75 – θ) = (110/50) x (θ – 25)
This is now equal to
(75 – θ) = 2.2 x (θ – 25)
and we will have
75 – θ = 2.2θ – 55
We can further simplify the above expression into
75 + 55 = 2.2θ + θ
Thus, 130 = 3.2θ
and θ = 130/3.2 = 40.6250C = 40.60C
Therefore, the final temperature of the water bath is 40.60C
Problem 8
An electric heater, rated 10V, 12W, fitted into a metal block supplies heat to the block of mass 1kg and a specific heat capacity of 460Jkg-1K-1. Calculate the rise in temperature in the block if the current flow for 5 minutes.
Solution
Data:
Power = 12 Watts
Time, t = 15 minutes = 5 x 60 seconds = 300s
Mass of the block = 1kg
The specific heat capacity of the metal, c = 460Jkg-1K-1
Rise in temperature, Δθ =?
We can now apply the formula Pt = mcΔθ to get
12 x 300 = 1 x 460 x Δθ
and we will have
3,600 = 460 x Δθ
By making Δθ subject of the formula, we will have
Δθ =3,600/460 = 7.830C
Therefore the change in temperature is 7.830C
Drop a comment on any question regarding how to calculate specific heat capacity so that we can assist you.
You may also like to check:
How to Calculate Dimension in Physics
How to Calculate Escape Velocity of a Satellite
References:
Check our websites:
Wokminer – Apply for any type of job at any location
Arewagist – For health and nutrition