Root-Mean Square Definition in Physics
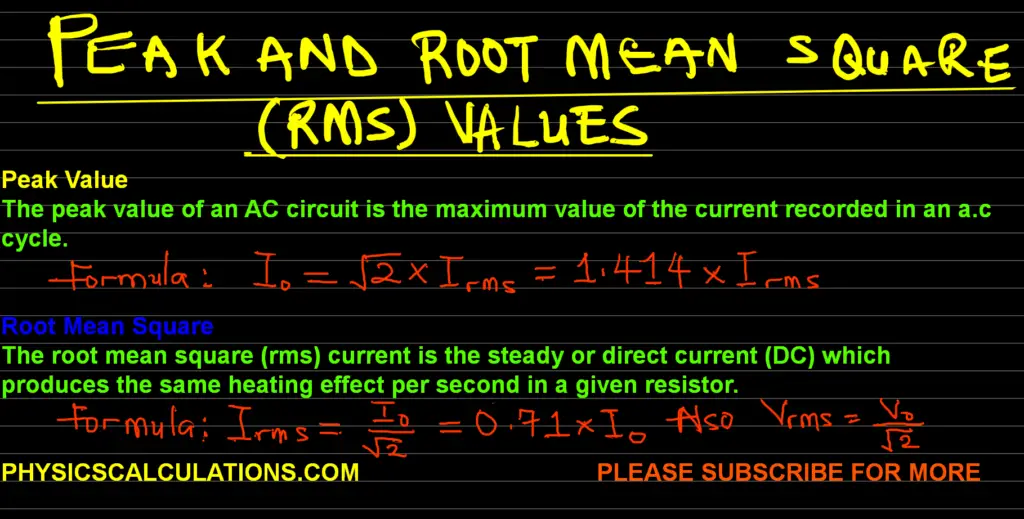
Definition: The root mean square (rms) current in physics is the steady or direct current (DC) which produces the same heating effect per second in a given resistor. The symbol for root mean square value is Irms.
The formula for calculating the root mean square value is Irms = I0 / √2 = 0.71 x I0
Where I0 is the peak current.
Peak Current (I0): Is the peak value of an alternating current (AC) circuit which is the maximum value of the current recorded in an AC cycle. The formula for peak current is: I0 = √2 x Irms = 1.414 x Irms
We also have Root mean square voltage (Vrms). The formula for root mean square voltage is Vrms = V0 / √2 = 0.71 x V0. Where V0 is the peak voltage value.
It is also important to know that we can represent alternating current and alternating voltages by:
Alternating current, I = I0sin2πft = I0sinωt
And the alternating voltage, V = V0sin2πt = V0sinωt
From the above symbols, we have:
I = Instantaneous current (si unit = Ampere(A))
I0 = Peak or maximum current (si unit = Ampere(A))
f = frequency (si unit = Heartz (Hz))
t = time (s) (si unit = seconds (s))
ωt = θ = phase angle of current or voltage
V = voltage
V0 = Peak voltage
Root Mean Square Solved Problems
Here are a few problems to help you understand how to calculate rms in physics:
Problem 1
The current, I in an a.c circuit is given by the equation: I = 30sin100πt where t is the time in seconds. Deduce the following from this equation:
a. Frequency of the current
b. Peak value of the current
c. rms value of the current
Solution
Data: The information from the question
We were given I = 30sin100πt, and by comparing it with I = I0sin2πft we will obtain
a. Frequency, f as 100πt = 2πft
t and π will cancel each other leaving us with
f = 100 / 2 = 50 Hz
Therefore, the frequency is 50 Hz
b. The peak current is already available from the equation I = 30sin100πt.
Therefore: I0 = 20 A
c. We can calculate the rms value by applying the formula: Irms = I0 / √2 = 0.71 x I0
Thus, Irms = 0.71 x I0 = 0.71 x 30 = 21.3 A
Therefore, the rms value is 21.3 A.
Problem 2
Calculate the peak voltage of a main supply of rms value 220V.
Solution
We have Vrms = 220 volts
Applying the formula: V0 = Vrms x 1.414 = 220 x 1.414 = 311.08 Volts
Problem 3
The current through a resistor in an a.c circuit is given as 2sinωt. Determine the d.c equivalent of the current.
Solution
We have I = I0sinωt. Therefore, the peak current is I0 = 2A
The direct current (DC) equivalent of a.c is the root mean square current Irms
Therefore, Irms = I0 / √2 = 2 / √2
We will now multiply both the numerator and denominator by √2 to obtain
Irms = (2/√2) x (√2/√2) = (2√2) / (√2×2) = (2√2) / 2 = √2 Ampere
Problem 4
If an a.c is represented by I = I0sinωt. Calculate the instantaneous value of such a current, if in a circuit it has rms value of 15.0 A when it is phase angle is 300.
Solution
The equation available to us is I = I0sinωt. And ωt = 300, Irms = 15.0 A
To find I0 will use the formula: I0 = 1.414 x Irms = 1.414 x 15 = 21.21 A
Therefore, we will now apply the equation: I = I0sinωt = 21.21 x sin300 = 10.605 Ampere
Problem 5
In an a.c circuit the peak of the potential difference 180V. What is the instantaneous potential difference when it has reached (1/8)th of a cycle?
Solution
We have a formula for instantaneous potential difference as: V = V0sinωt
where V = ?, V0 = 180V,
ωt = θ = phase angle of the voltage = (1/8)th of a cycle = (1/8) x 3600 = 450 [Remember that 1 cycle = 3600]
sin450 =
Thus, to find V, we will substitute our data into the formula:
V = V0sinωt = 180 x sin450 = 180 x √2/2 = 90√2 = 127 Volts.
Problem 6
The instantaneous value of the induced e.m.f as a function of time is ε = ε0sinωt where ε0 = is the peak value of the e.m.f. The instantaneous value of the e.m.f, one-quarter of the period is
Solution
ωt = θ = phase angle of the e.m.f
Therefore, ωt = θ = one-quarter of the period is = (1/4) x 3600 = 900
By applying the equation available to us from the question, we will obtain:
ε = ε0sinωt = ε0sin900 = ε0
You may also like to read: