How to Calculate Electric Current
To calculate electric current, use the formula I=Q/t, or I = V/R or I = P/V depending on the problem you are dealing with. Where (I) is current, (Q) is charge, V is voltage (potential difference), R is resistance, P is the power, and (t) is time. Measure charge flow over time for accurate results. Here is a step-by-step guide on how to calculate electric current when the formula I=Q/t is involved:
| Step | Description | Formula |
|---|---|---|
| 1 | Measure the charge flowing through a | |
| conductor (Q). | ||
| 2 | Measure the time (t) during which the charge | |
| flows. | ||
| 3 | Calculate electric current (I): Note: The unit of current Ampere | I = Q/t |
Note:
- The unit of current (I) is the ampere (A), representing the flow of electric charge. It is defined as one coulomb of charge passing per second.
- Ensure consistent units for charge (coulombs, C) and time (seconds, s) in the calculation.
Methodology
Calculating electric current involves following a series of steps to obtain accurate results. These steps include gathering the necessary data, identifying the unknowns, utilizing the appropriate formula, and finally arriving at a solution. Let’s break down each step in detail.
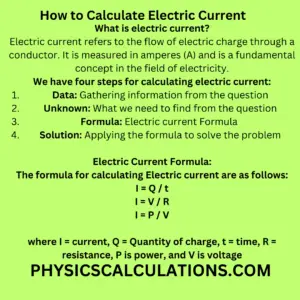
Step 1: Data
The first step in calculating electric current is collecting the relevant data. This data typically consists of two key pieces of information: the voltage (V) applied across a circuit and the resistance (R) within the circuit. Voltage represents the potential difference that drives electric current, while resistance impedes the flow of current. By knowing these values, we can proceed to the next step.
Step 2: Unknown
After gathering the data, we identify the unknown value that needs to be determined. In most cases, this unknown is the electric current (I) flowing through the circuit. By measuring the voltage and resistance, we can use the appropriate formula to find the value of electric current.
Step 3: Formula
To calculate electric current, we employ Ohm’s Law, named after the German physicist Georg Simon Ohm. Ohm’s Law states that the electric current (I) flowing through a conductor is directly proportional to the voltage (V) across the conductor and inversely proportional to the resistance (R) of the conductor. Mathematically, Ohm’s Law can be expressed as:
I = V / R
This simple formula allows us to determine the electric current in a circuit when we know the voltage and resistance.
Step 4: Solution
Once we have the formula and the data, we can substitute the values into the formula and solve for the electric current (I). By dividing the voltage (V) by the resistance (R), we obtain the electric current flowing through the circuit.
Now that we have covered the methodology, let’s move on to solving some practice problems to solidify our understanding.
Solve Problems
Problem 1:
Given a circuit with a voltage of 12 volts (V) and a resistance of 4 ohms (Ω), what is the electric current flowing through the circuit?
Solution:
To find the electric current (I), we can use Ohm’s Law:
I = V / R
Substituting our data into the formula above, we will have:
I = 12V / 4Ω
Therefore, the current, I = 3A
Therefore, the electric current flowing through the circuit is 3 amperes (A).
Problem 2:
Suppose a circuit has a voltage of 24 volts (V) and an electric current of 6 amperes (A). What is the resistance in the circuit?
Solution:
Rearranging Ohm’s Law, we can solve for resistance (R):
R = V / I
Substituting our data into the above formula, we will obtain:
R = 24V / 6A
Therefore,
R = 4Ω
Thus, the resistance in the circuit is 4 ohms (Ω).
Problem 3:
If the electric current in a circuit is 0.5 amperes (A) and the resistance is 10 ohms (Ω), what is the voltage across the circuit?
Solution:
Using Ohm’s Law, we can determine the voltage (V):
V = I x R = 0.5A x 10Ω = 5V
The voltage across the circuit is 5 volts (V).
Problem 4:
You have a circuit with a voltage of 18 volts (V) and an electric current of 2 amperes (A). Calculate the resistance in the circuit.
Solution:
Applying Ohm’s Law, we can find the resistance (R):
R = V / I = 18V / 2A = 9Ω
The resistance in the circuit is 9 ohms (Ω).
Problem 5:
Consider a circuit with a resistance of 5 ohms (Ω) and an electric current of 0.2 amperes (A). What is the voltage across the circuit?
Solution:
Using Ohm’s Law, we can determine the voltage (V):
V = I x R = 0.2A x 5Ω = 1V
The voltage across the circuit is 1 volt (V).
How to Calculate Electric Current
The Basic Formula: I = Q / t
To calculate electric current, we can use the basic formula: I = Q / t. Here, I represents the electric current, Q denotes the charge flowing through the conductor, and t signifies the time taken for the charge to pass through.
Let’s break it down further:
- I: Electric current in amperes (A)
- Q: Electric charge in coulombs (C)
- t: Time in seconds (s)
To find the electric current, we need to know the amount of charge passing through the conductor and the time it takes to pass. By dividing the charge by the time, we can determine the electric current flowing through the circuit.
Example Calculation
Let’s illustrate the calculation of electric current using an example:
Suppose a circuit has a charge of 40 coulombs passing through it in 10 seconds. To find the electric current, we can use the formula I = Q / t.
I = 40 C / 10 s
I = 4 A
Therefore, the electric current flowing through the circuit is 4 amperes.
Understanding Electric Current in Series and Parallel Circuits
Series Circuits
In a series circuit, components are connected in a sequence, forming a single pathway for the current. The current flowing through each component remains the same, while the voltage may vary across each component.
To calculate the total current in a series circuit, we can simply add up the individual currents of each component. This means that the total current remains constant throughout the circuit.
Parallel Circuits
In a parallel circuit, components are connected side by side, creating multiple pathways for the current. Each component in a parallel circuit has the same voltage across it, while the current may differ.
To calculate the total current in a parallel circuit, we add up the individual currents using the formula Itotal = I1 + I2 + … + In, where Itotal is the total current and I1, I2, and In represent the currents through each component.
How to Calculate Electric Current in Real-Life Scenarios
Now that we’ve covered the basics of calculating electric current, let’s explore some real-life scenarios where this knowledge becomes valuable.
Scenario 1: Calculating Current in a Home Circuit
Imagine you want to determine the current flowing through a circuit in your home. Here are the steps to follow:
- Identify the circuit: Determine the circuit for which you want to calculate the current. For example, let’s consider a lighting circuit.
- Determine the total power: Find the total power consumption of all the devices connected in that circuit. You can usually find this information on the devices or consult the device’s user manual.
- Calculate the current: Divide the total power by the voltage of the circuit using the formula P = V x I, where P is power, V is voltage, and I is current. Rearranging the formula, we get I = P / V. By substituting the known values, you can calculate the current.
Scenario 2: Calculating Current in an Automotive Circuit
If you’re an automotive enthusiast or mechanic, knowing how to calculate current in an automotive circuit is vital. Here’s a step-by-step guide:
- Identify the circuit: Determine the specific circuit in the vehicle that you’re interested in. For example, let’s consider the circuit for the headlights.
- Determine the total power: Find the total power consumption of the headlights. This information is usually available in the vehicle’s manual or can be obtained from the manufacturer.
- Calculate the current: Use the formula P = V x I, where P is power, V is voltage, and I is current. Rearrange the formula to solve for I: I = P / V. Plug in the known values and calculate the current.
Summary
In summary, calculating electric current involves four key steps: gathering the necessary data (voltage and resistance), identifying the unknown value (electric current), utilizing Ohm’s Law to determine the formula, and substituting the values to obtain the solution. By following these steps, we can accurately calculate electric current in various circuits.
Evaluation
Calculating electric current is an essential skill in the field of electricity and electronics. Understanding the relationship between voltage, resistance, and current enables us to analyze and design electrical circuits effectively. By learning the methodology and applying Ohm’s Law, we can solve a wide range of problems and gain a deeper understanding of electric current.
Frequently Asked Questions
Q1: What is electric current?
Electric current refers to the flow of electric charge through a conductor. It is measured in amperes (A) and is a fundamental concept in the field of electricity.
Q2: What is voltage?
Voltage, also known as electric potential difference, represents the electrical potential energy per unit charge. It is measured in volts (V) and determines the force that drives electric current.
Q3: What is resistance?
Resistance is the opposition offered by a material or component to the flow of electric current. It is measured in ohms (Ω) and determines how much current flows through a circuit for a given voltage.
Q4: What is Ohm’s Law?
Ohm’s Law states that the electric current (I) flowing through a conductor is directly proportional to the voltage (V) across the conductor and inversely proportional to the resistance (R) of the conductor. The relationship is expressed mathematically as I = V / R.
Q5: Can electric current flow in a vacuum?
No, electric current requires a medium or conductor to flow. In a vacuum, there are no free electrons to carry the charge, so current cannot pass through.
Q6: How is electric current measured?
Electric current is measured using an ammeter, which is connected in series with the circuit. The ammeter measures the flow of charge through the circuit and provides the current reading in amperes (A).
Q6: What units are used to measure electric current?
Electric current is measured in amperes (A). This unit is named after the French physicist André-Marie Ampère, who made significant contributions to the field of electromagnetism.
Q7: How does the thickness of a wire affect electric current?
The thickness of a wire, also known as its cross-sectional area, affects the electric current. Thicker wires have lower resistance, allowing a greater amount of current to flow through them. In contrast, thinner wires offer more resistance and restrict the flow of current.
Q8: Does electric current always flow in a closed loop?
Yes, electric current always flows in a closed loop. It requires a complete circuit for the current to flow continuously. If the circuit is broken, the flow of current stops.
Q9: What is the difference between AC and DC current?
AC (alternating current) and DC (direct current) are two different types of electric current. AC current periodically changes direction, commonly used in household power supply. DC current flows continuously in one direction, often found in batteries and electronic devices.
Q10: Can electric current be negative?
Yes, electric current can be negative. The sign of the current indicates the direction of the flow of electrons. Positive current flows in one direction, while negative current flows in the opposite direction.
Assignment
Now it’s time for you to apply your knowledge of calculating electric current. Solve the following problems:
- Given a circuit with a voltage of 9 volts (V) and an electric current of 3 amperes (A), what is the resistance in the circuit?
- Suppose a circuit has a resistance of 8 ohms (Ω) and a voltage of 24 volts (V). Find the electric current flowing through the circuit.
- If the electric current in a circuit is 0.2 amperes (A) and the voltage is 12 volts (V), what is the resistance in the circuit?
- You have a circuit with a resistance of 6 ohms (Ω) and a voltage of 30 volts (V). Find the electric current flowing through the circuit.
- Consider a circuit with an electric current of 0.5 amperes (A) and a resistance of 20 ohms (Ω). Calculate the voltage across the circuit.
After solving the problems, check your answers below:
- The resistance in the circuit is 3 ohms (Ω).
- The electric current flowing through the circuit is 3 amperes (A).
- The resistance in the circuit is 60 ohms (Ω).
- The electric current flowing through the circuit is 5 amperes (A).
- The voltage across the circuit is 10 volts (V).
Remember, practice makes perfect!
Conclusion
In conclusion, understanding how to find electric current is essential for anyone working with electricity. By following the four-step methodology of gathering data, identifying the unknown, utilizing the formula (Ohm’s Law), and finding the solution, we can determine the electric current in a circuit accurately.
Remember to pay attention to the units and follow the correct formulas to obtain reliable results. Now you have the knowledge and skills to calculate electric current confidently.
I hope this article satisfies your curiosity about how to calculate electric current.
You may also like to read: