Question 1
Calculate the Energy of a Photon of Each Frequency 7.5 x 105 Hz
Here is how to calculate the energy of a photon of each frequency.
Data: You will need to first extract the important values from the question
The frequency, f = 7.5 x 105 Hz
Planck’s constant, h = 6.63 x 10-34 Js
Unknown value to find:
The energy of the photon, E =?
Formula:
Energy of the photon, E = hf
Solution:
E = hf = 6.63 x 10-34 x 7.5 x 105 = 4.9725 x 10-28 J
Therefore, we have obtained
E = 4.9725 x 10-28 J
Therefore, the energy of a photon of each frequency is 4.9725 x 10-28 Joules. We can further convert our answer to electron-volt (eV).
How to calculate the energy of a photon
Calculating the energy of a photon depends on the data available in the question you want to solve. Here are available methods to calculate the energy of a photon:
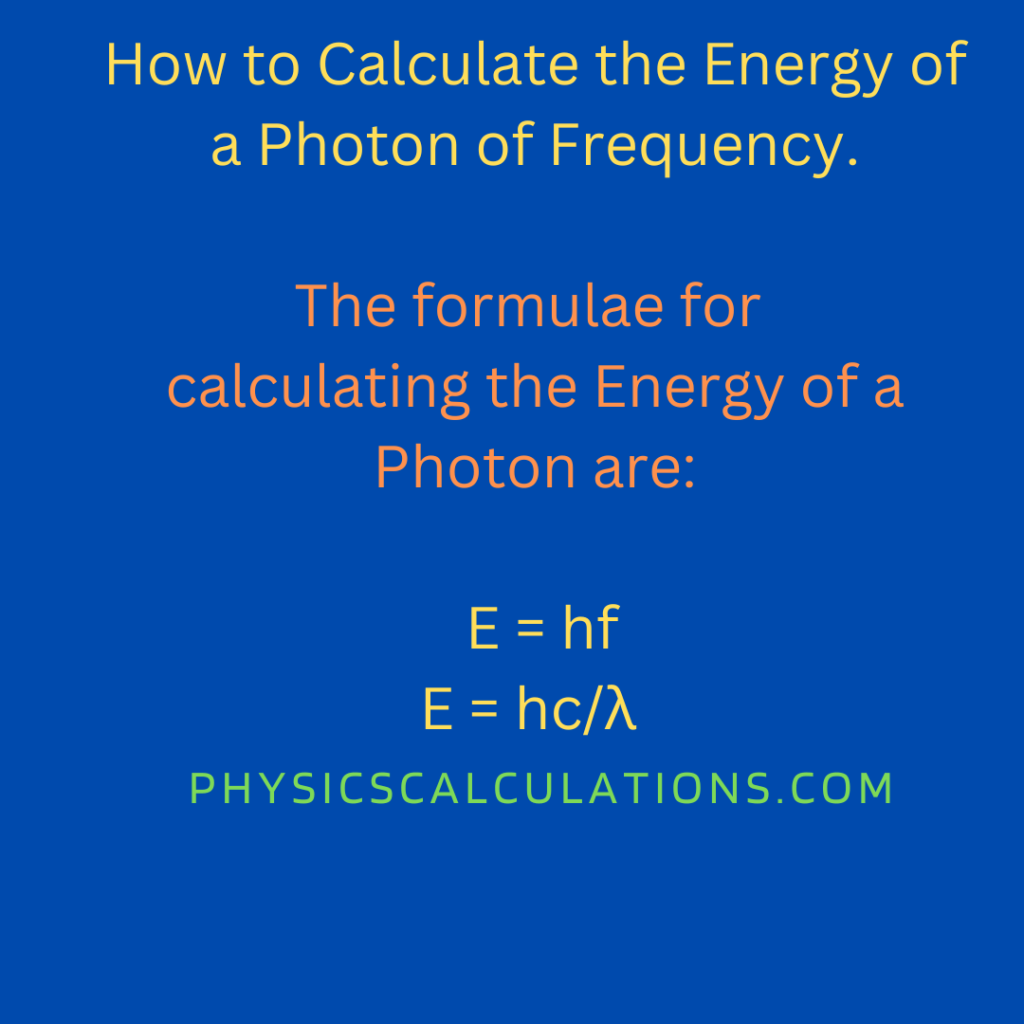
Method 1
- The first method to calculate the energy of a photon is by applying the formula that says:
E = hf
Where
E = Energy of a photon
h = Planck’s constant = constant = 6.23 x 10-34 Js
f = Frequency
In this case, the frequency is provided from the question. You will multiply Planck’s constant by the frequency to obtain the value of the energy of the photon in joules (J) or electronvolt (eV).
For instance, if f = 5 x 107 s-1
The energy of the photon will become
E = hf = 6.23 x 10-34 Js x 5 x 107 s-1 = 3.115 x 10-28 J
Note: The units of frequency are Hertz (Hz) and per second (s-1)
Method 2
2. This method helps us to understand how to calculate energy of a photon using wavelength. We will apply the formula below to calculate the energy of the photon;
E = hc/λ
Proof: This is because f = c/λ
and since we have E = hf
we can substitute the values of f into the above expression to obtain
E = hf = h x c/λ = hc/λ
The formula above helps us to calculate the energy of a photon of electromagnetic radiation.
Where
E = energy of the photon
h = Planck’s constant = constant = 6.23 x 10-34 Js
c = Speed of light = constant = 299,792,458 metres per second = 3 x 108 m/s (after approximation)
λ = wavelengths
For example, if the value of the wavelength is 8 x 10-9 meters, the energy of the photon will become
E = hc/λ = (6.23 x 10-34 x 3 x 108)/8 x 10-9 = 1.869 x 10-25 / 8 x 10-9 = 2.336 x 10-17 Joules
Question 2
Calculate the Energy of a Photon of Radiation with a Wavelength of 6.4 x 10-7 m
We will apply the formula E = hc/λ
and since we have a wavelength of 6.4 x 10-7 m
we will now write
E = hc/λ = (6.23 x 10-34 x 3 x 108)/6.4 x 10-7 =1.869 x 10-25 / 6.4 x 10-7 = 2.9203125 x 10-19 Joules
Therefore, the energy of a photon of radiation with a wavelength of 6.4 x 10-7 meters is 2.9203125 x 10-19 Joules.
Question 3
Calculate the energy of a gamma-ray photon whose frequency is 5.02 x 1020 Hz
Data: You will need to first extract the important values from the question
The frequency, f = 5.02 x 1020 Hz
Planck’s constant, h = 6.63 x 10-34 Js
Unknown value to find:
The energy of the photon, E =?
Formula:
E = hf
Solution:
E = hf = 6.63 x 10-34 x 5.02 x 1020 = 3.32826 x 10-13 J
Therefore, we have obtained
E = 3.32826 x 10-13 J
Therefore, the energy of a gamma-ray photon whose frequency is 5.02 x 1020 Hz is 3.33 x 10-13 Joules.
Question 4
Calculate the Energy of a Photon of Radiation With a Frequency of 8.5×10^14 Hz
Data: Here is the available data from the question above:
The frequency, f = 8.5 x 1014 Hz
Planck’s constant, h = 6.63 x 10-34 Js
Unknown value to find:
The energy of the photon of radiation, E =?
Formula:
The formula for calculating the photon of radiation, E = hf
Solution:
E = hf = 6.63 x 10-34 x 8.5 x 1014 = 5.6355 x 10-19 J
Hence, our final answer for the energy of the photon of radiation is
E = 5.6355 x 10-19 J
Therefore, the energy of a photon of radiation is 5.6355 x 10-19 Joules.
You can also calculate the energy of a photon of each frequency. by applying the energy of a photon calculator.
You may also like to read:
How to Calculate the Work Function of a Metal
Solved Problem: How to Calculate the Wavelength of Radiation
How to Calculate the Kinetic Energy of an Ejected Electron
Also How to Calculate the Threshold Frequency of Quantum Energy