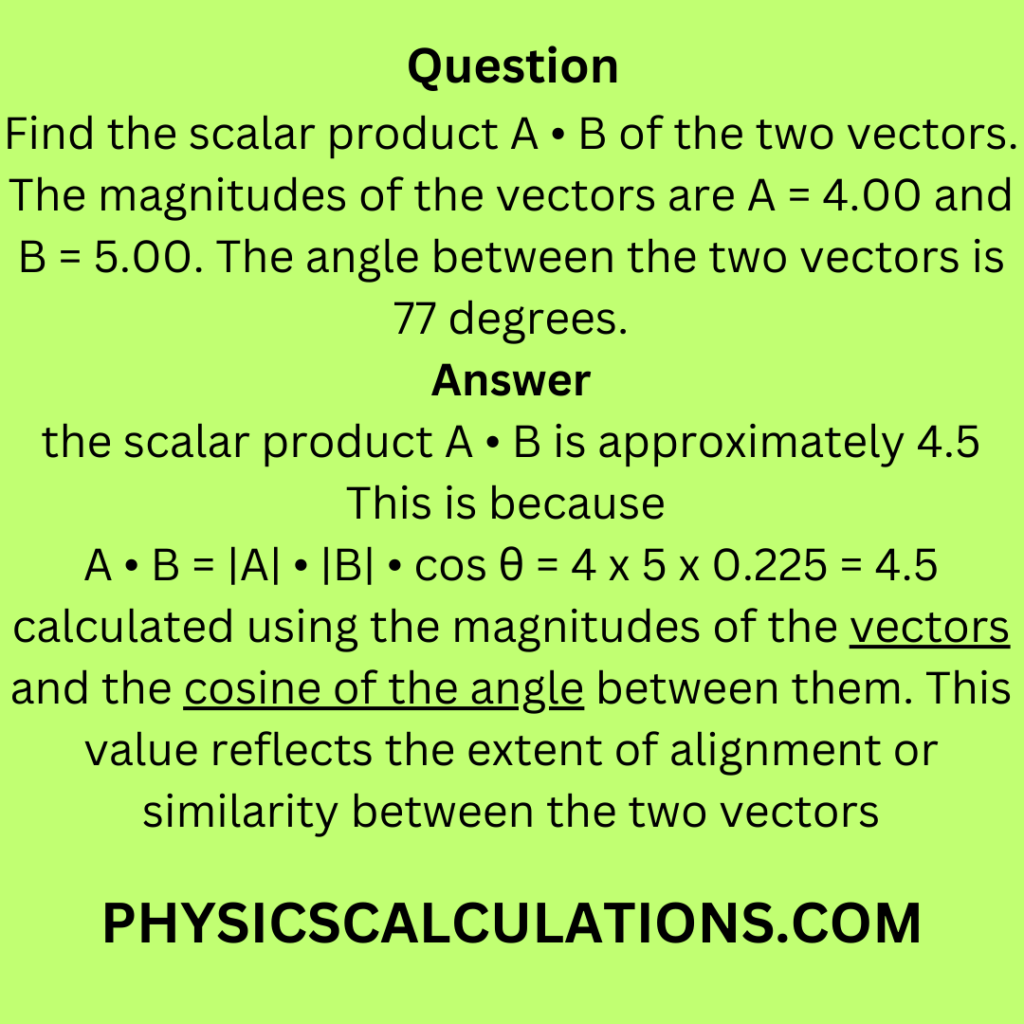
Question
Find the scalar product A • B of the two vectors. The magnitudes of the vectors are A = 4.00 and B = 5.00. The angle between the two vectors is 77 degrees.
Answer
In summary, the scalar product A • B is approximately 4.5 (because A • B = |A| • |B| • cos θ = 4 x 5 x 0.225 = 4.5), calculated using the magnitudes of the vectors and the cosine of the angle between them. This value reflects the extent of alignment or similarity between the two vectors.
However, below is a comprehensive explanation and a step-by-step guide to the problem:
Our task is to find the scalar product A • B of two vectors, A and B, given their magnitudes and the angle between them.
You may also like to read:
Given the two displacements D = (6i + 3j – k) m and E = (4i – 5j + 8k) m
Data:
We are provided with the following information:
- The magnitude of vector A, denoted as |A|, is 4.00.
- Magnitude of vector B, denoted as |B|, is 5.00.
- The angle between the two vectors is 77 degrees.
Unknown:
We need to find the scalar product A • B = ?
Formula:
To solve this problem, we’ll use the formula for the scalar (dot) product of two vectors (A) and (B):
A • B = |A| • |B| • cos θ
Where:
- |A| and |B| are the magnitudes of vectors A and B.
- θ is the angle between the two vectors.
Solution:
First Step:
Let’s start by identifying the given data:
- Magnitude of vector A, |A| = 4.00.
- The magnitude of vector B, |B| = 5.00.
- Angle between the two vectors θ = 77 degrees.
Second Step:
Now, we can apply the formula for the scalar product:
A • B = |A| • |B| • cos θ
Plugging in the values:
A • B = 4 x 5 • cos 770
Third Step:
Calculate the cosine of 77 degrees. You can use a calculator for this:
cos 770 = 0.225
Fourth Step:
Now, let’s substitute this value back into the equation:
A • B = 4 x 5 x 0.225
Fifth Step:
Calculate the result:
A • B = 4 x 5 x 0.225 = 4.5
Explanation:
The scalar product A • B of the two vectors A and B is approximately 4.5.
In this problem, we used the formula for the scalar product of vectors and applied it to the given magnitudes |A| and |B| and the angle θ between them. The scalar product represents the product of the magnitudes of the vectors and the cosine of the angle between them.
This concept is very important in physics and engineering, as it allows us to determine the extent to which two vectors are aligned. A scalar product of zero means the vectors are orthogonal (perpendicular), while a positive scalar product indicates they are oriented in the same general direction.
In this particular case, the scalar product of 4.5 suggests that there is some degree of alignment between vectors A and B, given the angle of 77 degrees.